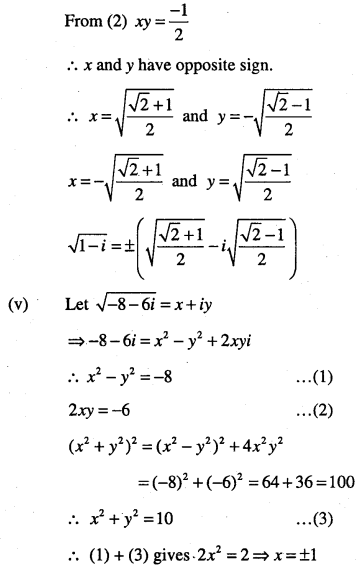1st PUC Maths Question Bank Chapter 5 Complex Numbers and Quadratic Equations

Students can Download Maths Chapter 5 Complex Numbers and Quadratic Equations Questions and Answers, Notes Pdf, 1st PUC Maths Question Bank with Answers helps you to revise the complete Karnataka State Board Syllabus and score more marks in your examinations.

Karnataka 1st PUC Maths Question Bank Chapter 5 Complex Numbers and Quadratic Equations

Question 1.
Define a complex number.
Answer :
A number of the form a + ib, where a and b are real numbers, is defined to be a complex number, where i² = -1.
Here ‘a’ is called the real part, denoted by Re (z) and ‘b’ is called the imaginary part, denoted by Im (z), of the complex number z = a + ib.

Question 2.
When you say that two complex numbers are equal?
Answer :
Two complex numbers z₁ = a + ib and z₂ = c + id are equal if a = c and b – d.

Question 3.
Define purely real and purely imaginary numbers.
Answer :
A complex number z is said to be:

• Purely real, if Im(z) = 0,
• Purely imaginary, if Re(z) = 0

Question 4.
If 4x + i(3x – y) = 3 +i (-6), where x and y are real numbers, then find the values of
x and y.
Answer :
Given 4x + i(3x – y) = 3 + i(-6), Equating the real and the imaginary parts,we get
4x = 3 and 3x – y = -6
\(\Rightarrow x=\frac{3}{4} \text { and } y=3\left(\frac{3}{4}\right)+6=\frac{33}{4}\)

Question 5.
Define addition of two complex numbers.
Answer :
Let z₁=a + ib and z₂=c + id be any two complex numbers. Then addition of z₁ and z₂ defined as z₁ + z₂ = (a + c) + i(b + d) which is again a complex number.
Properties of addition of complex numbers:

(i) Closure property: The sum of two complex numbers is always a complex number, i.e., addition is closed in C set of complex numbers.

(ii) Commutative property: For any two complex numbers z₁ and z₂
we have z₁ + z₂ = z₂ + z₁

(iii) Associative properly: For any complex numbers z₁,z₂ and Z₃, we have
\( z_{1}+\left(z_{2}+z_{3}\right)=\left(z_{1}+z_{2}\right)+z_{3}\)

(iv) Existence of additive identity: For any complex number z, we have z + 0 = 0 +-z = z
Thus, 0 is the additive identity for complex numbers.

(v) Existence of additive inverse: Every complex number z-a + ib has -z = (-a) + i(-b) as its additive inverse, as z + (-z) = (-z) + z = 0.

Question 6.
Define difference of two complex numbers.
Answer :
Let z₁ and z₂ be any two complex numbers. The different z₁ – z₂ is defined as follows:
z_{1 –} z₂ =z₁ +(-z₂)

Question 7.
Define multiplication numbers.
Answer :
Let z₁=a + ib and z₂ = c + id be any two complex numbers. Then, the product z₁z₂ is defined as follows:
z₁z₂ = (ac – bd) + i(ad + bc)

Properties of product of complex numbers:

• Closure property: The product of two complex numbers is always a complex number.
• Commutative law: For any two complex numbers z₁ and z₂ we have z₁ z₂ = z₂z₁
• Associative law: For any three complex numbers z₁ z₂ and z₃ we have (z₁z₂)z₃=z₁(z₂z₃).
• Existence of multiplicative identity: For every complex number z, we have z . 1 = 1. z = z. Thus, 1 is the multiplicative identity.
• Existence of multiplicative inverse: Let z = a + ib. Then
  \(z^{-1}=\frac{1}{z}=\frac{1}{a+i b}=\frac{1}{a+i b} \cdot \frac{a-i b}{a-i b}=\frac{a-i b}{a^{2}+b^{2}} \)
  Clearly
  \(z \cdot \frac{1}{z}=\frac{1}{z} \cdot z=1\)
  Thus, every z = a + ib has its multiplicative inverse, given
  \( z^{-1}=\frac{1}{z}=\frac{a}{a^{2}+b^{2}}-\frac{a}{a^{2}+b^{2}} i \quad(z \neq 0)\)

Question 8.
Define division of two complex numbers.
Answer :
Let z₁ and z₂ be two complex numbers and z₂ ≠0.Then \( \frac{z_{1}}{z_{2}} \) is defined by  \( \frac{z_{1}}{z_{2}}=z_{1}\left(\frac{1}{z_{2}}\right) \)

Question 9.
Prove the following
(i) \(\left(z_{1}+z_{2}\right)^{2}=z_{1}^{2}+2 z_{1} z_{2}+z_{2}^{2}\)
(ii) \(\left(z_{1}-z_{2}\right)^{2}=z_{1}^{2}-2 z_{1} z_{2}+z_{2}^{2}\)
(iii) \(\left(z_{1}+z_{2}\right)^{3}=z_{1}^{3}+3 z_{1}^{2} z_{2}+3 z_{1} z_{2}^{2}+z_{2}^{3}\)
(iv) \(\left(z_{1}-z_{2}\right)^{3}=z_{1}^{3}-3 z_{1}^{2} z_{2}+3 z_{1} z_{2}^{2}-z_{2}^{3}\)
(v) \(z_{1}^{2}-z_{2}^{2}=\left(z_{1}+z_{2}\right)\left(z_{1}-z_{2}\right) \)
Answer:
(i) We have, (z₁ + z₂)² = (z, + z₂)(z₁ + z₂)
= (z₁ + z₂)z₁ + (z₁ + z₂)z₂ (by distributive law)
= z²₁ + z₂z₁ +z₁z₂ + z₂ (by distributive law)
\( =z_{1}^{2}+2 z_{1} z_{2}+z_{2}^{2} \quad \ z_{1} z_{2}=z_{2} z_{1}\)
Remaining try yourself.

Question 10.
Express the following in the form of a+ib
(i) \( (-5 i)\left(\frac{1}{8} i\right)\)
(ii) \((5 i)\left(-\frac{3}{8} i\right)\)
(iii) \((2+i 3)+(-6+i 5)\)
(iv) \((6+3 i)-(2-i)\)
(v) \((2-i)-(6+3 i)\)
(vi) \((3+5 i)(2+6 i)\)
(vii) \(3(7+7 i)+i(7+7 i)\)
(viii) \((1-i)-(-1+6 i)\)
(ix)\(\left(\frac{1}{5}+\frac{2}{5} i\right)-\left(4+\frac{5}{2} i\right)\)
(x)\(\left[\left(\frac{1}{3}+i \frac{7}{3}\right)+\left(4+i \frac{1}{3}\right)\right]-\left(-\frac{4}{3}+i\right) \)
(xi) \(i^{9}+i^{19}\)
(xii)\( i^{-39}\)
(xiii)\((-i)(2 i)\left(-\frac{1}{8} i\right)^{3}\)
(xiv) \((5-3 i)^{3}\)
(xv) \(\left(\frac{1}{3}+3 i\right)^{3}\)
(xvi) \(\left(-2-\frac{1}{3} i\right)^{3}\)
(xvii) \((\mathbf{1}-i)^{4}\)
(xviii) \(\frac{5+\sqrt{2} i}{1-\sqrt{2 i}}\)
(xix) \( \frac{(3+i \sqrt{5})(3-i \sqrt{5})}{(\sqrt{3}+\sqrt{2} i)-(\sqrt{3}-\sqrt{2} i)}\)
Answer:

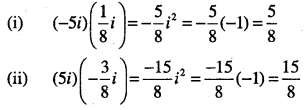
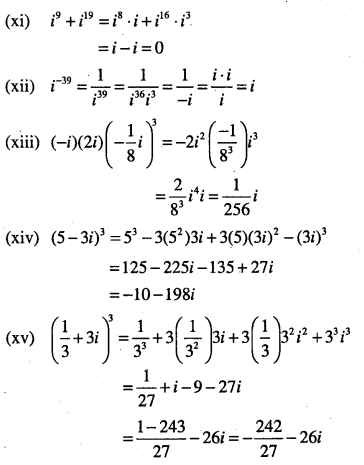
Question 11.
Define modulus of a complex number.
Answer :
Let. z = a + ib be a complex number. Then, the modulus of z, denoted by |z| to be the non-negative real number \(\sqrt{a^{2}+b^{2}}\)

Question 12.
Define conjugate of a complex number.
Answer :
Let z = a + ib be a complex number. Then, the conjugate of complex number z, denoted \(\bar{z} \) as , is the complex number a – ib, i.e., i= a – ib

Question 13.
Write down the modulus of
(i) i
(ii) \( 3+\sqrt{-3}\)
(iii)\((-1-i)^{3}\)
Answer:

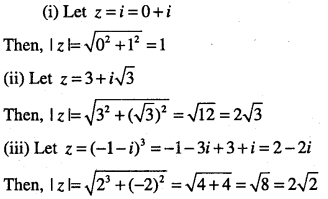
Question 14.
Write down the conjugate of
(i) \((2+\sqrt{-2})\)
(ii) \( i^{3}\)
(iii) \((3-4 i)^{2} \)
Answer:

Question 15.
Find the multiplicative inverse of the following complex numbers:
(i) 2-3i
(ii) 4-3i
(iii) \(\sqrt{5}+3i\)
(iv) \(\sqrt{5}+3i \)
(v) \(-i\)
Answer:

Question 16.
Find the conjugate of  \(\frac{(3-2 i)(2+3 i)}{(1+2 i)(2-i)}\)
Answer:

Question 17.
Evaluate:
\( \left[i^{18}+\left(\frac{1}{i}\right)^{25}\right]^{3}\)
Answer:

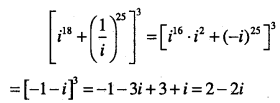
Question 18.
Reduce \( \left(\frac{1}{1-4 i}-\frac{2}{1+i}\right)\left(\frac{3-4 i}{5+i}\right)\) to the standard form.
Answer:

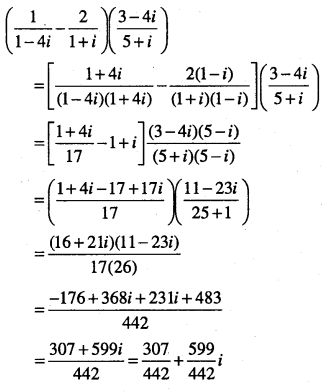
Question 19.
Find the modulus of
\(\frac{1+i}{1-i}-\frac{1-i}{1+i}\)
Answer:

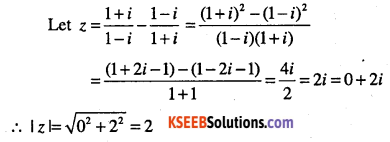
Question 20.
Find the real numbers x and y if (x – iy) (3 + 5i) is the conjugate of -6 – 24i
Answer:

Question 21.
For any two complex numbers Z₁ and Z₂, prove that
Re(z₁z₂) = Re(z₂) . Re(z₂) – Im(z₁) . Im(z₂)
Answer :
z₁=a + ib and z₂=c + id
z₁z₂ = {a + ib)(c + id) = (ac-bd) + i(ad + bc)
∴ Re(z₁z₂) = ac-bd
= Re(z₁).Re(z₂)-1m(z₁)\m{z₂)

Question 22.
\(\text { If } z_{1}=2-i, z_{2}=1+i, \text { find }\left|\frac{z_{1}+z_{2}+1}{z_{1}-z_{2}+1}\right| \)
Answer:

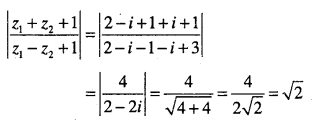
Question 23.
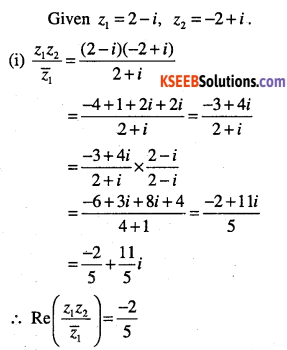
Let z₁ = 2 – i, z₂ = -2 + i .Find

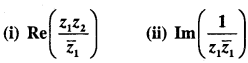
Answer:

Question 24.
If \(x+i y=\frac{a+i b}{a-i b}, \text { prove that } x^{2}+y^{2}=1\)
Answer:

Question 25.
If \(x-i y=\sqrt{\frac{a-i b}{c-i d}}\) Prove that
\(\left(x^{2}+y^{2}\right)^{2}=\frac{a^{2}+b^{2}}{c^{2}+d^{2}}\)
Answer:

Question 26.
If \(a+i b=\frac{(x+i)^{2}}{2 x^{2}+1}\), Prove that \(a^{2}+b^{2}=\frac{\left(x^{2}+1\right)^{2}}{\left(2 x^{2}+1\right)^{2}}\)
Answer:

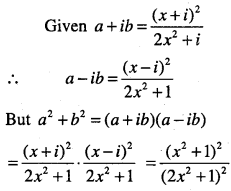
Question 27.
If (x + iy)³ =u + iv, then show that
\(\frac{u}{x}+\frac{v}{y}=4\left(x^{2}-y^{2}\right)\)
Answer:

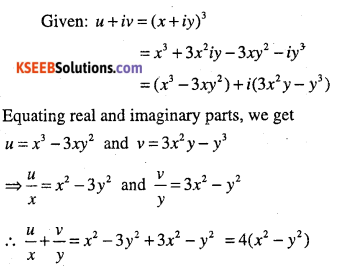
Question 28.
If (a + ib)(c + id){e + if) (g + ih) = A + iB then show that (a²+b²)(c²+d²)(e²+f²) (g²+h²) = A²+B²
Answer:
Given: A + iB = (a + ib)(c + id)(e + if)(g + ih)
∴  A-iB = (a- ib)(c – id)(e – if)(g – ih)
But A² + B² = (A + iB)(A – iB)
= (a² +b²)(c²+d²)(c²+f²)(g²+h²)

Question 29.
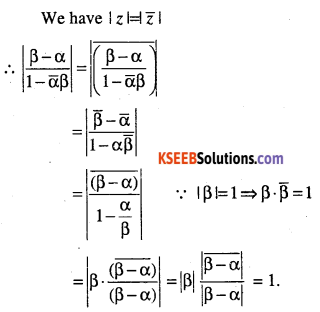
If α and β are different complex numbers with |β|=1 then find
\(\left|\frac{\beta-\alpha}{1-\bar{\alpha} \beta}\right|\)
Answer:

Question 30.
If \(\left(\frac{1+i}{1-i}\right)^{m}=1 \)then find the least positive integral value of m.
Answer:

Question 31.
Find the number of non-zero integral solutions of the equation \(|1-i|^{x}=2^{x}\)
Answer:

Question 32.
Find real θ such that \(\frac{3+2 i \sin \theta}{1-2 i \sin \theta}\) is purely real
Answer:

Question 33.
Define the polar form of complex number.
Answer:

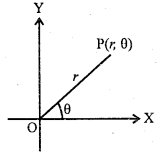
Let the complex number z = x + iy be represented by the point P(x,y) in the complex plane.
Let \(| X O P=\theta \text { and }|O P|=r>0\)
Then
P(r, θ) are called the polar coordinates of P.
where x = rcosθ
y = rsinθ
∴ z = r(cosθ+ i sin θ)
This is called the polar form or trigonometric form or modulus – amplitude form of z.

Keen Eye;

• \( r=\sqrt{x^{2}+y^{2}}=1 z 1 \) is called the modulus of z and 0 is called the argument (or amplitude) of z, written as arg (z) or amp (z).
• The value of such that -π<θ≤n is called principal argument of z.
• To find θ

Case (i):
When z is purely real. Then, it lies on the x-axis.
(i) If x > 0, then θ=0
(ii) If x < 0, then θ = π

Case (ii):
When z is purely imaginary. Then, it lies on the y-axis
(i) \(\text { If } y>0, \text { then } \theta=\frac{\pi}{2}\)
(ii) \(\text { If } y<0, \text { then } \theta=-\frac{\pi}{2}\)

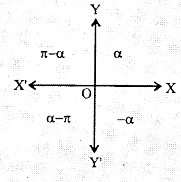
Case(iii):
Let tan α =|tan θ|
\(\text { where } 0<\alpha<\frac{\pi}{2}\)

(i) θ = α, when z lies in I quadrant
(ii) θ – π – α, when z lies in II quadrant
(iii) θ = α – π, when z lies in III quadrant
(iv) θ = -α, when z lies in IV quadrant

Question 34.
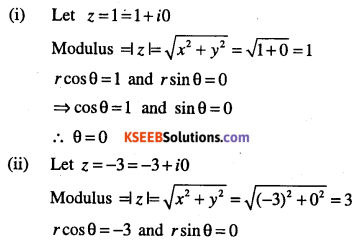
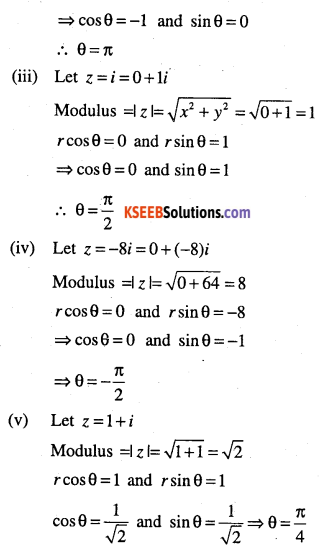
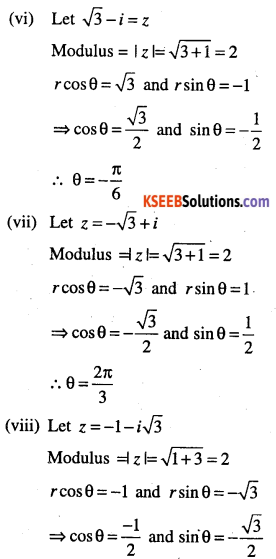
Find the modulus and the arguments of the following:
(i) 1
(ii) -3
(iii) i
(iv) -8i
(v) 1+i
(vi) \(\sqrt{3}-i\)
(vii)\(-\sqrt{3}+i\)
(viii)\(-1-i \sqrt{3}\)
(ix)\(\frac{1+2 i}{1-3 i}\)
(x)\(\frac{1+i}{1-i}\)
(xi) \(\frac{1}{1+i}\)
Answer:

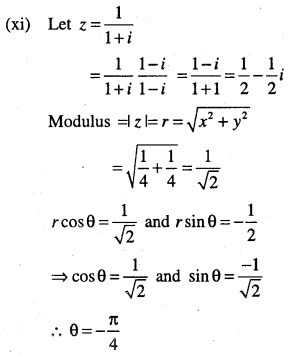
Question 35.
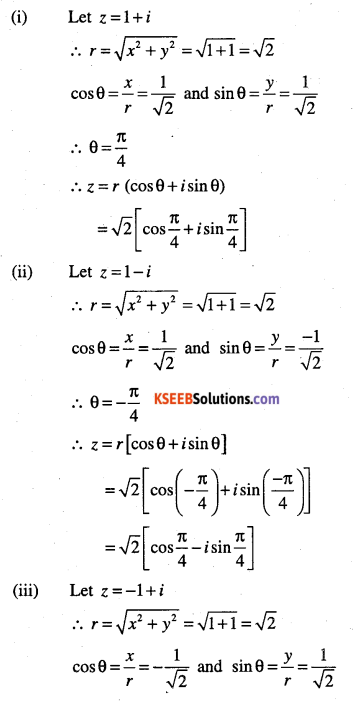
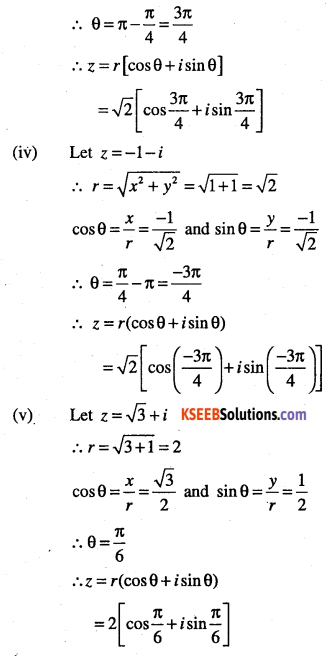
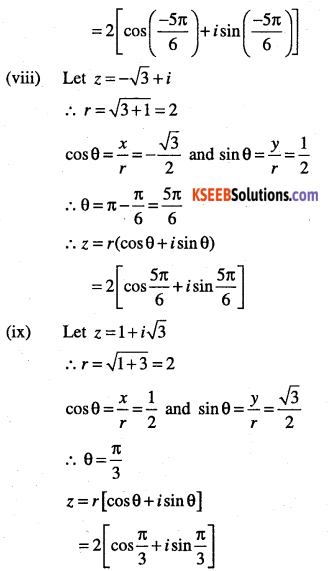
Convert the Following complex numbers in the polar form:
(i) 1+i
(ii) 1-i
(iii) -1+i
(iv) -1-i
(v) \(\sqrt{3}+i\)
(vi) \(\sqrt{3}-i\)
(vii) \(-\sqrt{3}-i\)
(viii) \(-\sqrt{3}+i\)
(ix) \(1+i \sqrt{3}\)
(x) \(1-i \sqrt{3}\)
(xi) \(-1+i \sqrt{3}\)
(xii) \(-1-i \sqrt{3}\)
(xiii) -3
(xiv) i
(xv) \(\frac{-16}{1+i \sqrt{3}}\)
(xvi) \(\frac{1+7 i}{(2-i)^{2}}\)
(xvii) \(\frac{1+3 i}{1-2 i}\)
(xviii)
\(\frac{i-1}{\cos \frac{\pi}{3}+i \sin \frac{\pi}{3}}\)
Answer:

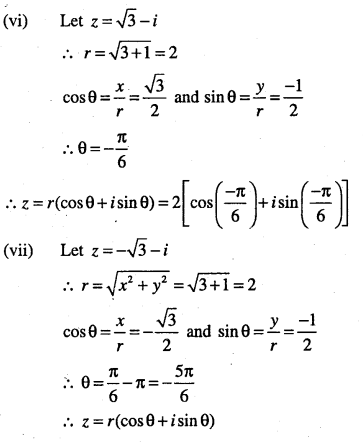
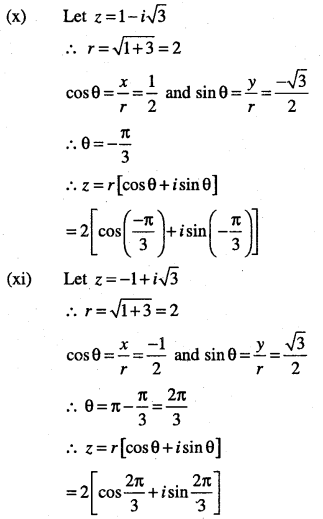
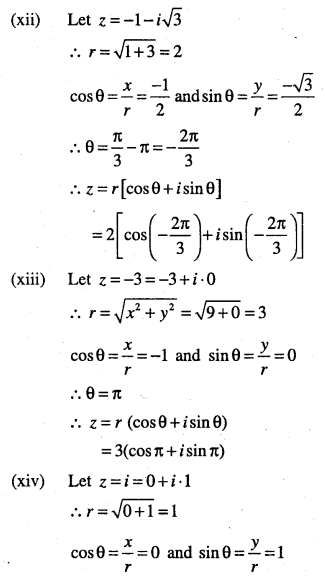
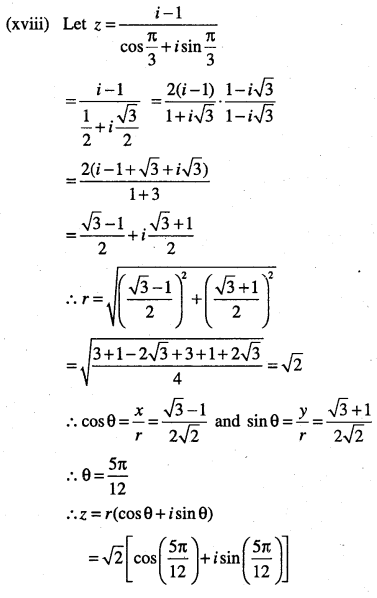
Question 36.
Keen Eye:

• A polynomial equation has at least one root.
• A polynomial equation of degree V has n roots. Solve each of the following equations:

(i) x² + 2 = 0
(ii) x² + 3 = 0
(iii) x² + x +1 = 0
(iv) 2x² + x +1 = 0
(v) x² + 3x + 9 = 0
(vi) -x² + x – 2 = 0
(vii) x² + 3x + 5 = 0
(viii) x² – x + 2 = 0
(ix)\(3 x^{2}-4 x+\frac{20}{3}=0\)
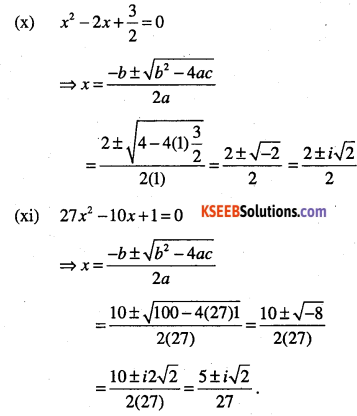
(x) \(x^{2}-2 x+\frac{3}{2}=0\)
(xi) 27x² -10x + 1 = 0
(xii) \(\sqrt{2} x^{2}+x+\sqrt{2}=0\)
(xiii) \(\sqrt{3} x^{2}-\sqrt{2} x+3 \sqrt{3}=0\)
(xiv)\( x^{2}+x+\frac{1}{\sqrt{2}}=0\)
(xv) \(x^{2}+\frac{x}{\sqrt{2}}+1=0\)
Answer:



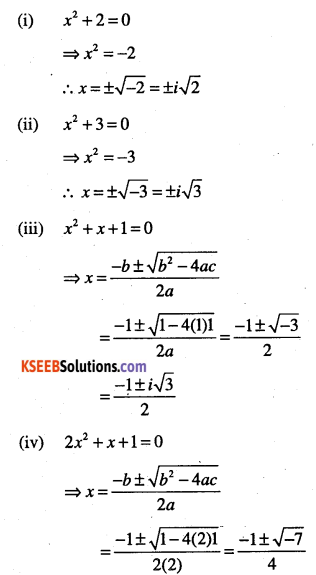
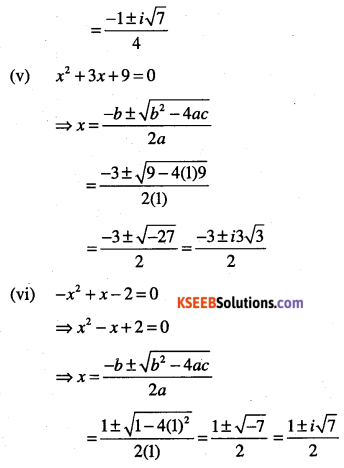
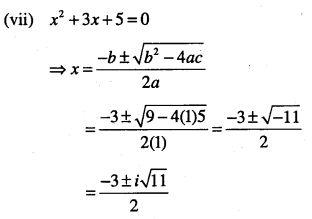
(viii) x² -x+2=0

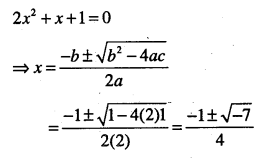
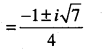
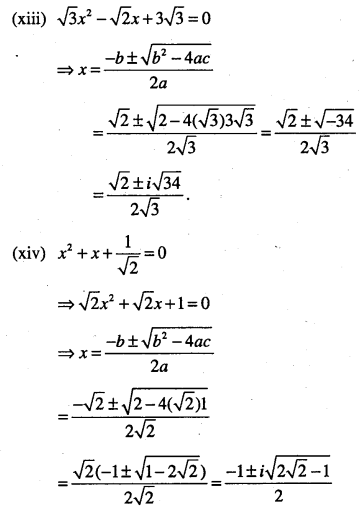
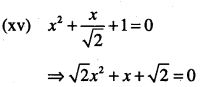
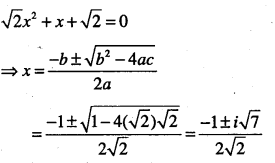
Question 37.
Find the equation roots of the following:
(i) i
(ii) i
(iii) 1+i
(iv) 1-i
(v) -8-6i
(vi)-15-8i
(vii) -7-24i
Answer:
(i)

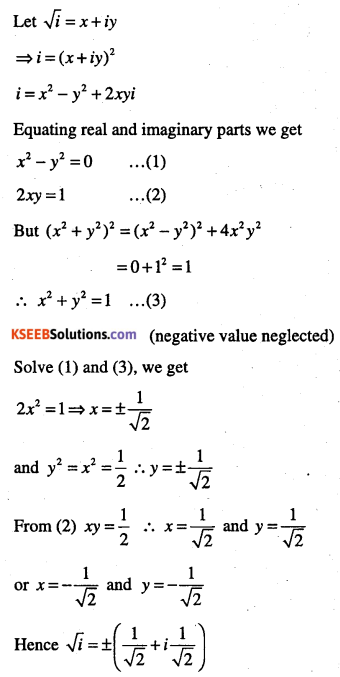
(ii)


(iii)

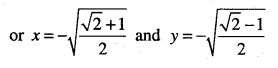
(iv)