KSEEB SSLC Class 10 Maths Solutions Chapter 9 Polynomials Ex 9.2 are part of KSEEB SSLC Class 10 Maths Solutions. Here we have given Karnataka SSLC Class 10 Maths Solutions Chapter 9 Polynomials Exercise 9.1.
Karnataka SSLC Class 10 Maths Solutions Chapter 9 Polynomials Exercise 9.2
Question 1.
Find the zeroes of the following quadratic polynomials and verify the relationship between the zeroes and their coefficients
(i) x2 – 2x – 8
(ii) 4s2 – 4s + 1
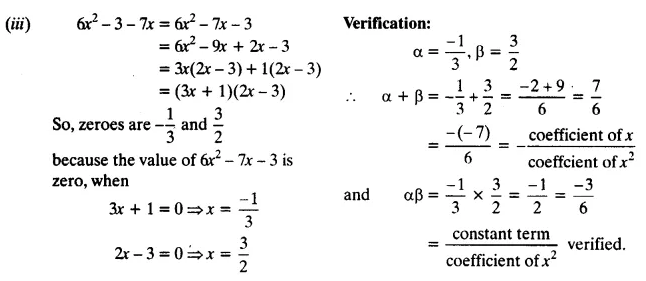
(iii) 6x2 – 3 – 7x
(iv) 4u2 – 8u
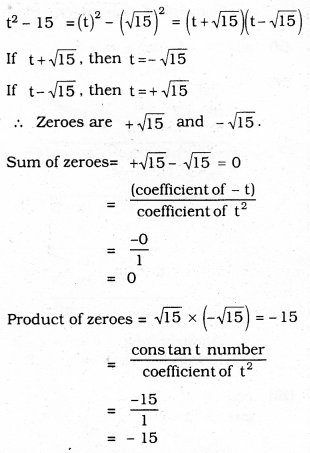
(v) t2 – 15
(vi) 3x2 – x – 4
Solution:


(iv) 4u2 – 8u
= 4u2 – 8u + 0
= 4u (u – 2)
If 4u = 0, then u = 0
If u – 2 = 0, then u = 2
∴ Zeroes are 0 and 2

![]()
(v) t2 – 15
= t2 + 0 – 15
(vi) 3x2 – x – 4
= 3x2 – 4x + 3x – 4
= x(3x – 4) + 1 (3x – 4)
= (3x – 4) (x + 1)
If 3x – 4 = 0, then x = \(\frac{4}{3}\)
If x + 1 = 0, then x = -1
∴ Zeroes are \(\frac{4}{3}\) and -1.

![]()
Question 2.
Find a quadratic polynomial each with the given numbers as the sum and product of its zeroes respectively.
(i) \(\frac{1}{4}\), 1
(ii) \(\sqrt{2}, \frac{1}{3}\)
(iii) 0, \(\sqrt{5}\)
(iv) 1, 1
(v) \(-\frac{1}{4}, \frac{1}{4}\)
(vi) 4, 1
Solution:
(i) \(\frac{1}{4}\), 1
Here m + n = \(\frac{1}{4}\), mn = -1
∴ Quadratic Equation
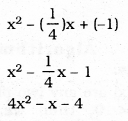
![]()
(ii) \(\sqrt{2}, \frac{1}{3}\)
Here m + n = \(\sqrt{2}\), mn = \(\frac{1}{3}\)
∴ Quadratic Equation
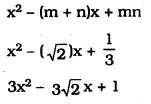
(iii) 0, \(\sqrt{5}\)
Here m + n = 0, mn = \(\sqrt{5}\)
∴ Quadratic Equation
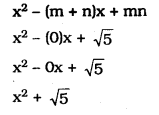
(iv) Standard form of quadratic polynomial
sum and product of its zeroes is
K(x2 – sum of the zeroes) x + product of zeroes.
= K(x2 – 1x + 1)
Taking K = 1
= x2 – x + 1
(v) \(-\frac{1}{4}, \frac{1}{4}\)
Here m + n = \(-\frac{1}{4}\), mn = \(\frac{1}{4}\)
∴ Quadratic Equation
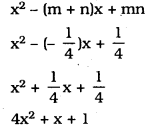
(vi) Standard form of quadratic polynomial sum and product of its zeroes is
= K[x2 – (sum of the zeroes) x + Product of zeroes]
= K(x2 – 4x + 1)
Taking K = 1
= 1(x2 – 4x + 1)
= x2 – 4x + 1
We hope the given KSEEB SSLC Class 10 Maths Solutions Chapter 9 Polynomials Ex 9.2 will help you. If you have any query regarding Karnataka SSLC Class 10 Maths Solutions Chapter 9 Polynomials Exercise 9.2, drop a comment below and we will get back to you at the earliest.