2nd PUC – KSEEB Solutions

2nd PUC Sociology Question Bank Chapter 3 Inclusive Strategies

November 2, 2023

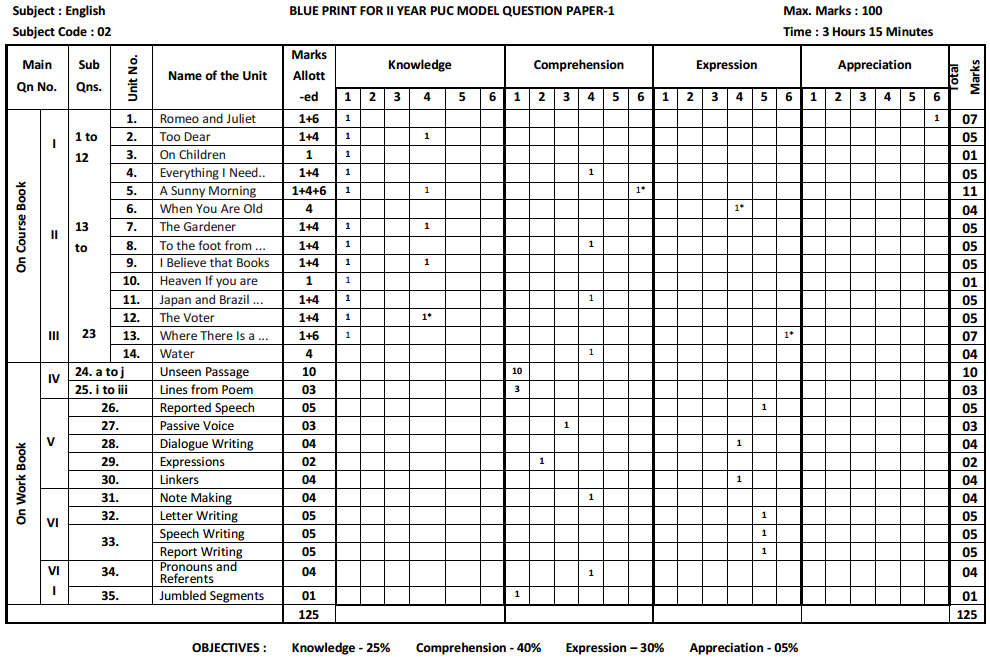
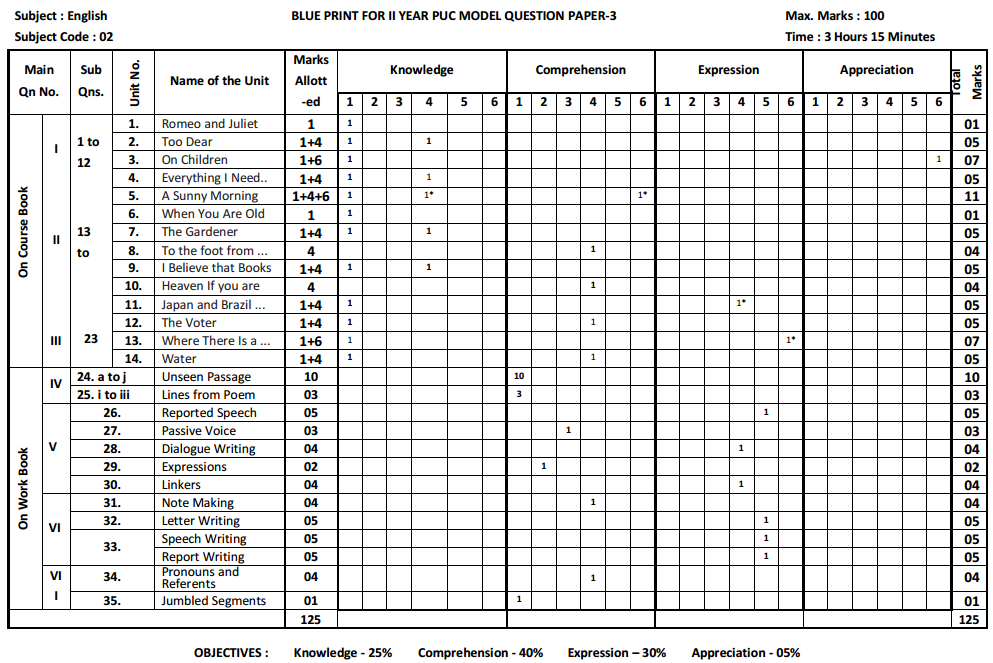
Karnataka 2nd PUC Sociology Question Bank Chapter 3 Inclusive Strategies

You can Download Chapter 3 Inclusive Strategies Questions and Answers, Notes, 2nd PUC Sociology Question Bank with Answers Karnataka State Board Solutions help you to revise complete Syllabus and score more marks in your examinations.

2nd PUC Sociology Inclusive Strategies One Mark Questions and Answers

Question 1.
Which Article of the Indian Constitution abolishes untouchability?
Answer:
Article 17.

Question 2.
In which year the untouchability (Offences) Act was enacted?
Answer:
1955.

Question 3.
In which year the Programe Stree Shakhti was launched?
Answer:
2000-2001.

Question 4.
Who introduced the concept of Micro Finance in Bangladesh?
Answer:
Muhammad Yunus.

Question 5.
Where is the Headquarters of Lijjat located?
Answer:
Mumbai.

Question 6.
Which non-governmental organization is working for Soligas?
Answer:
Vivekananda, Girijana Kendra.

Question 7.
Who started Harijana and young India Newspapers?
Answer:
Mahathama Gandhi.

Question 8.
Who started Mookanayak News Papers?
Answer:
Dr. B.R. Ambedkar.

Question 9.
Who founded Sulabh International?
Answer:
Dr. Bindeshwar Pathak.

Question 10.
What is gender discrimination?
Answer:
Discrimination against people based on their Gender.

Question 11.
In which year towards equality report was Submitted?
Answer:
1974.

Question 12.
Which year Government of India declared as year of women empowerment?
Answer:
2001.

Question 13.
Who coined affirmative Action?
Answer:
John. F. Kennedy.

Question 14.
Who started Harijana Sevaka Sangha?
Answer:
Mahathama Gandhi.

Question 15.
Who started Bahishkritha Hitha Karinisabha?
Answer:
Dr. B.R. Ambedkar.

Question 16.
Expand LAMPS.
Answer:
Large Area Multi Purpose Societies.

Question 17.
Expand TRIFED.
Answer:
Tribal Co-Operative Market Development Federation of India.

Question 18.
In which year protection of civil Rights Act was passed?
Answer:
1976. .

Question 19.
Expand NCW.
Answer:
National Commission for Women.

Question 20.
Expand SGH.
Answer:
Self – Help Groups.

Question 21.
Expand SEWA.
Answer:
SelfEmployed Women Association.

Question 22.
Expand SKDRDP.
Answer:
Shri Kshethra Dharmasthala Rural Development Project.

Question 23.
Expand SCP.
Answer:
Special Component Plan.

Question 24.
Expand SCA.
Answer:
Special Central Assistance.

Question 25.
Expand SCDC.
Answer:
Scheduled Caste Development Corporation.

Question 26.
Expand CIIL.
Answer:
Central Institute of Indian languages.

Question 27.
What is the provision of Article 335 of the constitution?
Answer:
Reservations to SCs and STs.

Question 28.
In which year national commission for women was established.
Answer:
1992.

Question 29.
Expand NABARD.
Answer:
National Bank for Agriculture and Rural Development.

2nd PUC Sociology Inclusive Strategies Two Marks Questions and Answers

Question 1.
Give the meaning of Affirmative action.
Answer:
Affirmative action refers to policies that take factors including “caste, creed, religion, or sex, into consideration, in order to benefit an underrepresented group in areas of employment, education, and business”.

Question 2.
Explain the concept of Women Empowerment.
Answer:
The dictionary meaning of the terms “empowerment” is to give power or authority. Hence empowerment is the act of giving power. Thus women’s empowerment is the act of empowering women i .e. to give them the power or authority. The term Empowerment has different meanings to women of different classes.

Question 3.
What is a self help group?
Answer:
“A Self-Help Group comprises a group of micro enterpreneurs having homogenous social and economic backgrounds, all voluntarily coming together to save regular small sums of money, mutually agreeing to contribute to a common fund and to meet their emergency needs on the basis of mutual help”.

Question 4.
What is micro finance?
Answer:
Micro Finance is defined as, financial services such as Saving A/c, Insurance Fund & credit provided to poor & low income clients so as to help them to rise their income & there by improve their standard of I living.

Question 5.
What is Stree Shakthi?
Answer:
The programme was launched during 2000-01 and it is being implemented throughout the state to empower rural women and make them self reliant is called as Stree Shakthi.

Question 6.
Mention any two objectives of stree shakhti.
Answer:

To strengthen the process of economic development of rural women and create a conducive environment for social change.
To form self help group based on thrift and credit principles which builds self reliance and enable women to have greater access and control over resources.

Question 7.
Mention the advantages of Internal lending in self help groups.
Answer:
Managing their own common fund, financing needs of each other develops their skills of financial management.

2nd PUC Sociology Inclusive Strategies Five Marks Questions and Answers

Question 1.
What were the provisions of the Untouchability Act 1955?
Answer:
The Untouchability (Offences) Act -1955. It provides penalties for the following offences.

Preventing a person on grounds of untouchability, from entering a place of public worship, offering prayer therein or taking water from a tank, well or spring.
Enforcing all kinds of social disabilities such as denying access to any shop, public
restaurant, public hospital or educational institutions, hotel or any other place of public entertainment, the use of any road, river, well, tank, water tap, cremation ground, sanitary convenience and Dharmashalas.’
Enforcing occupational, professional or trade disabilities in the matter or enjoyment of any benefit under the charitable trust in the construction or occupation of any residential premises in any locality or the observance of any social or religious usages or ceremony.
Refusing to sell goods or render services to an untouchable.

Question 2.
Briefly explain the role of NGOs for upliftment of SCs/STs.
Answer:
Non-Governmental. Organizations have played a vital role in welfare of Scheduled Castes. The important ones are (1) Harijan Sevak Sangh, (2) Indian Depressed Classes League, (3) Hindi Sweepers Sevak Sangh, (4) Servants India Society, (5) Ishwar Saran Ashram, (6) All India Backward Classes Federation, (7) Bharatiya Adimajati Sevak Sangh, Vanarasikalayana Sabha, Sri Ramakrishna Mission etc., have also made efforts to eradicate untouchability.

Question 3.
List out the strategies for women empowerment.
Answer:
The strategies for empowerment of women can be classified as legal, social and economic.
(1) Legal Strategies: After the independence several laws were drafted with the aim to treat women on par with men. Some of the legislation are as follows :

Hindu Marriage Act of 1955
Hindu Succession Act of 1956.
Hindu Adoption and Maintenance Act of 1956.
Dowry Prohibition (Amendment) Act 1984
Domestic Violence Act 2005 etc.

(2) Social Strategies: Social strategies are as follow:

Establishment of Women Welfare Services.
Legal literacy of women through mass media.
Help of neighbours to be sought in the cases of abused women.
Conducting public education and awareness programmes in order to help women.
Males are also to be educated to realize their new roles in the changed times and the necessity of their own contribution to family life.

(3) Economic Strategies: Economic strategies are as follow;

Educational and vocational training for women which enable them to seek jobs and become economically dependent.
Technological aids that will be labour saving devices and will lighten women’s burden of heavy daily tasks.
Train women in both formal and non-formal education.
Credit facilities to start small-scale industr.evself-employment.
Programmes of placing women in important positions at various levels.

Question 4.
Explain the main features of micro finance.
Answer:
Micro Finance is defined as, financial services such as Savings Accounts, Insurance Fund & credit provided to poor & low income clients so as to help them to rise their income & there by improve their standard of living. Microfinance is a source of financial services for entrepreneurs and small businesses lacking access to banking and related services.
Major Features of Microfinance:

Loan without security
Loans to people who live BPL (Below Poverty Line)
Even members of SHG may get benefit from Micro Finance
Maximum limit of loan under microfinance is relatively small amount.
The terms and conditions given to poor people are decided by SHG.

For some, microfinance is a movement whose object is a world in which as many poor to have permanent access to an appropriate range of high quality financial services, including not just credit but also savings, insurance, and fund transfers. Many of those who promote microfinance generally believe that such access will help poor people out of poverty. For others, microfinance is a way to promote economic development, employment and growth through the support of micro-entrepreneurs and small businesses.

Question 5.
Explain the objectives of towards equality report.
Answer:
A National Commission was formed to examine the status and problems of Indian women. Report of this Commission is called as “Towards Equality Report 1974”.
Objectives of the Towards Equality Report

To examine the Constitutional, legal, and administrative provisions that have a bearing on the social status of women, their education and employment.
To assess the impact of these provisions during the last two decades on the status of women in the country, particularly in the rural sector and to suggest more effective programmes.
To consider the development of educations among women and determine the factors responsible for the slow progress in some areas and suggest remedial measures.
To survey the problems of the working women including discrimination in employment and remuneration.
To examine the status of women as housewives and mothers in the changing social pattern and their problems in the sphere of further education and employment.
To undertake survey of case studies on the implications of the population policies and family planning programmes on the status of women.
To suggest any other measures which would enable women to play their roles to the fullest in building up the nation.

2nd PUC Sociology Inclusive Strategies Ten Marks Questions and Answers

Question 1.
Explain the importance of towards equality report 1974.
Answer:
The study collected extensive data related to the various aspects of women. Trends observed by the study were surprising. Excepting the fields of education and employment where women belonging to the middle class achieved moderate success, almost all fields showed a very low percentage of participation by women. Many worked longer hours for meagre wages. Almost all women actively participated in economic activities without any financial benefit. In addition, they were also responsible for taking care of their family members especially children and the elderly.

It was believed that, due to the equality principle adopted by the constitution acted in favour of women who were denied equal rights earlier. It was felt that, Indian women enjoy equal status on par with men and also enjoy the support of men in their endeavours. In reality, only the middle class women were able to achieve some amount of progress in the fields of education and employment. But, a majority of Indian women still were victims of violence, dowry, gender discrimination etc., thus, the report of the Commission exposed hard realities.

The Commission expressed concerns about issues related to women like, lower rates of life expectancy, declining sex ratio, high death rates, low participation in economic activities etc. Such trends were against the accepted goals of our Constitution. Another point to be noted here is that, during the 19th century, women were seen as the victims of certain social evils and in the post-independence period they were seen as beneficiaries of development projects but not as active participants of development projects. Their role in the national movement, labour movements and peasant movement is ignored.

As observed by the scholars political parties accepted the role of women in building a new society in a rather reluctant way. Report of the Commission influenced the policies of the government while formulating developmental projects. It identified certain wrong notions about the role and status of women. It was a starting point for many future studies of women with a fresh perspective.

Question 2.
Mention any ten Constitutional provisions relating to upliftment of SCs and STs.
Answer:
Constitutional provisions relating to the above said groups are as follow:

Article 15: The state shall not discriminate against any citizen on the grounds of Religion, Race, caste sex, place of birth or any of them. The removal of any disability, restriction or condition with regard to access to shops, public restaurants, hotels and place of public entertainment or the use of wells, tanks, roads, and place of public resort maintained wholly or partly out of state funds or dedicated to the use of general public.
Article 16: There shall be equality of opportunity for all citizens in matter relating to employment or appointment to any office under the state.
Article 17: Untouchability is abolished and its practice in any form is forbidden. The enforcement of any disability arising out of untouchability shall be an offence punishable in accordance with law.
Article 23: Illegalizes traffic in human beings and forced labour.
Article 25 B: Hindu religious institution of public characters is open to all classes and sections of Hindu.
Article 29: Cultural and linguistic minority has right to conserve its language or culture. The article provides protection to scheduled tribe communities to preserve their languages, dialects and cultures. The state would not by law enforce upon it any other culture or language.
Article 46: The state shall promote with special care the educational and economic interest of the weaker sections of the people and in particular of the scheduled castes and scheduled tribes and shall protect them from social injustice and all forms of exploitation.
Article 164: provides for a separate ministry in charge of welfare of scheduled castes and scheduled tribes and backward classes.
Article 325 of part XV: It guarantees to all citizens of India the right to vote.
Article 330, 332 and 334: Provides seats shall be reserved for Scheduled Castes and Scheduled Tribes in the house of people and state legislature.
Article 335: It mentions the claim of Scheduled Castes and Scheduled Tribes to services and posts.
Article 338: Empowers the central government to appoint a commission for Scheduled Castes and Scheduled Tribes.
Article 339: Empowers the president to appoint a commission to report on the administration of the scheduled areas and the welfare of scheduled tribes in the states.
Article 341: Empowers the president to specify the castes, races or tribes deemed as Scheduled Castes in a particular state or union territory.
Article 342: Empowers the president to specify the tribes deemed to be Scheduled Tribes in a particular state or union territory.

Question 3.
Explain the developmental programs for the upliftment of Scheduled Castes.
Answer:
1. Appointment of a National Commission for the Welfare of Scheduled Castes and Tribes: A National Commission for the Scheduled Castes and Scheduled Tribes has been set up by the Central Government to safeguard the interests of the SCs and STs. It functions as an advisory body on issues and policies related to the development of the SCs and STs.

2. Educational Opportunities: Due attention is paid to extend the educational opportunities of SCs and STs and hence special provisions have been made in this regard. Free education, free distribution of books, stationery, uniform etc. giving scholarships, educational loan facilities, providing mid-day meal, arranging for free boarding and lodging facilities, reserving seats for SCs and STs in all the government and government aided institutions, etc.

3. Expansion of Economic Opportunities: Government has taken up economic programmes also for the benefit of SCs and STs. Examples: Landless SC labourers are allotted land. Land reforms have been undertaken to bring benefits of land ownership for them. Poor SC farmers are supplied with seeds, agriculture implements, fertilizers, pesticides, interest-free loans, pair of bullocks for ploughing, subsidy for developing dairy farming, poultry farming, piggery, animal husbandry, handicrafts, spinning and weaving.

4 Expansions of Employment Opportunities and Reservation: In order to enhance the economic position of the SCs and STs the Constitution has provided for the reservation in services. Reservation exists in all these for the SCs and STs to the extent of 15% and 7.5% respectively.

5. Upliftment of Scheduled Castes through Five Year Plans: The welfare of the Scheduled Castes has been given special attention in the Five Year Plan. The Central Government sponsored a comprehensive three strategies for the development of the SCs during the 6th Five Year Plan [1980-85]. This consisted of three schemes:

Special Component Plan [SCPs]
Special Central Assistance [SCA]
Scheduled Development Corporation (SCDCs).

1. Special Component Plan [SCP]: The main objective of this plan is to assist the SC families to improve their income substantially. This plan envisages identification of schemes of development which would benefit SCs, quantification of funds from all programmes of specific targets as to the number of families to be benefited from these programmes.

2. Special Central Assistance [SCA]: The main purpose of this scheme is to provide additional assistance to the States from the Centre to help the economic advancement of the maximum possible number of Schedule Caste families living below the poverty line.

3. Scheduled Caste Development Corporation (SCDC): These SCDCs provide money and loan assistance to SC families and help them to increase the flow of funds from financial institutions to SC families. These Corporations established in the States are expected to act as interface between the SC families and financial institutions including banks. Both the Central and the State Governments contribute grants to these SCDCs.

Question 4.
Explain the Tribal welfare programs in India.
Answer:
The following welfare programmes are implemented for the upliftment of tribals.

I. Economic Programmes: The amount allocated for the tribal welfare schemes in different plans go to prove, that tribal development is one of the priorities. The amount allocated in fifth plan was Rs. 1100 crore and it was Rs. 5535 crore and Rs. 10.500 crore in the sixth plan (1980-85) and seventh plan (1985-90) respectively.

(a) The 20-point Programme: The 20-Point Programme too focused attention on the development of Scheduled Tribes. Economic assistance was lent to tribal families to move above the poverty line.

(b) Establishment of LAMPS and TRIFED: To relieve the tribals of the bonded labour system. The bonded Labour System (Abolition) Act. 1976 was passed. That apart, to loosen the grip of the moneylenders and the middlemen on the tribals, the government organized “Large Area Multi-Purpose Societies” (Lamps). These were intended to provide adequate credit facilities for- productive purposes.

These were the Co-operative societies helping tribals in selling their agricultural and minor forest produce and providing them with improved varieties of seeds, manure, -insecticides, agricultural implements, etc. For marketing the tribal produce, the “Tribal Co-operative Marketing Development Federation of India” (TRIFED) has been set up. It works to eliminate exploitation of tribals and realization of better prices.

(c) Assistance to Agriculture: Tribals cultivation is uneconomic and also unscientific. They are being persuaded to take up scientific agriculture. Agricultural implements, manure, seeds and loan facilities are being provided and tribals are also given land rights.

II. Educational Programmes: They are also provided with free hostels, faculties such as free tuition, stipends, scholarships, mid-day meals, text-books, etc. “Ashrama Schools” with lands attached to them and “Technical Schools” have come up in tribal areas. They are also given training free of cost in poultry, forestry, animal husbandry, Apiculture, etc. Tribal-students taking competitive examinations are given pre-examination training free of cost.

There are pre-examination training centers and coaching- cum-guidance centers exclusively meant for students of Scheduled Tribes. National Council of Educational Research and Training (NCERT) and Central Institue of Indian languages Mysore have already prepared teaching module for more than 60 tribal dialects to popularize education among the tribals.

III. Research Programmes: For the study of tribal in scientific way Tribal Research centres have been set up. There are at present 11 such centres in India. To co-ordinate their activates, a 30 member “Central Research Advisory Council” has also been set up. The council provides guidance on policy formulation.

IV. Health, Housing and Other Schemes: Under various schemes, houses and sites have been given to the tribals. There are a number of voluntary organizations working for the welfare of tribals. For instance, Dr. H. Sudarshan’s Vivekananda Girijana Kendra and Karuna Trust has done a commendable work in the upliftment of Soliga, a tribal community inhabiting Biligiri Ranga Hills in Chamarajnagar district of Karnataka. They are helping in Education, Health and Empowerment of Soligas.

Question 5.
Briefly explain the Shri Mahila Griha Udyog Lijjat and SEWA.
Answer:
Shri Mahila Griha Udyog Lijjat Papad, popularly known as Lijjat, is an Indian women’s cooperative involved in manufacturing of various fast moving consumer goods. The organisation’s main objective is empowerment of women by providing them employment opportunities. Started in the year 1959 with a seed capital of Rs. 80, Lijjat has an annual turnover of around Rs. 6.50 billion in 2010, with Rs. 290 million in exports. It provides employment to around 42,000 women. Lijjat is headquartered in Mumbai and has 67 branches all over India.

Lijjat is primarily a cottage industry, urban by its origin, that has spread to the rural areas. It is considered as one of the most remarkable entrepreneurial initiatives by women that are identified with female empowerment in India. Self-Employed Women Association – SEWA: Self-Employed Women Association has made significant contribution to the empowerment of women. It has strived to integrate self-employed poor women with the mainstream economy through the twin strategies of struggle and development.

Women are the worst victims of poverty. Poor women generally experience a The self-employed women of Ahmadabad organized and formed the Self-Employed Women Association – (SEWA) in 1972. The motivation and guidance was furnished by the leadership Ela Bhatt. SEWA has strived to create conditions of full employment and self-reliance for all its members.

The central concern of SEWA has been to secure the existence of its members by furnishing financial support in the form of micro-credit to self-employed women. In order to provide finance facility at the earliest The Shri Mahila SEWA Sahakari Bank Ltd. Was registered in 1974. In the beginning SEWA Bank started functioning in urban areas; later on it extended its operations to rural areas. SEWA began its activities in rural areas in 1975.

Question 6.
Explain the role of Mahatma Gandhi and Dr. B. R. Ambedkar in eradicating untouchability?
Answer:
Freedom struggle and eradication of untouchability were both very important for Gandhij i. He preached against untouchability and has set a model by his deeds and words. He himself lived with the Harijans and shared their sorrows and sufferings. He made them participate in worships, prayers, keerthanas. He wrote extensively in ‘Harijan’ and ‘Young India’ about the condition of Harijans and propagated in favour of various legal provisions against several kinds of injustice meted out to the untouchables.

He cleaned the streets and toilets of Scheduled Castes. By his selfless, sincere self effort he created awareness among the Harijans regarding cleanliness, sanitation and health. After 1931, Indian National Congress set up a council to consider the problem of untouchability.

It was due to ceaseless effort of this council that ‘Harijan Sevak Sangh’ came into existence. The sangh also provides the Harijan students with financial assistance and scholarships. Kasturaba Balika Ashram in Delhi, Harijan Balika Vidyalaya at Sabarmati are just two examples . of schools started by the Sangh for the cause of female education. The Sangh has branches all over the country and it is maintaining 120 boarding houses. Gandhiji called untouchables as UED Harijana and popularised the word Harijana.

The word Harijana was first coined by Gujarathi saint Narasimha Mehatha. Dr. B.R. Ambedkar, popularly known as Baba Saheb stood for the emancipation of untouchables. Being the chief architect of the constitution, he has legalised the upliftment of the untouchables through the inclusion of many articles. The constitution of India guarantees, protects, and safeguards the rights and interests of all in general and of untouchables in particular.

Ambedkar wanted to instil in the hearts of untouchables, the ideas of self-dignity, self-confidence and self-respect. For the very same purpose he had started the ‘Bahishikrita Hitakarini Sabha’. The movement he had started was known as ‘self-respect movement’. In order to attain a respectable position in society, he asked untouchables to follow five principles, i.e., Pancha Sutras’. They are; Self Improvement, Self-Dependence Self-Respect Self-Confidence Self Progress.

In order to create awareness among the untouchables Ambedkar started a paper called Mooka Nayaka. He brought them under one banner; organized ‘All-India Depressed Classed Conference’ in 1942 at Nagpur. In his Dalit movement, Ambedkar suggested three principles: Education, Agitation and Organization.

Question 7.
Explain the role of sulabha souchalaya in Empowering scavengers?
Answer:
Sulabh International is an Indian based social service organization which works to promote Rehabilitation of manual Scavengers human rights, environmental sanitation, non-conventional sources of energy, waste management and social reforms through education. Sulabh was founded by Dr. Bindeshwar Pathak in 1970.

Innovations include a scavenging- free two-pit flush toilet (Sulabh Shauchalaya); safe and hygienic on-site human waste disposal technology; a new concept of maintenance and construction of pay-&-use public toilets, popularly known as Sulabh Complexes with bath, laundry and urinal facilities being used by about ten million people every day and generates bio-gas and bio-fertilizer produced from excreta-based plants, low maintenance wastewater treatment plants of medium capacity for institutions and industries.

Other work includes setting up public school in New Delhi and also a network of centres all over the country to train boys and girls from poor families, specially scavengers, so that they can compete in open job market. The United Nations Centre for Human Settlements has praised Sulabh’s sanitation system as a “Global Urban Best Practice” at the Habitat-II conference held at Istanbul (Turkey), in June, 1996.

The Economic and Social Council of the United Nations granted Special Consultative Status to Sulabh in recognition of its work. Sulabh claims their plan on human waste disposal and social reforms has provided jobs directly to 35,000 people, and made 240 towns scavenging free.

Question 8.
Explain the strategies of Women Empowerment.
Answer:
The strategies for empowerment of women can be classified as legal, social and economic.
(1) Legal Strategies: After the independence several laws were drafted with the aim to treat women on par with men. Some of the legislation are as follows :

Hindu Marriage Act of 1955
Hindu Succession Act of 1956.
Hindu Adoption and Maintenance Act of 1956.
Dowry Prohibition (Amendment) Act 1984
Domestic Violence Act 2005 etc.

(2) Social Strategies: Social strategies are as follow:

Establishment of Women Welfare Services.
Legal literacy of women through mass media.
Help of neighbours to be sought in the cases of abused women.
Conducting public education and awareness programmes in order to help women.
Males are also to be educated to realize their new roles in the changed times and the necessity of their own contribution to family life.

(3) Economic Strategies: Economic strategies are as follow:

Educational and vocational training for women which enable them to seek jobs and become economically dependent.
Technological aids that will be labour saving devices and will lighten women’s burden of heavy daily tasks.
Train women in both formal and non-formal education.
Credit facilities to start small-scale industries/self-employment.
Programmes of placing women in important positions at various levels.

2nd PUC Sociology Question Bank Chapter 2 Social Inequality, Exclusion and Inclusion

November 2, 2023

Karnataka 2nd PUC Sociology Question Bank Chapter 2 Social Inequality, Exclusion and Inclusion

You can Download Chapter 2 Social Inequality, Exclusion and Inclusion Questions and Answers, Notes Pdf, 2nd PUC Sociology Question Bank with Answers Karnataka State Board Solutions help you to revise complete Syllabus and score more marks in your examinations.

2nd PUC Sociology Social Inequality, Exclusion and Inclusion One Mark Questions and Answers

Question 1.
Who Popularized the term Harijan?
Answer:
MahathamaGandhiji.

Question 2.
Who Advocated the policy of Isolation?
Answer:
Verrier Elwing.

Question 3.
Who Advocated the Policy of Tribal Panchasheela?
Answer:
Jawaharlal Nehru.

Question 4.
Name anyone Backward Classes Commission appointed by the Government of India.
Answer:
Mandal Commission.

Question 5.
Who Introduced the concept of Dominant Caste?
Answer:
M.N Srinivas.

Question 6.
Mention any one category of backward classes in India.
Answer:
Scheduled castes.

Question 7.
Who wrote “Caste and race in India”?
Answer:
G.S. Ghurye.

Question 8.
Who wrote “People of India”?
Answer:
Herbert Risley.

Question 9.
Who wrote caste in India?
Answer:
J.H. Hutton.

Question 10.
Who wrote “History of caste in India”?
Answer:
S.V. Kethkar.

Question 11.
Who called tribals as Backward Hindus?
Answer:
G.S. Ghurye.

Question 12.
Who was the chairman of First Backward class commission in India?
Answer:
Kalalker.

Question 13.
Who was the chairman of Second Backward class commission in India?
Answer:
B.P. Mandal.

Question 14.
In Karnataka which was the First Backward class commission?
Answer:
Naganna Gowda Commission.

Question 15.
Who is the present chairman of permanent Backward class commission of Karnataka?
Answer:
H. Kantharaj.

Question 16.
Which state has large number of scheduled Tribes? ‘
Answer:
Madhya Pradesh (23.27).

Question 17.
Which state has highest percentage of Scheduled Tribes?
Answer:
Mizoram (94.75).

2nd PUC Sociology Social Inequality, Exclusion and Inclusion Two Marks Questions and Answers

Question 1.
What is social capital?
Answer:
Social capital in the form of networks of contacts and social associations. Someone with influential relatives and friends (social capital) may – through access to good advice, recommendations or information – manage to get a well-paid job.

Question 2.
Name any two tribes of the southern zone.
Answer:
Kadu kuruba, Hakki-Pikki.

Question 3.
Define Tribe.
Answer:
Tribe in the dictionary of Anthropology is defined as “a social group Usually with a definite area, dialect, cultural homogeneity and unifying social organization. It may include several sub-groups such as sibs or villages”.

Question 4.
How is the word caste derived?
Answer:
The word caste is derived from the Spanish/Portuguese word “CASTA”, which means breed, Race, strain or a complex of hereditary qualities. The Portuguese applied the term to the classes of people in India known by name of Jati. The English word caste is a modification of the original term system CASTA.

Question 5.
Who coined the term Scheduled Caste?
Answer:
The term scheduled caste was coined by the Simon commission in 1928 which came to be used for the people described as untouchables and was used by the then Government of Indian Act 1935. According to Ambedkar, in early India, they were known as Broken men or outcastes. The British described them as “depressed classes”.

Question 6.
Define Prejudices.
Answer:
Prejudices refer to pre-conceived opinions or attitudes held by members of one group towards another. The word literally means ‘pre-judgement’, that is, an opinion formed in advance of any familiarity with the subject, before considering any available evidence.

Question 7.
Give the meaning of social inequality.
Answer:
Patterns of unequal access to social resources are commonly called social inequality.

Question 8.
Mention one definition of caste.
Answer:
M.N. SRINIVAS defines caste as a “Hereditary, endogamous, usually localized group, having a traditional association with an occupation and a particular position in the local hierarchy of castes. Relation between castes is governed, by the concept of pollution and purity, and generally maximum commensality occurs within the caste.

Question 9.
Mention two dominant castes of Karnataka.
Answer:
Vokkaligas and Lingayath.

Question 10.
Name any two Tribals mentioned in Vedic literature.
Answer:
Bharathas and Kinnaras.

Question 11.
Mention any two changes in Caste system.
Answer:
Occupational and food restriction are relaxed

Question 12.
Mention any two social reform movements.
Answer:
Brahma Samaja, Arya Samaja.

Question 13.
What is social exclusion?
Answer:
Social exclusion refers to ways in which individuals may become cut off from full involvement in the wider society.

Question 14.
Mention any two causes for changes in the caste system.
Answer:
Education and Industrialization.

Question 15.
Mention any two determinants of dominant caste.
Answer:
Preponderates Numerically over other castes and high

Ritual status in local.
hierarchy

Question 16.
Write any two problems of Scheduled Caste.
Answer:
Restriction to access public facilities and Restriction to Read Holy Scriptures.

Question 17.
Mention the three zones of tribals.
Answer:
The North and the Northeastern Tribal zone.

The Central Tribal Zone
The Southern Tribal Zone

Question 18.
Mention any two problems of Scheduled tribes.
Answer:
Geographical Isolation and Exploitation ofTribalbyNon-Tribal.

Question 19.
Mention the three views on the tribal welfare.
Answer:
The policy of Isolation, The policy of Assimilation and policy of Integration.

Question 20.
Give any two criteria of backwardness.
Answer:
Educational Criteria

Children between 5 and 15 years never attending school 25% above the state average.
Student drop-out rate 25% above the state average.

Question 21.
State any two reform movements affects the caste system.
Answer:
Brahma Samaja, Sathya Shodaka Samaja.

Question 22.
Define the concept of dominant caste.
Answer:
According to M.N. Srinivas “A caste is dominant when it preponderates numerically over the other castes, when it also wields preponderant economic and political power, and when it enjoys a high ritual status in local caste hierarchy”.

Question 23.
Define the Backward class or caste.
Answer:
The term backward class refers to those social groups or classes or castes, which are characterized by low literacy or lack of education, poverty, exploitation, non representation in services and untouchability. It may be also called as a social category which consists of all the socially, educationally, economically and politically backward groups, castes and tribes.

Question 24.
State any two scheduled castes of Karnataka.
Answer:
Holayas and Bhories.

Question 25.
State any two scheduled Tribes of Karnataka.
Answer:
Kadu Kuruba and Siddis.

Question 26.
Write any two definition of tribes.
Answer:
Tribe in the dictionary of Anthropology is defined as “a social group usually with a definite area, dialect, cultural homogeneity and unifying social organization. It may include several sub-groups such as sibs or villages”.

Question 27.
State the two Backward class commissions of India.
Answer:
Kalalker commission and Mandal Commission.

Question 28.
Mention any two Backward class commissions of Karnataka.
Answer:
L.G. Havannor Commission, Venkataswamy Commission.

Question 29.
Define other Backward classes or castes?
Answer:
Other Backward Classes (Castes) comprise the non-untouchables, lower and intermediary castes, who were traditionally engaged in agriculture, animal husbandry, and handicrafts, services, and other castes. The OBCs do not constitute a homogeneous category. There are many divisions within the overall category. The other backward classes are not classes at all, but group of communities. The OBCs constitute 51% of the total population of the country.

Question 30.
What do you mean by creamy layer?
Answer:
Creamy layer is used to refer to the relatively wealthier and better educated members of the Other Backward Classes (OBC’s) who are not eligible for Government sponsored Educational and professional benefits. The term was first introduced by the Sattanathan Commission in 1971 which directed that the ‘creamy layer’ should be excluded from the reservation (quotas) of civil posts and services granted to the OBCs.

Question 31.
How many caster and Tribes are enlisted in Karnataka as SCS & STS?
Answer:
101 SCs and 50 STs.

2nd PUC Sociology Social Inequality, Exclusion and Inclusion Five Marks Questions and Answers

Question 1.
Explain social inequality and social exclusion.
Answer:
Patterns of unequal access to social resources are commonly called social inequality. Social inequality is not the outcome of innate or ‘natural’ differences between people, but is produced by the society in which they live. People often face discrimination and exclusion because of their gender, religion, ethnicity, language, caste and disability. People often harbour prejudices about other social groups.

Often these ideas reflect prejudices. Prejudices refer to pre-conceived opinions or attitudes held by members of one group towards another. The word literally means ‘pre-judgement’, that is, an opinion formed in advance of any familiarity with the subject, before considering any available evidence. Social exclusion refers to ways in which individuals may become cut off from full involvement in the wider society.

It focuses attention on a broad range of factors that prevent individuals or groups from having opportunities open to the majority of the population. Social exclusion can be defined as a situation in which multiple deprivations prevent individuals from participating in important activities. Thus socially excluded might be unable to find work, or actively participate in a society. Social exclusion is not accidental but systematic – it is the result of evolved structural features of society.

Question 2.
Briefly explain changes in caste system during British rule.
Answer:
The impact of British rule on caste system in India may be studied under the following heads.

Introduction of Universalistic Legal system
Impact of English Education
Impact of Social Reform Movement
Influence of New Social Formation
Impact of Freedom Struggle
Impact of Industrialization and Urbanization

1. Introduction of Universalistic Legal System: The establishment of British courts removed authority from the purview of caste panchayats. They introduced a new principle of justice, according to which all are equal before the law, and the caste panchayat in proportion lost their former importance. Some of the legislation which brought changes in the caste system can be made as the, following.

The Caste Disabilities Removal Act of 1850 This act served to remove some of the disabilities associated with castes including the practice of untouchability.
The Hindu Widow Remarriage Act 1856, This act made legal provision for the Hindu widows to remarry.
The Special Marriage Act of 1872 which considered marriage as a civil contract and legalized inter-caste or inter-religious marriage.
Other Legislative and Administrative Measures. In 185 8 British government announced that all schools maintained by the government shall be open to all the classes of its subjects without any discrimination. In 1923, the government issued a resolution that no grants would be paid to any aided educational institutions, which refused admission to the children of the depressed classes.

In 1925, a bill was passed by the Madras legislative council, throwing open all public office, well, tank, or place of public resort, to all classes of people including the depressed. Montogue-Chelmshford reforms made constitutional provisions for the special representation of depressed classes in the local as well as in the legislative bodies.

As education spread to the lower strata, it kindled libertarian impulses among them. Western education provided an indispensable passport to the new economic opportunities. Members from the lower castes became different professionals and took new commercial opportunities offered by the western education.

3. Impact of Social Reform Movements: Social reforms movements brought changes in ‘the caste system in British period. They were set out to eradicate caste and to establish a casteless and, classless society. They were against the fictitious difference between caste. They attacked the tendencies of separatism and Inequality in the caste system. Some important social reform movements are:

The Brahma Samaj founded by Raja Ram Mohan Roy
Prarthana Samaja’s Athma Rama Pandurang Justice M. Ranade
The Arya Samaja founded by Swamy Dayananda Saraswathi
The Ramakrishna Mission represents the synthesis of the ancient and the modern thoughts. Swamy Vivekananda started Ramakrishna Mission.
Other Reform Movements such as Annie Beasant’s Theosophical society, Maharshi Arabindo Ghosh’s Divine life Society and etc. Thus all these organizations aimed at the destruction of caste system and social re-construction of Indian society.

4. Impact of New Social Formations: The new economic system brought about a new grouping of the population in the economic sphere. The Indians could be differentiated into such categories as capitalists, workers, peasants, propritiators, merchants, tenants, land lords, doctors, lawyers, teachers and technicians Each category being composed of individuals belonging to various castes, but having identical material and political interests. This division weakened the vertical caste lines.

Thus there came into existence such organization as Mill Owners Associations, All India Trade Union Congress, All India Kishan Sabha and etc., these groups struggled for their own interests. In the process of this struggle they developed a new consciousness and outlook and a new solidarity, which slowly weakened the caste consciousness.

5. Impact of Freedom Struggle: The growth of the nationalist movement played a great role in weakening caste consciousness. In India, the presence of foreign rule was a permanent stimulus to the Indians to unite on a national basis. Thus the growth of the national movement undermined the caste consciousness.”

6. Impact of Industrialization and Urbanization: The growth of Industries destroyed the old craft and provided new ways to earn a livelihood. Occupational mobility and movement from compact ancestral village started breaking down in the caste norms. New transport facilities, specially crowed trains and buses, which threw together millions of people of all castes and left little room for the necessities of ceremonial purity.

Taboos on food and water gradually weakening when industrial workers belonging to various castes started working under one roof. The demarcation observed by the members of different castes regarding eating food, physical contact with those of other castes, steadily crumbled in cities.

Question 3.
Explain the determinants of dominant caste.
Answer:
M.N. Srinivas introduces the concept of “Dominant Castes” which is of great help in understanding inter-caste relations and conflicts in Indian society. According to M.N. Srinivas “A caste is dominant when it preponderates numerically over the other castes, when it also wields preponderant economic and political power, and when it enjoys a high ritual status in local caste hierarchy”. Nature of Dominant Castes

(a) Determinants of Dominance: A dominant caste should own a sizeable amount of the land and it should enjoy greater economic and political power. In addition to this, a number of educated persons found in the caste and the nature of high occupation people pursue in the caste add to the dominant caste. When a caste enjoys all the elements of dominance, i.e. numerical strength, economic and political power, high ritual status, it is said to be dominant in a decisive way.

(b) Distribution of Dominance: Different elements of dominance are distributed differently among different castes in a village. For example, a caste, which is numerically high, maybe poor and lacking in political power, while a ritually high status caste may be rich economically and lacking strength in numbers. It can also be said that when a caste enjoys one form of dominance, it is frequently able to acquire other form of dominance.

(c) Dominance is Not Purely a Local Phenomenon: As M.N. Srinivas says in Rural India dominance is purely a local matter. A caste group, which has only a family or two in a particular village but enjoys decisive dominance in the wider region. Because the caste members of these families maintain a network of ties with the dominant relatives found in the wider region.

(d) New Factors Affecting Dominance of Caste: According to M.N. Srinivas, western education, jobs in the administration and urban sources of income are also significant in contributing to the prestige and power of particular caste groups in the village.

(e) Dominant Caste at the State Levels: Dominant castes, such as Lingayats and Vokkaligas in Karnataka, Reddys and Kammas in Andhra Pradesh, Nairs and Ezhavas in Kerala, Gounder, Padayachi and Mudaliars in Tamil Nadu, Marathas, Brahmins and Mahars in Maharashtra, Rajputs, Jats, Takurs, Gujars, Baniyas, Bhoomihars etc., in the North Indian states.

Question 4.
Discuss the changing concept of tribe.
Answer:
(a) Tribe as Homogeneous, Self-contained Unit: The Tribals are believed to be the original inhabitants of Indian Peninsula. They are generally called ‘adivasis’ which means original inhabitants. Vedic literature mentions various Tribes like the Bharathas, the Bhils, the Kolias, the Kirathas, the Kinnaras, the Matsyas, and the Nishadas. A Tribe was a homogeneous a self-contained unit without any hierarchical discrimination.

Each Tribe was organized under a chief. Most capable was elected as the chief and his continuation as military leader depended much upon his skill in war and defence. This process heralded the emergence of little republics and monarchies. Each Tribe had its own system of administration. Tribal chief exercised considerable influence over social, economic and religious affairs of the Tribe. Tribal councils were vested with legislative, judicial and executive powers.

(b) Tribe as a Political Division: The concept Tribe derives its origin from the Latin term ‘Tribuz’ means three division. For Romans, the Tribe was a political division. The Tribe was the highest political unit comprising several districts which in turn were composed of class It was a territorial organization exercising control over its people. The territory under the domain of a particular Tribe was generally named after it.

Thus, it is presumed that the name Bharath is derived from the mighty Bharatha Tribe. Similarly, the matsya kingdom of 6th B. C. The Minas of Rajasthan and Madhya Pradesh are believed to be the descendants of the matsya Tribe. Mizoram, Nagaland and Tripura are named after the Mizo, the Naga and the Tripuri Tribes. Similarly, Santhal Paraganas, Gondawana, Lahaul and Kinnaur derive their names from the Santhalas, the Gondas, the Lahaulas and the kinnaras.

(c) Tribe as a Race: Race is used to designate a category of persons whose similar characteristics could be attributed to common descent. The Tribes in India broadly belong to three stocks namely, the Negritos, the Mongoloids and the MediterraneAnswer: The negritos are believed to be the earliest inhabitants of the Indian Peninsula. The traces of this race are found among the onges, Andamanese, jarwas of Andaman and Nicobar Islands and also among Kadars, Irulas and Paniyans of South-India.

They are generally known as the ‘Dravidians’, Tribes belonging to the Dravidian race are found both in southern parts and central parts of India. Dravidians are known as the original inhabitants of India. They speak Kannada, Tamil, Telugu and Malayalam or corrupt form of these languages.

(d) Tribe and Scheduled Tribe: With a view to have classified information about the Tribals, the Britishers conducted census operations in the tribal areas. A sub-heading called ‘Forest Tribes’ was formed under the category of ‘Agricultural and Pastoral Castes’ in the census report of 1891 by the Commissioner of Census J. A. Bains. Since then, in the subsequent census reports of 1901, 1911, 1921, 1931 and 1941 Tribals have been classified as ‘Animists’, ‘Tribal Animists’, ‘Hill and Forest Tribes’, ‘Primitive Tribes’ and ‘Tribes’ respectively.

As per Article 366 (25) of the Constitution of India, Scheduled Tribes means such Tribes or tribal communities or parts of or groups within such Tribes. Article 342 empowers the President of India to specify the Scheduled Tribes by a public notification. The Parliament may, by law, include or exclude from the list of Scheduled Tribes any tribal community or part thereof in any state or union territory.

The foregone analysis makes it clear that the concept of Tribe has undergone a change from that of a political unit of older days to a group of people identified with poverty and backwardness. Though grouping together of tribal communities under the constitution has helped them in consolidating their position as a distinct ethnic, linguistic and cultural unity.

Question 5.
Explain the Geographical distribution of Indian tribes.
Answer:
(i) The North and the North-Eastern Tribal Zone This zone comprises the Sub-Himalayan Region and the Mountain Ranges of the North-Eastern Frontier of India, the Tista valley and the Jamuna-padma, portion of the Brahmaputra. It includes Himachal Pradesh, Northern UP, Sikkim and the seven states of the Northeast consisting of Arunachal Pradesh, Assam, Meghalaya, Mizoram, Nagaland, Manipur and Tripura. They belong to the Mongoloid race and their language resembles the languages of Austric family.

This zone is inhabited by the Tribes such as Gurung, Limbu, Lepcha, Aka, Mishmi, Mikir, Rabha, Kachari, Garo, Khasi, Chakmas, Naga, Angami, Serna, Pham, Chang and so on. Spinning, weaving and agriculture are the predominant occupations of this zone. Nagas are having patriarchal family: Khasi and Gharos are having matriarchal family. Some tribes of this zone practice polyandry. However, monogamy is the usual practice in this zone. Nagas are the occasional head-hunters.

(2) The Central Tribal Zone: The central zone comprises plateau and mountains belt between the Indo-Gangetic plains to the North and the Krishna River in the South. It includes West Bengal, Orissa, Bihar, Southern UP, Rajasthan, Gujarat, Madhya Pradesh and Maharashtra. The important Tribes among these are the Gonds of Madhya Pradesh, Bhils of Rajasthan, Santhalas of Chotanagpur, Ho of Singhbhumi, Manbhumi, Khond and Kharia of Orissa, Sawara of Ganjam and the Mundas. Madhya Pradesh has the largest concentration of tribal population (23.27%). Santhalas of this zone are more advanced Tribe. Some of the Tribes are engaged in small-scale cottage industries and settled form of cultivation. Some of them live in very dense forests and difficult terrains.

(3) The Southern Tribal Zone These are the Tribes of South India (Andhra Pradesh, Tamilnadu, Karnataka, Kerala and two Union territories Andaman and Nicobar Islands and Lakshadweep). The Tribes of this zone are the original inhabitants of India and these people speak Dravidian languages. This zone consists of the Tribes like Chenchu, Kota, Kurumba, Badaga, Toda, Kadar, Malaya, Muthuran, Koya, Soliga, Kannikar, Paniya, Yeravas, Irula, Kadu Kuruba, Jenu Kuruba, Akki Pikki, etc.

Todas of Nilagiri practice fraternal polyandry and in some Tribes there is matriarchal type – of social organization. In the Andaman and Nicobar islands, there are six Tribes namely the great Andamanees, the Onges, the Sentinelese, the Jarwas represent the Negritos race and the Nicobaresand Shompens belong to the Mongoloids race. The Nicobares numbering about 22000 are comparatively an advanced Tribe and are settled in the Nicobar Islands. The remaining five Tribes are numerically very small and have been declared as the primitive Tribes.

Question 6.
Discuss three views, on tribal welfare.
Answer:
The Tribal problems have been approached from three points. They are as follows:

The Policy of Isolation
The Policy of Assimilation
The Policy of Integration.

(1) The Policy of Isolation: This policy favoured Isolation of Tribals from the mainstream society. J.H. Hutton, who was a commissioner for census of 1931, gave, a solution to the tribal problems of uncontrolled acculturation. He suggested the creation of self-governing tribal areas with free power of self-determination. Verrier Elwin suggested the creation of “National Parks” where the tribal people could safely live without being victims of Over-Hasty and Unregulated Process of Belief, and Civilization.

Both Hutton and Elwin were severely criticized for recommending this policy of Isolation, which was looked upon as proposal to create a museum or a zoo, instead of helping the tribal people to utilize the resources of knowledge and improve the conditions of their life.

(2) The Policy of Assimilation: The social reformers like Takkar Bapa, G.S. Ghurye some voluntary organizations and Christian missionaries advocated this policy. They have recommended for the assimilation of these tribal groups either into Christianity or into Hinduism. According to Takkar Bapa tribal problem could be solved only through contact with more advanced people. Separatism and Isolation seem to be dangerous theories and they strike at the root of National Solidarity.

(3) The Policy of Integration: The only approach that would make available to the Tribes the benefit of modem advanced society and yet retain their separate identity is Integration. This policy aims at developing a creative adjustment between Tribes and non-Tribes of India leading to responsible partnership. Pandit Nehru, M.N. Srinivas, D.N. Mujumdhar and others here had supported this view.

Question 7.
Explain tribal Panchasheela.
Answer:
Jawaharlal Nehru laid down the policy of Integration to five principles (1957) in his foreword note to Verrier Elwin’s book, called “The Philosophy of NEFA” (NEFA- North East Frontier of Assam). The tribal panchasheela as enunciated by him as follows:

People should ‘develop along the lines of their own genius ‘ and we should avoid imposing anything on them. We should try to encourage in every way their own traditional – arts and culture.
Tribal rights in land and forests should be respected.
We should try to train and build up a team of their own people to work, administration and development. Some technical personnel from outside will, no doubt be needed especially in the beginning. But we should avoid introducing too many outsiders into tribal territory.
We should not over-administer these areas or overwhelm them with a multiplicity of schemes. We should rather work through and not in rivalry to their own social and cultural institutions.
We should judge the results not by statistics or the amount of money spent but by the quality of human character that is evolved.

Question 8.
Explain the criteria of Backwardness according to Mandal Commission.
Answer:
The Second Backward Classes Commission came into existence in 1979, under the chairmanship of B. P. Mandal. The Mandal Commission in its report has listed 3743 castes and communities in the central list. The commission has recommended 27% reservation for other backward castes. One of the primary objectives of the Mandal Commission was to find out the criteria to be used to determine the socially, economically and educationally backward people. The commission found out 11 criteria for determining the social, economic and educational backwardness of the communities. These criteria fall into three categories as mentioned below.

A. Social Criteria:

Social backwardness as considered by others.
Dependence mainly on manual labor for livelihood.
Marriage of 25% girls and 10% boys in rural areas, and of 10% girls and 5% boys in urban area below 17 years.
Female work participation 25% above the state average.

B. Educational Criteria:

Children between 5 and 15 years never attending school 25% above the state average.
Student drop-out rate 25% above the state average.
Matriculation rate 25% below the state average.

C. Economic Criteria:

Average value of family assets 25% below the state average.
Families living in kachcha houses 25% above the state average.
Sources of drinking water beyond500 meters for more than 50% of the families.
Consumption of loan by households25% above the state average.

These criteria were differently weighted; three points each for social indicators, two points each for educational indicators, and one point each for economic indicators, adding up to 22 points. Any caste getting more than 11 points was counted as backward. The criteria of backwardness recommended by the Mandal Commission are widely applied today to determine the relative backwardness of a community.

Question 9.
Explain the problem of other Backward classes or castes.
Answer:
Other Backward castes/classes have been suffering from number of problem since a long time. The problems, which are common to all OBC’s are as follows :

(1) Other Backward Castes (Classes) constitute an Indefinite, Abstract and Unorganized Category: First Backward class commission known as Kalalker Commission was appointed to prepare a list of communities. Kalalker’s report had listed 2399 castes as backward castes and then Government had rejected this list. The mandal commission (Second Backward Class Commission) listed 3743 castes and communities as Backward classes.

Most of the OBC communities are strangers to one another. They do not have common awareness regarding their own problems. Conflicts do arises among themselves regarding the issue of “Backwardness”. No single All-India level organization has been established. These groups are scattered all over India and exhibit lot of diversities and difficult to unite them.

(2) Economic Backwardness: Most of OBCs are economically backward like SC and ST. A large number of poor, unemployed, Under-employed are found in this category. Only a few people are self employed, but majority of them are working for poor wages. Sizable numbers are economically exploited.

(3) Educational and Social Backwardness: Illiterates are found in a large number in this category. Higher education among OBCs at low level. Even though OBCs are not directly the victims of untouchability, but lot of social distance prevails between these and so called forward castes.

(4) Politically Unorganized: OBCs are comparatively unorganized because OBCs do not comprise a single caste. Most of these castes spreaded across the nation. No single backward caste numerically dominant in any one province. Hence they are not able to work as powerful “Pressure Groups” at the all India level.

Question 10.
Write a note on creamy layer.
Answer:
Creamy layer is used to refer to the relatively wealthier and better educated members of the Other Backward Classes (OBC’s) who are not eligible for Government sponsored Educational and professional benefits. The term was first introduced by the Sattanathan Commission in 1971 which directed that the ‘creamy layer’ should be excluded from the reservation (quotas) of civil posts and services granted to the OBCs.

The Government’s decision to implement the mandal commission report on reservation for the backward castes/class was referred to the Supreme Court, which gave its judgement on November 15, 1992. The Court accepted the policy of 27 percent reservation for the backward castes/ classes, though it gave certain direction for change in this policy.

(IThe creamy layer of backward castes/ classes should be excluded, (2) Armed forces and sensitive higher civilian posts (like Scientists, University Professors, Pilots., etc) should be kept outside the purview of caste reservation, (3) The Supreme Court recognised only 1238 castes/classes as OBC and reservation only in first appointments, (4) The court directed that the reservation quota should not go beyond 50% (SC + ST + OBC: 15.50% + 7.50% + 27% = 50).

Creamy layer was kept out of the quota as directed by the Supreme Court and high posts in the creamy layer (like President, Vice President, Supreme Court, High Court Judges, Class I Officers, Members of PSUs, CEC, CAG and wards of officers working in the World Bank or International organisation etc) were also identified.

The officers working in public sector undertakings professionals, like doctors, lawyers, chartered accountants, income tax consultants, architects and computer specialists, whose annual income was more than Rs one lakh were also included in the creamy laver. However, political posts (like PM, Union Ministers, CMs, Ministers, Governors and MPs, MLAs and MLCs were not included in the creamy layer category.

Backward class movement in Karnataka – The backward class movement in Karnataka is a desire of the under-privileged people to develop their own potentialities and contribute to the economic development of the nation. In every society some groups of people are higher and some are lower due to the opportunities they have in general. By such opportunities well-off people equip themselves and pursue careers which give them prestige and profit. By contrast, the lower or other backward classes have no opportunities to equip themselves.

In 1918, a committee of six non-official members presided over by Sir Leslie Miller. Miller committee recommended the acceptance of all the demands. Since then Backward Classes in princely Mysore state have availed benefits in the field of education, employment and political arena.

(A) Naganna Gowda Commission: The Karnataka Government appointed a backward class commission in 1960 under the Chairmanship of Dr. Naganna Gowda. It is the First Backward Class Commission in Karnataka. The commission has submitted its report on 1961, which recommends 15% for SCs, 3% for STs and 50% OBCs, providing total 68% of reservation. The government attempted to implement the report was stayed by the Supreme Court. However in 1963 the government issued an order guaranteeing 15% of reservation to SCs, 3% STs and 30% to OBCs.

(B) L. G Havanoor Commission: In 1972 the government has appointed the second backward class commission headed by Sri L. G. Havanoor. This commission in its report submitted in 1975 stated that though more than 75% of the people in the state belonged to backward classes and deserved reservation facilities. There was no constitutional provision for giving it. Hence, it made provision for up to 50% reservation. Government made provision for 58% reservation. However it was challenged in Supreme Court and govt, gave a submission to court stating to initiate a new commission.

(C) Venkataswamy Commission: In 1983, the government has appointed the Venkataswamy commission, which gave its report in 1986. The report created wide spread dissatisfaction. The government decided not to implement the report but to establish a new commission to find an amicable settlement to this problem.’

(D) Chinnappa Reddy Commission: The government instituted the Chinnappa Reddy commission in 1990, which has been comparatively more widely welcomed. The commission seems to have tried its best to uphold social justice. In Karnataka, the SCs and STs together enjoyed 18% while the OBCs quota is 32%.’ Based on the Mandal commission’s report, the supreme court of India gave directions to establish a permanent Backward Classes Commission in the centre as well as in states and union territories.

Accordingly, a permanent backward classes commission was set up in Karnataka Sri K. Narayana Rai (1994-1997), Prof, Ravi Verma Kumar (1997-2000), Sri Muniraju(2001-2003), Sri Siddalingaih(2003-2006), Dr. C. S. Dwarakanath (2007-2010)N. Shankarappa (2011 -13) headed the Backward Classes Commission in Karnataka. At present H. Kantharaj is the chairman of Karnataka state Back word class commission. The commission recommends for inclusion or exclusion of a caste in the backward class list. In Karnataka 101 and 51 Triber are enlisted as scheduled caster and scheduled Tribes Respectively.

2nd PUC Sociology Social Inequality, Exclusion and Inclusion Ten Marks Questions and Answers

Question 11.
Define caste system and Explain the characteristics of caste system.
Answer:
Life of every member of the Indian society is to a large extent influenced by three systems viz., joint family, caste system and village community. They influence one’s occupation, food dress, habits, philosophy and marriage etc. The study of caste system is important because caste in India is an all pervasive and deep rooted social institution. Definitions of Caste

1. Herbert Risley has defined caste as “A collection of families of or a group of families bearing a common name, claiming a common descent from a mythical ancestor, human or divine, professing to follow the same hereditary calling and regarding by those who are competent to give an opinion as forming a single homogeneous community”.

2. S. V. Kethkar in his work “History of Caste in India”, “A caste is a group having two characteristics 1) Membership is confined to those who are born of members. 2) The members are forbidden by an inexorable social law to marry outside the group (Endogamy)”. G. S. Ghurye explains the features of caste system in his book “Caste and Race in India”, which are as follow;

1. Caste as a Segmental Division of Society: The society is divided into various castes with a well developed life of their own. The membership in caste is determined by birth. Caste has hereditary status, which is determined by birth. Each caste has a council of its own known as caste panchayat. Caste panchayts imposed certain restriction on social intercourse marriage commensal, occupational. These restrictions each caste had its own way of life. Violation of caste norms attached punishment from the caste panchayath depending on violation of caste norms.

2. Hierarchy: The whole society is divided into distinct castes with a concept of high and low, or superior and inferior is associated with this gradation or ranking. The Brahmins were placed at the top of the hierarchy and regarded as pure. The degraded castes or untouchables have occupied the other end of the hierarchy. They were subjected to manifold disabilities.

3. Restrictions on Feeding and Social Intercourse: There are minute rules as to what sort of food or drink can be accepted by a person and from what castes, who should accept food or drink at the hands of whom is defined by caste.

4. Civil and Religious Disabilities and Privileges of the Different Sections: Segregation of individual castes or groups of castes in a village is the most obvious mark of civil privileges and disabilities and it has prevailed in a more or less definite form all over India. Generally, untouchables were made to live on the outskirts. Certain parts of the town or village are inaccessible to certain castes. Restriction on using of public, roads, water facilities and Hotels etc.

5. Restrictions on Occupations: According to G.S. Ghurye every caste was associated with a traditional occupation. The technical skill of the occupation was made hereditary. Since a distinction was made between occupation being clean and unclean. The hereditary occupations reflected a caste status.

6. Restrictions on Marriage (Endogamy): Finally every caste also maintained its rank and status upon marriage relation. Inter caste marriages were prohibited. Hence they practiced endogamy. Caste is an endogamous group. “Endogamy is the essence of the caste system. Every caste was segmented into sub-castes, and these sub castes were the units of endogamy.

Question 12.
Explain the problems of Scheduled Castes.
Answer:
I. Social Disabilities:

Denial or restriction of access to public facilities, such as wells, schools and roads.
Restrictions on movement were also imposed. Untouchables were not allowed to walk on roads and streets within prescribed distance of the houses or persons of higher castes.

II. Economic Disabilities:

Exclusion from any honorable and most profitable employment and fixity to dirty or
menial occupations. –
Restrictions on style of life, especially in the use of goods indicating comfort or luxury. Riding on horseback, use of bicycles, the wearing of gold and silver ornaments, all of these were forbidden in many areas.
Liability to unremunerated labour for the higher castes and to the performance of menial services for them.

III. Religious Disabilities:

In Indian untouchables were subjected to various religious disabilities. They were prevented from entering temples, Monasteries and cremation grounds and could not make use of them because it was believed that these places would become impure by their touch and presence. The untouchable could hot worship in the temples. Their presence was considered sufficient to defile the God. They were not allowed to read and listen to the Holy Scriptures.

D. N. Majumdar summarized the position of the untouchable castes by maintaining that these castes are not depressed in all states, the same caste may be depressed in one area but may not suffer from any social and political disability in another. The disabilities are rigid where the depressed castes are numerically small, and fewer or on the decline where they numerically strong.

Where the higher castes are not numerous and the depressed castes form the bulk of Population, the degree of ceremonial pollution observed is very small and often we find few disabilities attached to the inferior castes. A caste may be depressed but individual, members of the caste who have succeeded in life and who are wealthy and own property have been admitted to a higher social status.

Question 13.
Explain the problems of scheduled tribes in detail.
Answer:
The problems of Tribals are as follows:

1. Geographical Isolation: Tribals are the people who have been living in remote areas . and hill tracks, without any access to socio-economic inputs. For centuries Tribals were isolated from the rest of the community, which has also given them wide cultural variations. Their geographical isolation from the mainstream deprived them the chances of progress.

2. Cultural Problems: Contact with outsider, the tribal culture is undergoing a change. It has led to the degeneration of Tribal life and Tribal arts such as dance, music and different types of crafts. In several tribal areas, influence of other religions have affected their culture. This also responsible for alienating the Tribals from their culture.Then tribal groups have been divided into several sects on the basis of religion. This has shattered their collective life.

3. Social Problems: Due to the influence of outsiders the Tribals are facing the problem of dowry, child marriage, infanticide and untouchability. The contact with outsiders created several social and health related problems.

4. Economic Problems: Tribal people are economically backward. The major economic problems of tribals are as follows:

Alienation of Tribal Land to the Non-Tribals
Problem of Indebtedness
Exploitation in Forestry Operations
Primitive Methods of Cultivation

5. Educational Problems: According to 2011 census, the literacy among the scheduled Tribes was 29.6 percent. Main causes of slow progress in literacy among the scheduled. Tribes are poverty of the parents, content of education, inadequate educational institutions and supporting services, absenteeism, medium of instruction and educational policy, etc.

6. Exploitation of Tribal by the Moneylenders: The Tribals continue to be the victims of exploitation by the moneylenders. Indebtedness among the Tribals may be attributed to the following reasons: Poverty Loopholes in the existing money lending laws, lack of awareness about sources of institutional finances and existing legal protection, Inability to follow complicated procedure to obtain loan and consumer credit from institutional sources. Indifferent attitude of government and bank officials, Private money lenders willingness to advance money to the Tribals without any security.

Absence of alternative credit facility has compelled the Tribals to compromise their fate with moneylenders Accept indebtedness as almost an inescapable aspect of their existence Lack of employment opportunities.
6. Health Problems: The main cause of their sickness is the lack of clean drinking water, nutritive food and prevalence of communicable diseases are major health problems.

Question 14.
Explain the major reasons for the changes in caste system.
Answer:
The impact of British rule on caste system in India may be studied under the following heads.

Introduction of Universalistic Legal system
Impact of English Education
Impact of Social Reform Movement
Influence of New Social Formation
Impact of Freedom Struggle
Impact of Industrialization and Urbanization

The Caste Disabilities Removal Act of 1850 This act served to remove some of the disabilities associated with castes including the practice of untouchability.
The Hindu Widow Remarriage Act 1856, This act made legal provision for the Hindu widows to remarry.
The Special Marriage Act of 1872 which considered marriage as a civil contract and legalized inter-caste or inter-religious marriage.
Other Legislative and Administrative Measures.

In 185 8 British government announced that all schools maintained by the government shall be open to all the classes of its subjects without any discrimination. In 1923, the government issued a resolution that no grants would be paid to any aided educational institutions, which refused admission to the children of the depressed classes. In 1925, a bill was passed by the Madras legislative council, throwing open all public office, well, tank, or place of public resort, to all classes of people including the depressed. Montogue-Chelmshford reforms made constitutional provisions for the special representation of depressed classes in the local as well as in the legislative bodies.

2. Impact of English Education: British education was based on scientific, secular and universal principles. It made an accessible to everyone, irrespective of caste or community, who could pay for it. It remained liberal in content. It propagated principles such as the liberty equality and fraternity. As education spread to the lower strata, it kindled libertarian impulses among them. Western education provided an indispensable passport to the new economic opportunities. Members from the lower castes became different professionals and took new commercial opportunities offered by the western education.

The Brahma Samaj founded by Raja Ram Mohan Roy
Prarthana Samaja’s Athma Rama Pandurang Justice M. Ranade
The Arya Samaja founded by Swamy Dayananda Saraswathi
The Ramakrishna Mission represents the synthesis of the ancient and the modern thoughts. Swamy Vivekananda started Ramakrishna Mission.
Other Reform Movements such as Annie Beasant’s Theosophical society, Maharshi Arabindo Ghosh’s Divine life Society and etc. Thus all these organizations aimed at the destruction of caste system and social re-construction of Indian society.

4. Impact of New Social Formations: The new economic system brought about a new grouping of the population in the economic sphere. The Indians could be differentiated into such categories as capitalists, workers, peasants, propritiators, merchants, tenants, land lords, doctors, lawyers, teachers and techniciAnswer: Each category being composed of individuals belonging to various castes, but having identical material and political interests. This division weakened the vertical caste lines.

Question 15.
Explain the functional changes and changer in the role of caste system in post – Independent India.
Answer:
Changes in caste system in post independent period can be discussed under two headings, viz., Functional Changes in Caste System and Changes in the Role of Caste System.

(A) Functional Changes in the Caste System: The caste system has neither disintegrated of people of all castes and left little room for the necessities of ceremonial purity. Taboos on food and water gradually weakening when industrial workers belonging to various castes started working under one roof. The demarcation observed by the members of different castes regarding eating food, physical contact with those of other castes, steadily crumbled in cities. 66. Explain the functional changes and changer in the role of caste system in post – Independent India.

nor did it disappear in present India despite many modifications. During the last six decades caste structures has considerably changed. Though, these two important features of hereditary membership and hierarchy have not changed at all. But we do find some changes in the following:

Occupational choice is relaxed
Decline and Disappearance of the caste panchayaths
Commensall restrictions are relaxed
The religious basis of caste has ramped. Caste is no more believed to be divinely
Caste is no longer restricts newly valued individual freedom and the occupational career of an individual, though his social status continue to be dependent on his caste membership.

(B) The Changes in the Role of Caste System: The major changes in the role of Caste system are following:

(1) Elections Based On Caste System Caste in modem India is very important, and every political party is aware of its vote catching power though the political leaders condemn caste verbally. But in practice caste consideration are potent.

(2) Increase of Caste Consciousness and Organizations: Caste consciousness and organization have increased in modem India. Caste based Educational Institutions, Banks, Hostels, Cooperative Societies, Charities, Marriage halls and journals, which are the indicators of caste consciousness. The journals, published by the caste organization, are the units of the media of communicative integration. The community aspect of caste has been made more comprehensive and permanent. According to G. S. Ghurye “Thus a vicious circle has been created. The feeling of caste solidarity is now so strong that it is truly described as caste patriotism”.

(3) Impact of Modern Means of Transport and Communication: According to M.N Srinivas “The building of Roads all over India, and the introduction ofRailway, Postal service, cheap paper and printing especially in regional languages enabled castes to organize as they had never done before. A post card carried news of a caste meeting and the railways enabled members sheltered in far-flung villages to come together when necessary, with the availability of cheap news print facilitated, the founding of caste journals, whose aim was to promote the interests of their respective castes.

(4) Impact of Modern Education: Education has been liberalized in post independence era. No doubt, modem educated youths being inspired by the ideals of equality, liberty, fraternity, scientific outlook, secularism, etc. have changed their attitudes towards caste system. It did not necessarily mean that caste has disappeared. The educated leaders started caste journals and held conferences. Funds were collected to organize the caste meetings and to help the poorer members. In general, it may be said that the last hundred years have seen a great increase in caste solidarity and the concomitant decrease of a sense of interdependence between different castes.

Question 16.
Define scheduled castes and explain the problem of scheduled castes.
Answer:
The description of scheduled castes as a marginalized group with a series of disabilities that are imposed on them. It is instead a catalogue typically associated with untouchability. These are as follows:

I. Social Disabilities.

Denial or restriction of access to public facilities, such as wells, schools and roads.
Restrictions on movement were also imposed. Untouchables might not be allowed to walk on roads and streets within prescribed distance of the houses or persons of higher castes.

II. Economic Disabilities.

Exclusion from any honourable and most profitable employment and fixity to dirty or menial occupations.
Restrictions on style of life, especially in the use of goods indicating comfort or luxury. Riding on horseback, use of bicycles, the wearing of gold and silver ornaments, all of these were forbidden in many areas.
Liability to unremunerated labour for the higher castes and to the performance of menial services for them.

III. Religious Disabilities.

In Indian untouchables were subjected to various religious disabilities. They were prevented from entering temples, Monasteries and cremation grounds and could not make use of them because it was believed that these places would become impure by their touch and presence. The untouchable could not worship in the temples. Their presence was considered sufficient to defile the God. They were not allowed to read and listen to the Holy Scriptures.

D.N. Majumdar summarized the position of the untouchable castes by maintaining that these castes are not depressed in all states, the same caste may be depressed in one area but may not suffer from any social and political disability in another. The disabilities are rigid where the depressed castes are numerically small, and fewer or on the decline where they numerically strong.

Question 17.
Define Tribe and explain the changing concept of Tribe.
Answer:
(a) Tribe as Homogeneous, Self-contained Unit: The Tribals are believed to be the original inhabitants of Indian Peninsula. They are generally called ‘adivasis’ which means original inhabitants. Vedic literature mentions various Tribes like the Bharathas, the Bhils, the Kolias, the Kirathas, the Kinnaras, the Matsyas, and the Nishadas. A Tribe was a homogeneous a self-contained unit without any hierarchical discrimination.

(b) Tribe as a Political Division: The concept Tribe derives its origin from the Latin term ‘Tribuz’ means three division. For Romans, the Tribe was a political division. The Tribe was the highest political unit comprising several districts which in turn were composed of clAnswer: It was a territorial organization exercising, control over its people. The territoiy under the domain of a particular Tribe was generally named after it. Thus, it is presumed that the name Bharath is derived from the mighty Bharatha Tribe.

Similarly, the matsya kingdom of 6th B. C. The Minas of Rajasthan and Madhya Pradesh are believed to be the descendants of the matsya Tribe. Mizoram, Nagaland and Tripura are named after the Mizo, the Naga and the Tripuri Tribes. Similarly, Santhal Paraganas, Gondawana, Lahaul and Kinnaur derive their names from the Santhalas, the Gondas, the Lahaulas and the kinnaras.

Tribal people of the Sub-Himalayan region belong to the Mongoloid race. They are sub-divided into Palaeo-Mongoloid represented by Tribes living in Assam, Meghalaya, Mizoram, Nagaland and Manipur and the Tibeto-Mangoloid represented by the Tribals living the Sikkim, Arunachal Pradesh and Ladak (Jammu & Kashmir). A major portion of the Tribal population in India belongs to the Mediterranean stock. They are generally known as the ‘Dravidians’. Tribes belonging to the Dravidian race are found both in southern parts and central parts of India. Dravidians are known as the original inhabitants of India. They speak Kannada, Tamil, Telugu and Malayalam or corrupt form of these languages.

Mahatma Gandhiji called Tribals as Girijana and G.S. Ghurye consider tribals as Backward Hindus. The concept of a Tribe has undergone further changes particularly after India’s independence. Dr. B.R. Ambedkar preferred the concept “Scheduled Tribe” to ‘Adivasis’. Under the Constitution of India certain Tribes have been specified as Scheduled Tribes. The constitution neither defined nor lays down any criteria for specifying the scheduled Tribes. Here Scheduled simply means ‘grouped together’. Tribes so grouped are given special treatment of facilities envisaged under the Constitution.

Question 18.
Give a Brief overview of – Backward class commissions of Karnataka.
Answer:
The backward class movement in Karnataka is a desire of the under-privileged people to develop their own potentialities and contribute to the economic development of the nation. In every society some groups of people are higher and some are lower due to the opportunities they have in general. By such opportunities well-off people equip themselves and pursue careers which give them prestige and profit. By contrast, the lower or other backward classes have no opportunities to equip themselves.

Anew awareness arose among the non-Brahmins in the princely state of Mysore. Vokkaligas, Lingayats and Muslims of Mysore had realized their position of relative deprivation as against the Brahmins. By 1917, these groups form an alliance called Prajamitra Mandali in 1918, this mandali pleaded Maharaja of Mysore for the representation in legislature, reservation in posts of public services and educational institutions. In 1918, a committee of six non-official members presided over by Sir Leslie Miller. Miller committee recommended the acceptance of all the demands. Since then Backward Classes in princely Mysore state have availed benefits in the field of education, employment and political arena.

(A) Naganna Gowda Commission: The Karnataka Government appointed a backward class commission in 1960 under the Chairmanship of Dr. Naganna Gowda. It is the First Backward Class Commission in Karnataka. The commission has submitted its report on 1961, which recommends 15% for SCs, 3% for STs and 50% OBCs, providing total 68% of reservation. The government attempted to implement the report was stayed by the Supreme Court. However, in 1963 the government issued an order guaranteeing 15% of reservation to SCs, 3% STs and 30% to OBCs.

(B) L. G Havanoor Commission: In 1972 the government has appointed the second backward class commission headed by Sri L. G. Havanoor. This commission in its report submitted in 1975 stated that though more than 75% of the people in the state belonged to backward classes and deserved reservation facilities. There was no constitutional provision for giving it. Hence, it made provision for up to 50% reservation. The government made provision for 58% reservation. However it was challenged in Supreme Court and govt, gave a submission to court stating to initiate a new commission.

(C) Venkataswamy Commission: In 1983, the government has appointed the Venkataswamy Commission, which gave its report in 1986. The report created wide spread dissatisfaction. The government decided not to implement the report but to establish a new commission to find an amicable settlement to this problem.

Based on the Mandal commission’s report, the supreme court of India gave directions to establish a permanent Backward Classes Commission in the centre as well as in states and union territories. Accordingly, a permanent backward classes commission was set up in Karnataka Sri K. Narayana Rai (1994-1997), Prof, Ravi Verma Kumar (1997-2000), Sri Muniraju (2001-2003), Sri Siddalingaih (2003-2006), Dr. C. S. Dwarakanath (2007-2010)N.

Shankarappa(2011 -13) headed the Backward Classes Commission in Karnataka. At present H. Kantharaj is the chairman of Karnataka state Back word class commission. The commission recommends for inclusion or exclusion of a caste in the backward class list. In Karnataka 101 and 51 Triber are enlisted as scheduled caster and scheduled Tribes Respectively.

2nd PUC Sociology Question Bank Chapter 1 Making of Indian Society and Demography

November 2, 2023

Karnataka 2nd PUC Sociology Question Bank Chapter 1 Making of Indian Society and Demography

You can Download Chapter 1 Making of Indian Society and Demography Questions and Answers, Notes, 2nd PUC Sociology Question Bank with Answers Karnataka State Board Solutions help you to revise complete Syllabus and score more marks in your examinations.

2nd PUC Sociology Making of Indian Society and Demography One Mark Questions and Answers

Question 1.
How is the term Demography derived?
Answer:
The term Demography is derived from two Greek words i.e. demos (people) and graphein (describe), implying the description of people.

Question 2.
What is Demography?
Answer:
Demography is the systematic study of population.

Question 3.
Name any one type of Demography.
Answer:
Formal Demography.

Question 4.
Give one major characteristic of Demographic profile of India.
Answer:
Size and Growth of India’s Population.

Question 5.
Mention sex ratio of India according to 2011 census.
Answer:
940.

Question 6.
Which district in Karnataka is Selected to Implement Beti Padavo and Beti Bachavo Programme?
Answer:
Vijyaypura District.

Question 7.
Name one racial group of India.
Answer:
Negritos.

Question 8.
Give one sub division of Mongoloid race in India;
Answer:
Paleo Mangoloid.

Question 9.
Who introduced Christianity to India?
Answer:
St Thomas and St Bharathaomew.

Question 10.
What is unity?
Answer:
Unity implies oneness, or a sense of we-ness.

Question 11.
Name one basis of diversity in India.
Answer:
Linguistic Diversity.

Question 12.
Name one basis of unity of India.
Answer: Religious unity.

Question 13.
What is National Integration?
Answer:
National integration refers to national unity and a sense of belonging to the nation.

Question 14.
Name anyone challenge to National Integration.
Answer:
Communalism.

Question 15.
Name any one religious community of India.
Answer: Hindus.

Question 16.
Name any one ancient name of India.
Answer:
Bharathakanda.

Question 17.
What does DEMARU stands for?
Answer:
Data from 2001 census to coin another acronym – DEMARU – where D stands for daughter and MARU stands for killing. In English “E” will denote elimination. Punjab, Hariyana, Himachal Pradesh & Gujarath, Maharashtra as DEMARU states, where the sharp decline in the Juvenile sex ratio.

Question 18.
Which European colonial group first entered India?
Answer:
Portuguese.

Question 19.
Expand the UNPF
Answer:
United Nations Population Fund.

Question 20.
Expand the Abrivation BIMARU
Answer:
Bihar, Madhya Pradesh, Rajasthan and Uttar Pradesh.

Question 21.
Define formal Demography.
Answer:
Formal demography is primarily concerned with the measurement and analysis of the components of population change. Its focus is on quantitative analysis for which it has a highly developed mathematical methodology suitable for forecasting population growth and changes in the composition of population.

Question 22.
Define social Demography.
Answer:
Social demography, on the other hand, enquires into the wider causes and consequences of population structures and change. Social demographers believe that social processes and structures regulate demographic processes; like sociologists, they seek to trace the social reasons that account for population trends.

Question 23.
In which year first census was conducted.
Answer:
1867-72.

Question 24.
Which is the oldest civilization of India.
Answer:
Indus valley civilization.

Question 25.
In which was year Kannada language has declared as one of the classical languages.
Answer:
2008.

Question 26.
What is the total population of Karnataka according to 2011 census?
Answer:
6,10,95,297.

Question 27.
What is the sex ratio of Karnataka according to 2011 census?
Answer:
973.

Question 28.
Which is the most populated district in Karnataka.
Answer:
Bangalore District.

Question 29.
Which district has Highest Sex Ratio in Karnataka? ‘
Answer:
Udupi District (1094).

Question 30.
Which district has Lowest Sex Ratio in Karnataka?
Answer:
Bangalore District (916).

Question 31.
What is the density of Karnataka according to 2011 census?
Answer:
319.

Question 32.
Which district in Karnataka registers Highest density?
Answer:
Bangalore District (2985).

Question 33.
Which district in Karnataka registers Lowest density?
Answer:
Kodagu District (134).

Question 34.
Which district has recorded highest SC population in Karnataka?
Answer:
Kolar District.

Question 35.
Which district has recorded highest ST population in Karnataka?
Answer:
Raichur District.

Question 36.
Which district in Karnataka has retained highest literacy rate?
Answer:
Dhakshina Kannada (88.57).

Question 37.
Which district in Karnataka has retained lowest literacy rate?
Answer:
Yadagiri(51.83).

Question 38.
What is the total literacy rate of Karnataka in 2011?
Answer:
75.39%.

Question 39.
What is Sex Ratio?
Answer:
Number of females for 100 male population.

Question 40.
State a Reason for Imbalance in Sex Ratio.
Answer:
Sex selective abortion.

Question 41.
Which year is considered as demographic divide?
Answer:
1921.

Question 42.
How many languages were recognized by Indian constitution as official languages?
Answer:
22.

Question 43.
Mention any one Indo-Aryan language
Answer:
Hindu.

Question 44.
Mention any one Dravidian Language.
Answer:
Kannada

Question 45.
Mention any one Austric Language
Answer:
Mundari.

Question 46.
In which year Government of India declared Kannada as one of the classical language?
Answer:
2008.

Question 47.
Which is the oldest water dispute in the world?
Answer:
Cauvery water dispute.

2nd PUC Sociology Making of Indian Society and Demography Two Marks Questions and Answers

Question 1.
Define Demography.
Answer:
Demography studies the trends and processes associated with population including – changes in population size; patterns of births, deaths, and migration; and the structure and composition of the population, such as the relative proportions of women, men and different age groups.

Question 2.
Give two major characteristics of Indian Demographic profile.
Answer:

Size and Growth of Population.
The declining sex ratio.

Question 3.
Mention any two factors responsible for decline of child sex ratio.
Answer:
Sex selective Abortions, Neglect of Girls in an Infancy.

Question 4.
Mention any two racial groups of India.
Answer:
Negritos and Mongoloids

Question 5.
Name two difficulties to the process of Aryanzation.
Answer:

Tribal groups refused to be absorbed.
Special problem posed by strong ethnic groups.

Question 6.
What does DEMARU stands for?
Answer:
Data from 2001 census to coin another acronym – DEMARU – where D stands for daughter and MARU stands for killing. In English “E” will denote elimination. Punjab, Hariyana, Himachal Pradesh & Gujarath, Maharashtra as DEMARU states, where the sharp decline in the Juvenile sex ratio.

Question 7.
Define national Integration.
Answer:
National integration refers to national unity and a sense of belonging to the nation.

Question 8.
What is Regionalism?
Answer: Regionalism refers to an extreme loyalty or love to a particular region which may undermine the interest of the nation.

Question 9.
What is communalism?
Answer:
Communalism is the antagonism practiced by the members of one community against the people of community and religion.

Question 10.
What is Iinguism?
Answer:
Linguism implies one-sided love and admiration towards one’s language and a prejudice and hatred towards others’ languages.

Question 11.
Give two measures to strengthen national integration.
Answer:

Reorganization of syllabus
conductiong community programmes.

Question 12.
State any two dravidian language.
Answer:
Tamil and Kannada.

Question 13.
State any two Indo – Aryan languages.
Answer:
Sanskrit and Hindu.

Question 14.
State any two Austric languages.
Answer:
Mundari and Santhali.

Question 15.
State any two Tibeto – Burman languages.
Answer:
Bodo and Ladaki.

Question 16.
Mention any two classical languages of India.
Answer:
Sanskrit and Tamil.

Question 17.
Mention any two threats to national Integration.
Answer:
Communalism and Regionalism.

Question 18.
State any two forms of regionalism.
Answer:

Demond for a separate Nation from India.
Demond for a separate State within India

Question 19.
Why India is regarded as museum of languages?
Answer:
India is a land of many languages and it has been called as a ‘museum of languages’.

Question 20.
What is demographic Dividend?
Answer:
Demographic dividend refers to demographic or population advantage which is obtained due to numerical domination of the young people in the population. It is an advantage due to less dependency ratio.

Question 21.
What is dependency ratio?
Answer:
Dependency ratio means children less than 14 years and people above 65 years are considered as to be dependent on the rest of the population. In simple terms the ratio of the combined age group 0-14 years plus 65 years & above to the 15-65 years age group is referred to as the total dependency ratio.

2nd PUC Sociology Making of Indian Society and Demography Five Marks Questions and Answers

Question 1.
Explain the racial groups classified by B.S. Guha.
Answer:
B.S. Guha who identified six major racial elements in the population of India:

Negrito
Proto-Australoid
Mongoloid
Mediterranean
Western Brachycephals .
Nordic

In the south, the Kadar, the Irula, and the Paniyan, and in the Andaman Islands, the Onge and Jarwas of the Andamanese have definite Negrito characteristics. Some traits of this group are found among the Angami Naga and the Bagadi of the Rajmahal hills. On the western coast there are some groups with pronounced Negrito traits, but they perhaps represent later arrivals, who came to India with the Arab traders.

The Proto-Australoid group is numerically more significant; most of the tribes of middle India belong to it. These were the people described by the Indo-Aryans as Anas, Dasa, Dasyu, and Nishad – all derogatory terms. The Mongoloid group is sub-divided into two branches – Paleo- Mongoloid and Tibeto-Mongoloid. Tribal groups in the Himalayan region and those in the north-east are of Mongoloid stock. Some Mongoloid features are seen in the non-tribal population of the eastern States – Assam, West Bengal, Manipur, and Tripura.

The Western Brachycephals (sub-divided into the Alpinoid, Dinaric, and Armenoid groups), Alpinoid and Dinaric characteristics are seen in some groups of northern and western India; the Parsis belong to the Armenoid section. The Mediterraneans are associated with the Dravidian languages and cultures. The Nordics were the last major ethnic element to arrive in India and make a profound impact on its culture and society. But before they came a unique civilization had slowly developed in India. It is known as the Indus Valley Civilization.

Question 2.
Write note on the processes of Aryanization.
Answer:
They were essentially a pastoral people with a flair for poetry, philosophical speculation, and elaborate rituals. They regarded themselves as superior and tended to look down upon the earlier inhabitants of the land, for whom they coined several derogatory terms. They were required to marry within their own group, i.e., practise endogamy, and had some elementary notions of ritual purity and pollution which governed their physical contacts and commensal – inter-dining – relations with others. This led to the origin of the Vamas and also of Jati (caste). Commensality and sexual relations within different tribal groups and earlier ethnic groups were governed by customary norms and taboos, and the advent of the Indo-Aryans brought in refinements and complexities in them.

The Indo-Aryans were divided into three groups – the Rajanya (warriors and the aristocracy), the Brahmana (priests), and the Vaishya (cultivators). These were Dvija (twice-born) groups

born first at physical birth and a second time when initiated into Varna status. The Shudras were the fourth Vama; they were from outside the Indo-Aryan group and were perhaps the progeny of unions between the Indo-Aryan and the Dasa (the pre-Aryan .inhabitants of the land). They emerged as cultivators, but were denied twice – bom status. Outside the four-fold vertical Vama structure, there was a fifth group – Avarna or the Pancham – whose ethnic status was so low and their occupations so degraded and polluting that any physical contact with them was prohibited for the twice-born and the Shudra.

The process of Aryanization of the sub-continental traditions was neither smooth nor complete. Its earlier phase was characterized by considerable cultural conflict and warfare. Much Accommodation and Compromise were also taking place.

This necessitated greater harmony with the older inhabitants; in any case, some kind of synthesis between them was already taking place. As a result the non-Aryans adopted some elements of Indo-Aryan ritual and their philosophy of social organization, while retaining their own ethnic and regional identities. Pluralism was being stabilized and a cultural mosaic being formed.

Question 3.
Explain the nature of diversities in India.
Answer:
The term Diversity denoting collective differences so as to find out dissimilarities among the people: geographical, religious, linguistic etc. Thus all these differences presuppose collective differences or prevalence of variety of groups and culture. Indian society is characterized by unity as well as diversity.
The Nature of Diversities in India
Primarily there are major four types of diversities in India, which are;

Regional Diversities
Linguistic Diversities
Religious Diversities
Cultural and Ethnic Diversities

(1) Regional Diversities: It is evident that there are extreme regional diversities in its geographic features. Indian territory contains huge mountains, thick forests, numerous rivers and etc. It is only in India that there are different regions, different types of climates and the temperatures. India is a vast country. From the Himalayas in the North to Indian Ocean in the south. There are difference in altitude, temperature, Flora and Fauna. India has every conceivable type of climate, temperature and physical configuration. There is the scorching heat of Rajastan and the biting cold of the Himalayas, Rainfall varies from 1200 to 7.5 cms per year. The result is that India has some of the wettest and driest areas in the world. India also possesses arid desserts and fertile riverine lands, bare and hilly tracts and luxuriant open plain.

spoken in the country. According to Grierson’s Linguistic Survey of India, these languages belong to five linguistic families, namely; Indo-Aryan languages, Dravidian languages, Austric languages, Tibeto – Burman languages and European languages.

The Indo-Aryan languages including in the Sanskrit, Hindi, Bengali, Marati, Gujarathi, Oriya, Panjabi, Bihari, Rajasthani, Assami, Sindhi and Kashmiri languages spoken by 3/4th of Indian population.
The Dravidian languages include Tamil, Kannada, Telugu and Malayalam.
The Austric languages include Mundari, Santhali, Maithili, Dogri and etc.
Tibeto – Burman languages: Tribal languages and dialects of North and North-East India belong to this category. For example Manipuri, Bodo, Ladiki, Khaki, etc.
The European languages include English, Portuguese and French. These last two languages are spoken mostly people in Goa and Pondicherry.

This makes language planning and promotion difficult. But the mother tongue does evoke strong sentiments and reactions. As a consequence of this multiplicity, there is considerable bilingualism and administration has to use more than one language. Linguistic diversity has posed administrative and political challenges.

(3) Religious Diversities: There are eight major religious communities in India. Hindus constitute 82.7%, Muslims 11.8%, Christians 2.6%, Sikhs 2%, Buddhists 0.7%, Jains 0. 4%, Zoroastrians 0.3%, and Jews 0.1 %. Each major religion is subdivided along the lines of religious documents, sects, and cults. The Hindus are now broadly divided into Shaivite (worshippers of Shiva), Vaishnavaite (worshippers of Vishnu and his incarnations), Shakta (worshippers of the Mother Goddess in various manifestations. Even among them there are sub – divisions based on doctrinal and ritual differences.

Buddhism was spread widely in India once, it lost its hold in the country of its birth and remained confined only to a few pockets. Jainism too, once held wide sway in India, and though its followers are now numerically small, they are found in both the northern and southern States. They have two main divisions: Digamber-unclothed, and Shwetamber. The Indian Muslims are divided broadly into the Sunni and Shia communities.

Indian Christians are divided into Roman Catholics and Protestants and into many denominational churches. Sikhism is synthesizing religion that emphasizes egalitarianism. Grantha Sahib is the holy book and Amritsar Golden Temple is one of their holy piligrimage. The Parsis are a small community, but they have played an important role in India’s industrial development. The Jewish has been established in India for over a millennium. They also had White and Black divisions and prohibition on inter-marriage and inter-dining, but they all worshipped in the same synagogues. The Jati-like restrictions are much less in Bombay and Cochin, to where many Jews have migrated.

(4) Cultural and Ethnic Diversities: Another important source of diversity is the cultural diversity. The people differ considerably in their social habits. Cultural difference varies from state to state. The conflicting and varying shades of blood, strains, culture, and modes of life, the character, conduct, beliefs, morals, food, dress, manners, social norms, Socio-Religious customs, rituals and etc. causes cultural and ethnic diversities in the country. Dr. R.K. Mukherji rightly said that “India is a museum of cults and customs, creeds and culture, faiths and tongues, racial types and social systems”. Another important source of diversity is the cultural identity of particular communities and region.

Question 4.
Explain the unity in India.
Answer:
In India aspects of Diversity is as follows as :

(1) Regional Unity: The Natural boundaries provide India a. geographical unity. In ancient times India was known as Bharatavarsha, Bharathakanda, Jambudweepa. This symbolizes the significance of historical unity. The very name “Bharatavarsha” has occupied an important place in the minds of poets, political philosophers, and religious thinkers. Each of them has conceived of the country as a single expanse from the Himalayas to Kanyakumari, a country ruled by one king Bharatha. The concept of Mother India also indicates the realization of geographical unity.

(2) Linguistic Unity: Despite the presence of number of languages, India also possesses lingual unity. Sanskrit as a common base of Indian languages provides the basis of unity as a result of which the linguistic multiplicity has been solved. Simultaneously Sanskrit became the language of Hindu culture and all classics were composed in this language, which demanded reverence and respect. People may speak different languages in different regions but they have common language of Engl ish and Hindi to communicate with each other. The formation of linguistic states and using regional languages as medium of teaching at schools, colleges and universities are the products of Independence.

In 2004 the govt, of India declared that languages that met certain requirements could be accorded the status of a classical language in India. Tamil (2004), Sanskrit (2005), Kannada (2008), Telugu (2008), Malayalam (2013) and Oriya (2014) are declared as classical languages of India. Thus it is an effort to restore linguistic heritage of India.

(3) Religious Unity: In spite of the religious diversities, it possesses religious unity. The feelings of each religious groups are the same, each accepts the truth of immortality of > soul, temporary nature of world, belief in rebirth, the doctrine of karma, Salvation, Contemplation etc., There may be differences in the way these elements are treated but each religion preaches a fundamentally single religious faith and shares a belief in purity ; and values of life in respect of belief in unseen power, benevolence, piety, honesty and liberality, with every religious faith. The worshippers may visit different centres of pilgrimage, but all have a common goal of “Earning religious merit by visiting a sacred place”. India is the sacred land not only for the Hindus but also for Sikhs, Jains and Buddhists. The Muslims and Christians too have several sacred centres of pilgrimage in India.

(4) Cultural Unity: In art and architecture, dress and food, literature, music and dance, sports and cinema, medicine and technology there was a fusion of style and the emergence

of new forms which were the result of their combined efforts. Thus it became apparently clear from the above account that running through various diversities. India has been helped both by nature and nurture, by her geographical condition and historical experiences, by her religious ethics, and political ideas. To realize a unity to perceive, preserve and strengthen the thread of basic unity which makes India a fine example of unity in diversity, transcending birth, caste, language, ethnicity and religious groupings to establish a big society and a big nation.
Modem education, the development of a network of transport and communications, industrialization and urbanization provided new bases for unity.

Question 5.
Discuss briefly the challenges to National Integration.
Answer:
There are many challenges to national integration. They are as follows:

Regionalism
Communalism
Linguism
Extremism and Terrorism

(1) Regionalism: Regionalism is expressed in the desire of people of one region to promote their own regional interest at the expense of the interests of other regions. It has often led to separatism and instigated separatist activities and violent movements. It has also gained tremendous momentum during the recent years because it is exploited by the selfish politicians Thus, regionalism has challenged the primacy of the nationalistic interests and undermines national unity. Regionalism assumed mainly four forms.

(2) Communalism: Communalism is the antagonism practiced by the members of one community against the people of community and religion. Bipin Chandra holds that communalism is the product of a particular society-, economy and polity, which creates problems. Asghar Ali Engineer, Moin Shakir and Abdul Ahmed try to explain communalism as an ideological tool for propagation of economic and political interests. According to them, it is an instrument in the hands of the upper class to concentrate power by dividing people. The elites strive to maintain a status quo against transformation by dividing people on communal and religious lines.

(3) Linguism: Linguism implies one-sided love and admiration towards one’s language and a prejudice and hatred towards others’ languages. India is a land of many languages and it has been called as a ‘museum of languages’. Diversity of languages has also led to linguism. It has often been manifested into violent movements posing threat to national integration. Linguistic tensions are prevailing in the border areas which are bilingual.

(4) Extremism and Terrorism: Extremism and terrorism have emerged during the recent years as the most formidable challenges to national integration. Extremism refers to the readiness on the part of an individual or group to go to any extreme even to resort to undemocratic, violent and harmful means to fulfill one’s objectives. In the past India has been facing the problems of terrorism since independence. India has faced this problem in Nagaland (1951), Mizoram (1966), Manipur (1976), Tripura (1980) and West Bengal in (1986).

Terrorism in India is essentially the creation of politics. According to According to Prof. Rama Ahuja there are four types of terrorism India, (1) Khalistan oriented terrorism in Puniab (2) Militants terrorism in Kashmir. (3) Naxalite terrorism in west Bengal, Bihar, Madhya Pradesh, Orissa, Andhra Pradesh Telangana, Maharastra, Uttarapradesh Jharkhand, chattisghad out of 318 district 77 districts are highly Naxal poore districts causing lot of bloodshed in these areas. (4) ULFA terrorism in Assam.

The Khalistan oriented Sikh terrorism was based on a dream of theocratic state, Kashmir militants are based on their separate identity. The Naxalite terrorism is based on class enmity. Terrorism in North Eastern India is based on the identity crisis and the grievance situation. In addition to these factors, corruption, poverty, unemployment/youth unrest, widening gap between rich and poor, which are also the major challenges for national integration.

Question 6.
Briefly explain the Dr. Sampurnananda committee recommendations to strengthen National Integration.
Answer:
The Central Education Ministry organized a ‘Committee for National Integration’ in 1961 under the Chairmanship of Dr. Sampurnanand. The Integration Committee gave some recommendations to promote and strengthen national integration. Some of them are stated below:

Re-organization of the syllabi at various levels – primary, secondary, college and university level- to promote national integration.
Giving due encouragement to extra-curricular activities besides imparting formal knowledge to the students with the intention of promoting national unity.
Improvement of textbooks helps a great deal in giving a true national perspective to the students. They can be made to understand their rich cultural heritage and feel proud of their nation.
Conducting community programmes such as mass prayers, mass meetings, speeches by respected leaders, etc., to help to bring the people together.

Apart from the governmental efforts to achieve the goal of national unity various stakeholders such as educational institutions, religious/cultural associations and mass media should involve in chalking out action-based programmes to enhance awareness/dissemination of traditional values among the masses and increase cultural exchange banking on the richness of our cultural heritage and diversity. Special steps should be taken by various interest groups to speed up development of economically and socially backward groups who are the easy victims of violent activities.

Question 7.
Write a note on BIMARU v/s DEMARU.
Answer:
The results of the 2001 census fully validate the diagnosis of India’s population problem in terms of the dominance of BIMARU States (Bihar, MP, Rajastan, UP) accounted for 39% of India’s population, 42% of growth and 48% of the total Illiterate population and the adverse role of these BIMARU states. To take note of the alarming data from 2001 census to coin another acronym – DEMARU – where D stands for daughter and MARU stands for killing. In English “E” will denote elimination. Punjab, Hariyana, Himachal pradesh & Gujarath, Maharashtra as DEMARU states, where the sharp decline in the Juvenile sex ratio.

These states are in the perverse practice of Foeticide. The unholy alliance between Tradition (Son complex) and Technology (Ultra sound etc.) is playing havoc with Indian society pre-birth sex determination tests & sex selective abortion are rampant in these states. During the last decade in Punjab, the sex ratio (0-6 age group) declined from 875 to 793. In Hariyana the sex ratio (0-6 age group) decreased from 879 to 820.

In Maharashtra every single district showed a decline in the Juvenile sex ratio between 1991-2001. In Himachal pradesh from 951 to 845. In Gujarath from 928 to 878. In Chandigarh from 899 to 845 and in Delhi from 915 to 865. Even though an increase in the sex ratio for the total population was 927 in 1991 to 933 to 2001. In contrast the sex ratio of child population (0-6 age group) which was 945 in 1991 decreased to 927 in 2001. In short the girl child (below 6 years) has lost out badly.

The state-level child sex ratios offer even greater cause for worry. As many as six states and union territories have a child sex ratio of under 900 females per 1000 males. Punjab is the worst off with an incredibly low child sex ratio of 793 (the only state below 800), followed by Haryana, Chandigarh, Delhi, Gujarat and Himachal Pradesh. Uttaranchal, Rajasthan, Uttar Pradesh and Maharashtra are all under 925, while Madhya Pradesh, Goa, Jammu and Kashmir, Bihar, Tamil Nadu, Karnataka and Orissa are above the national average of 927 but below the 950 mark. Even Kerala, the state with the best overall sex ratio does not do too well at 963, while the highest child sex ratio of986 is found in Sikkim.

Question 8.
What is Demographic Dividend? How it can be utilized?
Answer:
Demographic dividend refers to demographic or population advantage which is obtained due to numerical domination of the young people in the population. It is an advantage due to less dependency ratio. Dependency ratio means children less than 14 years and people above 65 years are considered as to be dependent on the rest of the population. In simple terms the ratio of the combined age group 0-14 years plus 65 years & above to the 15-65 years age group is referred to as the total dependency ratio.

The younger age groups in the age structure is believed to be an advantage for India. Like the East Asian economies in the past decade and countries like Ireland today, India is supposed to be benefitting from a ‘demographic dividend’. This dividend arises from the fact that the current generation of working-age people is a relatively large one, and it has only a relatively small preceding generation of old people to support. But there is nothing automatic about this advantage – it needs to be consciously utilised in the following ways.

a. The demographic advantage or ‘dividend’ to be derived from the age structure of the population is due to the fact that India is one of the youngest countries in the world. In 2020, the average Indian will be only 29 years old, compared with an average age of 37 in China and the United States, 45 in Western Europe, and 48 in Japan. This implies a large and growing labour force, which can deliver unexpected benefits in terms of growth and prosperity.

b. But this potential can be converted into actual growth only if the rise in the working age group is accompanied by increasing levels of education and employment.

c. India is indeed facing a window of opportunity created by the demographic dividend. The effect of demographic trends on the dependency ratio defined in terms of age groups is quite visible. The total dependency ratio fell from 79 in 1970 to 64 in 2005. But the process is likely to extend well into this century with the age-based dependency ratio projected to fall to 48 in 2025 because of continued fall in the proportion of children and then rise to 50 by 2050 because of an increase in the proportion of the aged.

d. This suggests that the advantage offered by a young labour force is not being exploited. Unless a way forward is found, we may miss out on the potential benefits that the country’s changing age structure temporarily offers.
Discuss the Manifestation of Regionalism.

Regionalism assumed mainly four forms:

(i) The Demand for Separate Nation from the Indian Union: The first and most challenging form of Regionalism took was the demand of the people of certain state to succeed from the Indian union and became an independent sovereign states. The first such example was the Tamil community of the state of Madras. The campaign throughout Madras state for the separation of Madras from India and for making it an independent sovereign state of dravidastan. In a similar way Sikhs of Punjab demanded for a separate nation called Khalistan and liberation of Jammu and Kashmir form the Indian union, Insurgency and Secessionist movement in North-Eastern States (Assam, Nagaland, Tripura, Manipur, Meghalaya, Arunachal Pradesh and Mizoram) etc. demand for separate nation.

(ii) Demand for a Separate Statehood: A new form of regionalism has manifested in recent years was the demand for separate statehood in several states of Indian Union, like; Vidarbha states (Maharastra), Telangana, (Andhra Pradesh), Bundelkhand (M.P. and U.P.), Vindhya, Bhojpur and etc. In early 1980s the Jharkhand movement grow much more miltant and the various groups demanding the formation of separate state of Jharkhand, as well in Darjaling, district of West Bengal for Gorkha Land.

(iii) Demand for Full Fledged Statehood: Yet another manifestation of regionalism in India is demand of union territories for full fledged statehood, e.g. Delhi.

(iv) Inter – State Disputes: The first such dispute took place between Karnataka and Maharashtra, Punjab and Haryana. In addition to the above the main disputes are over the sharing of river waters. Over the water of Narmada, Krishna, Kaveri, Bheema and etc. Kaveri water dispute is the oldest water dispute in the world, causing animosity between Karnataka and Tamilnadu frequently.

2nd PUC Sociology Making of Indian Society and Demography Ten Marks Questions and Answers

Question 1.
Define Demography and Explain the major characteristics of Demographic profile of India.
Answer:
Demography is the systematic study of population. The term Demography is derived from two Greek words i.e. demos (people) and graphein (describe), implying the description of people. The term Demography was coined by Achille Guillard in 1855. Demography studies the trends and processes associated with population including – changes in population size; patterns of births, deaths, and migration; and the structure and composition of the population, such as the relative proportions of women, men and different age groups.

There are different varieties of demography, including Formal demography which is a largely quantitative field, and Social demography which focuses on the social, economic or political aspects of population. All demographic studies are based on processes of counting or enumeration – such as the census or the survey – which involve the systematic collection of data on the people residing within a specified territory.

The American census of 1790 was probably the first modern census, and the practice was soon taken up in Europe as well in the early 1800s. In India, census was conducted by the British Indian government between 1867-72, and regular ten yearly (decennial) censuses have been conducted since 1881. Independent India continued the practice, and seven decennial censuses have been conducted since 1951, the most recent being in 2011. Demographic data are important for the planning and implementation of state policies, especially those for economic development and general public welfare.

The Major Characteristics of the Demographic Profile of India:

Size and Growth of India’s Population
Age Structure of the Indian Population
Sex-Ratio in India
Birth Rate and Death Rate
Increasing Literacy Rate of Indian Population
Increasing Rural-Urban Differences

(1) Size and Growth of India’s Population: India is the second most populous country in the world after China. According to 2011 census India’s population is 121 crores (1.21 billion). Between 1901-1951 the average annual growth rate did not exceed 1.33%, a modest rate of growth. In fact between 1911 and 1921 there was a negative rate of growth of-0.03%. This was because of the influenza epidemic during 1918-19 : 5%of the total population of the country. The growth rate of population substantially increased after independence from British rule going up to 2.2% during 1961-1981. Since then although the annual growth rate has decreased it remains one of the highest in the developing world.

(2) Age Structure of the Indian Population: India has a very young population – that is, the majority of Indians tend to be young, compare to most other countries, the share of the less than 15 age group in the total population has come down from its highest level of 42% in 1971 to 29% in 2011. The share of the 15-60 age group has increased slightly from 53% to 63%, while the share of the 60+ age group is very small but it has begun to increase (from 5% to 8%) over the same period. But the age composition of the Indian population is expected to change significantly in the next two decades. 0-14 age group will reduce its share by about 11% (from 34% in 2001 to 23% in 2026) while the 60 plus age group will increase its share by about 5% (from 8% in 2001 to about 12% in 2026).

(3) The Declining Sex-Ratio in India: The sex ratio is an important indicator of gender balance in the population. The sex ratio is defined as the number of females per 1000 males. The trends of the last four decades have been particularly worrying – from 941 in 1961 the sex ratio had fallen to an all time low of 927 in 1991 before posting a modest increase in 2001.

According to the Census of India 2011 sex ratio has been increased and now it is 940 females per 1000 males. But what has really alarmed demographers, policy makers, social activists and concerned citizens is the drastic fall in the child sex ratio. The sex ratio for the 0 – 6 years age group (known as the juvenile or child sex ratio) has generally been substantially higher than the overall sex ratio for all age groups, but it has been falling very sharply. In fact the decade 1991-2001 represents an anomaly in that the overall sex ratio has posted its highest ever increase of 6 points from the all time low of 927 to 933, but the child sex ratio in 2011 census has dropped from 927 to 914, a plunge of 13 points taking it below the overall sex ratio for the first time.

(4) Increasing Literacy Rate of Indian Population:. Literacy varies considerably across gender, regions, and social groups. As can be seen from Table No. 4, the literacy rate for women is almost 22% less than the literacy rate for men. However, female literacy has been rising faster than male literacy, partly because it started from relatively low levels. Female literacy rose by about 11.2 percent between 2001 and 2011 compared to the rise in male literacy of 6.2 percent in the same period.

Female literacy was 8.9% in 1951 has increased to 65.4 in 2011 male literacy in the same period wan 27.2% has increased to 82.17. In 1951 total literacy rates 18.3% has increased . to 74.04 in 2011.

Question 2.
Explain the development of Christianity and Islam in India.
Answer:
To understand the texture of Indian society we have also to take note of the long presence of Christianity and Islam in the country. Both were influenced by the prevailing ethos and both made some impact on society. Christianity and Islam acquired some special characteristics in the Indian setting. St. Thomas and St. Bartholomew (A.D. 50) are believed to have brought Christianity to India in A.D. 50. When the Portuguese arrived in India, Christianity was found to have spread over seventeen kingdoms of Kerala. It is believed that Kalyan, near Bombay, emerged as a major centre of Christianity. Apart from St. Bartholomew, a specially invited Stoic philosopher – Pantaenus – was preaching at Kalyan.

The early Christians, however, were held in high esteem by the Hindus. Things began to change with the arrival and establishment of the Portuguese in India. When St. Francis Xavier landed in Goa in 1542, it had become a Christian settlement with fourteen churches and over a hundred clergymen. The Italian Jesuit, Roberto de Nobili, who landed in Goa in 1605 and died in Mylapore (Madras) in 1656, communicated with the people in Tamil and other regional dialects. His discussions with the Brahmans were in Sanskrit. De Nobili was keen to get convinced converts from the top of Hindu society to facilitate the rapid spread of Christianity. He avoided contact with Christians of lower caste origins, including their priests. By the end of the seventeenth century De Nobili and his associates had made many converts.

The later development of Christianity in India is better known because of the association of the Church with foreign powers – The Portuguese, Dutch, British, and French. Considerable evangelical and humanitarian work was done by Christian missionaries of diverse nationalities and denominations. Besides Kerala and Tamil Nadu, there are pockets of Christianity in most States of the Indian Union. At least three of the tribal north-eastern States have substantial Christian majorities. Christians have a sizeable presence among the tribals of Chotanagpur too.

Islam first came to India by peaceful methods, often with the encouragement of Hindu rulers. On the western coast, the Balhara dynasty in the north and the Zamorin of the Malabar coast welcomed Muslim traders and encouraged them to settle in places like Anhilwara, Calicut, and Quilon. They could freely build mosques and practice their religion. Arab and Persian immigrants settled down along the coast and married non – Muslim women. This is how the Nawait (Natia) community of Konkan and the Mappilla (Moplah) community of the Malabar coast emerged. The Labbais, on the east coast of Tamil Nadu, are said to have originated from the union of Tamil women with Arabs who were either shipwrecked or exiled from Iraq.

In the thirteenth and fourteenth centuries several missionaries were active in the Punjab, Kashmir, the Deccan, and eastern and western India. What needs to be emphasized is that these missionaries transmitted the message of Islam through love and without the support of the armed might of the State. The sword doubtless won converts but it also evoked hostility; the saints used persuasion and they still have a grateful and devoted following even among non-Muslims.

The socio-political conditions in India had changed by the time Ghazni invaded the country. Infighting between rival kingdoms had weakened Indian resistance. The Muslim rulers were in India not only for the spread of Islam, but had other interests also. Some of them took upon themselves the mission of Islamizing society more seriously than others; discriminatory practices such as the imposition of jizyah (poll tax), in addition to kharaj (tax on land and property), were pursued more vigorously by some rulers, while others were relaxed about them.

Despite long years of Muslim rule the overwhelming majority of the people remained Hindu. The army, state administration, and trade and commerce all depended on the direct and indirect support of the Hindus. Thus, in respect of the hated jizyah we find that it was levied sometimes and then abolished, only to be re-levied by another zealot. In fact, Islam was being Indianized; in the process, it acquired some distinctive characteristics in India. In the realms of art and architecture, philosophy and religion, medicine and other secular knowledge, there was considerable interchange. A composite culture was gradually evolving.

It may be added that Hindu rule was never completely wiped out from India. During the Delhi Sultanate, the Hindu kingdom of Vijayanagar was an impressive power in south. During Mughal times warriors like Rana Pratap refused to surrender; others worked out adjustments which left them considerable internal autonomy.

In Muslim society itself there was internal differentiation. For example, the difference between the Ashraf (those claiming descent from groups of foreign extraction) and Ajlaf (Converts from the lower Hindu castes) had a lower social position, many of the lower groups converted to Islam continued to occupy more or less their old position in society. Some Hindus occupied higher position in the royal courts and were even Generals in the army. The Hindu and Muslim aristocracy was closer. The poorer Muslims could only have the psychological satisfaction of belonging to the religion of the ruling class. But economically and socially they remained backward and exploited. The Muslim rulers of India understood the value of communal amity and realized the importance of inter community tolerance and understanding.

Question 3.
Define diversity and explain types of diversity in India.
Answer:
The term Diversity denoting collective differences so as to find out dissimilarities among the people: geographical, religious, linguistic etc. Thus all these differences presuppose collective differences or prevalence of variety of groups and culture. Indian society is characterized by unity as well as diversity.
The Nature of Diversities in India
Primarily there are major four types of diversities in India, which are;

Regional Diversities
Linguistic Diversities
Religious Diversities
Cultural and Ethnic Diversities

(2) Linguistic Diversities: Language is another source of diversity. It contributes to collective identities and even to conflicts. The Indian Constitution has recognized 22 languages in the 8th schedule for its official purposes but as many as 1652 languages and dialects are spoken in the country. According to Grierson’s Linguistic Survey of India, these languages belong to five linguistic families, namely; Indo-Aryan languages, Dravidian languages, Austric languages, Tibeto – Burman languages and European languages.

The Indo-Aiyan languages including in the Sanskrit, Hindi, Bengali, Marati, Gujarathi, Oriya, Panjabi, Bihari, Rajasthani, Assami, Sindhi and Kashmiri languages spoken by 3/4th of Indian population.
The Dravidian languages include Tamil, Kannada, Telugu and Malayalam.
The Austric languages include Mundari, Santhali, Maithili, Dogri and etc.
Tibeto – Burman languages: Tribal languages and dialects of North and North-East India belong to this category. For example Manipuri, Bodo, Ladiki, Khuki, etc.
The European languages include English, Portuguese and French. These last two languages are spoken mostly people in Goa and Pondicherry.

(3) Religious Diversities: There are eight major religious communities in India. Hindus constitute 82.7%, Muslims 11.8%, Christians 2.6%, Sikhs 2%,-Buddhists 0.7%, Jains 0. 4%, Zoroastrians 0.3%, and Jews 0.1%. Each major religion is sub – divided along the lines of religious documents, sects, and cults. The Hindus are now broadly divided into Shaivite (worshippers of Shiva), Vaishnavaite (worshippers of Vishnu and his incarnations), Shakta (worshippers of the Mother Goddess in various manifestations. Even among them there are sub – divisions based on doctrinal and ritual differences.

Buddhism was spread widely in India once, it lost its hold in the country of its birth and remained confined only to a few pockets. Jainism too, once held wide sway in India, and though its followers are now numerically small, they are found in both the northern and southern States. They have two main divisions: Digamber- unclothed, and Shwetamber. The Indian Muslims are divided broadly n o the Sunni and Shia communities.
Indian Christians are divided into Roman a’.holics and Protestants and into many denominational churches.

Sikhism is synthesizing religion that emphasizes egalitarianism. Grantha Sahib is the holy book and Amritsar Golden Temple is one of their holy piligrimage. The Parsis are a small community, but they have played an important role in India’s industrial development. The Jewish has been established in India for over a millennium. They also had White and Black divisions and prohibition on inter-marriage and inter-dining, but they all worshipped in the same synagogues. The Jati-like restrictions are much less in Bombay and Cochin, to where many Jews have migrated.

(4) Cultural and Ethnic Diversities: Another important source of diversity is the cultural diversity. The people differ considerably in their social habits. Cultural difference varies from state to state. The conflicting and varying shades of blood, strains, culture and modes of life, the character, conduct, beliefs, morals, food, dress, manners, social norms, Socio-Religious customs, rituals and etc. causes cultural and ethnic diversities in the country. Dr. R.K. Mukherji rightly said that “India is a museum of cults and customs, creeds and culture, faiths and tongues, racial types and social systems”. Another important source of diversity is the cultural identity of particular communities and region.

Question 4.
Define Unity and explain the factors of unity in India.
Answer:
Unity implies one-ness ora sense ofwe-ness. Meaning of integration wherein hitherto divisive people and culture are synthesized into a united whole, along with higher levels of co operation, mutual understanding, shared values, common identity and national consciousness. It lightly holds together the various relationships of ethnic groups or institutions in a neatly combined through the bonds of planned structure, norms and values.

In India aspects of Diversity & Unity co-exist, which follows as :

(2) Linguistic Unity: Despite the presence of number of languages, India also possesses lingual unity. Sanskrit as a common base of Indian languages provides the basis of unity as a result of which the linguistic multiplicity has been solved. Simultaneously Sanskrit became the language of Hindu culture and all classics were composed in this language, which demanded reverence and respect. People may speak different languages in different regions but they have common language of English and Hindi to communicate with each other. The formation of linguistic states and using regional languages as medium of teaching at schools, colleges and universities are the products of Independence.

The worshippers may visit different centres of pilgrimage, but all have a common goal of “Earning religious merit by visiting a sacred place”. India is the sacred land not only for the Hindus but also for Sikhs, Jains and Buddhists. The Muslims and Christians too have several sacred centres of pilgrimage in India.

(4) Cultural Unity: In art and architecture, dress and food, literature, music and dance, sports and cinema, medicine and technology there was a fusion of style and the emergence of new forms which were the result of their combined efforts. Thus it became apparently clear from the above account that running through various diversities. India has been helped both by nature and nurture, by her geographical condition and historical experiences, by her religious ethics, and political ideas. To realize a unity to perceive, preserve and strengthen the thread of basic unity which makes India a fine example of unity in diversity, transcending birth, caste, language, ethnicity and religious groupings to establish a big society and a big nation.

Modern education, the development of a network of transport and communications, industrialization and urbanization provided new bases for unity.

Question 5.
Define National Integration and explain challenges to National Integration.
Answer:
National integration refers to national unity and a sense of belonging to the nation. It is an essential aspect in the making of a nation. Promotion of national integration is regarded as a part and parcel of the policy of any country. Many scholars have defined national integration in different ways. Among these Benjamin’s definition on national integration is quoted here According to Benjamin “National integration refers to the assimilation of the entire people of a country to a common identity”.

In simple words, National Integration refers to the process wherein a feeling of togetherness, a sense of national unity and above all, a sense of national belongingness is developed among people. It is in this context, the concept of ‘national integration’ has assumed importance. There are many challenges to national integration.

They are as follows:

Regionalism
Communalism
Linguism
Extremism and Terrorism

(1) Regionalism: Regionalism is expressed in the desire of people of one region to promote their own regional interest at the expense of the interests of other regions. It has often led to separatism and instigated separatist activities and violent movements. It has also gained tremendous momentum during the recent years because it is exploited by the selfish politiciAnswer: Thus, regionalism has challenged the primacy of the nationalistic interests and undermines national unity. Regionalism assumed mainly four forms.

(2) Communalism: Communalism is the antagonism practiced by the members of one community against the people of community and religion. Bipin Chandra holds that communalism is the product of a particular society, economy and polity, which creates problems. Asghar Ali Engineer, Moin Shakir and Abdul Ahmed try to explain communalism as an ideological tool for propagation of economic and political interests. According to them, it is an instrument in the hands of the upper class to concentrate power by dividing people. The elites strive to maintain a status quo against transformation by dividing people on communal and religious lines.

(4) Extremism and Terrorism: Extremism and terrorism have emerged during the recent years as the most formidable challenges to national integration. Extremism refers to the readiness on the part of an individual or group to go to any extreme even to resort to undemocratic, violent and harmful means to fulfil one’s objectives. In the past India has been facing the problems of terrorism since independence. India has faced this problem in Nagaland (1951), Mizoram (1966), Manipur (1976), Tripura (1980) and West Bengal in (1986).

Question 6.
Explain national population policy
Answer:
In 1977, ‘family planning’ was renamed as “family welfare”. The Government of India adopted the UNPF (United Nations Population Fund) guideline of delaying the -first child and spacing the subsequent birth(s);

The Primary health Centres are engaged in family planning programmes, perform two specific functions: providing services to the people and disseminating information about these services in an effective manner in order to motivate the people to accept family planning. The major objective of family planning is “To increase individual happiness and to enhance health of the society”.

National Population Policy 2000 [NPP-2000]: is the latest in the series. It reaffirms the commitment of the government towards administering family planning services. The object of NPP-2000 is to bring the total fertility rate (TFR) to replacement levels by 2010. It contains the goals and the target to be achieved by 2010.

They can be briefed here.

Reduce infant mortality rate.
Reduce maternal mortality ratio.
Achieve universal immunisation of chilcjren against all preventable diseases.
Achieve institutional deliveries by trained persons.
Achieve 100% registration of births, deaths, marriage and pregnancy.
Prevent and control communicable diseases.
Promote vigorously the small family norm to achieve TFR.
Contain the spread of AIDS (Acquired Immuno Deficiency Syndrome).
Address the unmet needs for basic reproductive and child health services, supplies and infrastructure.
Make school education up to age 14 free and compulsory and reduce drop-outs at primary and secondary school levels.
Achieve universal access to information/counselling and services for fertility regulation and contraception. .
To take appropriate steps to make family welfare programme a people-centred programme.

Question 7.
Define Demography. Explain the characteristics of demographic profile of India.
Answer:
Demography is the systematic study of population. The term Demography is derived from two Greek words i.e. demos (people) and graphein (describe), implying the description of people. The term Demography was coined by Achille Guillard in 1855. Demography studies the trends and processes associated with population including – changes in population size; patterns of births, deaths, and migration; and the structure and composition of the population, such as the relative proportions of women, men and different age groups.

The American census of 1790 was probably the first modem census, and the practice was soon taken up in Europe as well in the early 1800s. In India, census was conducted by the British Indian government between 1867-72, and regular ten yearly (decennial) censuses have been conducted since 1881. Independent India continued the practice, and seven decennial censuses have been conducted since 1951, the most recent being in 201L Demographic data are important for the planning and implementation of state policies, especially those for economic development and general public welfare.

The Major Characteristics of the Demographic Profile of India:

Size and Growth of India’s Population
Age Structure of the Indian Population
Sex-Ratio in India
Birth Rate and Death Rate
Increasing Literacy Rate of Indian Population
Increasing Rural-Urban Differences

(1) Size and Growth of India’s Population: India is the second most populous country in the world after China. According to 2011 census India’s population is 121 crores (1.21 billion). Between 1901-1951 the average annual growth rate did not exceed 1.33%, a modest rate of growth. In fact between 1911 and 1921 there was a negative rate of growth of-0.03%. This was because of the influenza epidemic during 1918-19 : 5% of the total population of the country. The growth rate of population substantially increased after independence from British rule going up to 2.2% during 1961-1981. Since then although the annual growth rate has decreased it remains one of the highest in the developing world.

(4) Increasing Literacy Rate of Indian Population: Literacy varies considerably across gender, regions, and social groups. As can be seen from Table No. 4, the literacy rate for women is almost 22% less than the literacy rate for men. However, female literacy has been rising faster than male literacy, partly because it started from relatively low levels. Female literacy rose by about 11.2 percent between 2001 and 2011 compared to the rise in male literacy of 6.2 percent in the same period.

Female literacy was 8.9% in 1951 has increased to 65.4 in 2011 male literacy in the same period wan 27.2% has increased to 82.17. In 1951 total literacy rates 18.3% has increased to 74.04 in 2011.

(5) Increasing Rural-Urban Differences: According to 2011 Census, 68.8% population lives in rural areas while 31.2% people live in urban areas. The urban population has been increasing its share steadily, from about 17.3% in 1951 to 31.2 in 2011, an increase of about two-and-a-half times. The results of the 2001 census fully validate the diagnosis of India’s population problem in terms of the dominance of BIMARU States (Bihar, MP, Rajastan, UP) accounted for 39% of India’s population, 42% of growth and 48% of the total Illiterate population and the adverse role of these BIMARU states.

To take note of the alarming data from 2001 census to coin another acronym – DEMARU – where D stands for daughter and MARU stands for killing. In English “E” will denote elimination. Punjab, Hariyana, Himachal pradesh & Gujarath, Maharashtra as DEMARU states, where the sharp decline in the Juvenile sex ratio. These states are in the perverse practice of Foeticide. The unholy alliance between Tradition (Son complex) and Technology (Ultra sound etc.) is playing havoc with Indian society pre-birth sex determination tests & sex selective abortion are rampant in these states. During the last decade in Punjab, the sex ratio (0-6 age group) declined from 875 to 793.

In Hariyana the sex ratio (0-6 age group) decreased from 879 to 820. In Maharashtra every single district showed a decline in the Juvenile sex ratio between 1991-2001. In Himachal pradesh from 951 to 845. In Gujarath from 928 to 878. In Chandigarh from 899 to 845 and in Delhi from 915 to 865. Even though an increase in the sex ratio for the total population was 927 in 1991 to 933 to 2001. In contrast the sex ratio of child population (0¬6 age group) which was 945 in 1991 decreased to 927 in 2001. In short the girl child (below 6 years) has lost out badly.

Question 8.
Explain the Demographic profile of Karnataka.
Answer:
According to 2001 census, Karnataka with an area of 1,91,791 sq. km. has a population of 52,850,562 with 26,898,918 males and 25,951,644 females. According to 2011 Census, the Population of Karnataka has increased to 6,10,95,297 (Males – 3,09,66,657; Females – 3,01,28,640)withasexratioof973 females for every 1000 males. Karnataka with a population of 6,10,95,297, retains the ninth rank as in 2001, in pupulation among all the 28 States, and seven Union Territories (including the National Capital Territory of Delhi) and accounts for 5.05 per cent of Country’s pupulation of 1,21,05,69,573 in 2011.

1. Rural – Urban population in Karnataka: Among the districts within the State, Bengaluru District is the most pupulated District with 96,21,551 persons and accounts for 15.75 percent of the State’s total pupulation while Kodagu District with a pupulation share of 0.91 per cent is the least pupulated District.

In terms of percentage, 61.33 per cent are Rural residents and 38.67 per cent are Urban residents. In terms of urbanization, the State has witnessed an increase of 4.68 per cent in the proportion of Urban pupulation in the last decade. Among the districts, Bengaluru is the most urbanized District with 90.94 per cent of its pupulation residing in Urban areas followed by Dharwad District (56.82 per cent), Dakshina Kannada District (47.67 per cent), Mysuru District (41.50 per cent) and Ballari District (37.52 per cent). The least urbanized District in the State is Kodagu with 14.61 per cent, preceded by Koppal District (16.81 per cent), Mandya District (17.08 per cent), Chamarajanagar District (17.14 per cent) and Yadgiri District (18.79 per cent).

During the decade 2001-11, the State population witnessed a net addition of 82,44,735 persons to its 2001 population of 5,28,50,562. Among the districts, Bengaluru District, has witnessed the highest decennial growth rate of 47.18 per cent followed by Yadgir, the newly created District, with 22.81 per cent.

Chikkamagaluru District, a predominantly plantation area in the Malnad region, is the only District in the State which has registered a negative growth rate of -0.26 per cent. Kodagu District another plantation area in the Malnad region with a growth rate of 1.09 per cent ranks 29, just above Chikkamagaluru District.

2. Sex Rartio in Karnataka: The Sex Ratio in Karnataka has increased from 965 in 2001 to 973 in 2011. The Sex Ratio for Scheduled Caste and Scheduled Tribe population is identical at 990 and is significantly higher than that of the State. Among the districts, the highest overall Sex Ratio of 1094 is recorded in Udupi District and the lowest of 916 is recorded in Bangalore District. Female population is higher than male population in Chikmagalur, Kodagu (1019), Hassan, (1012) Dakshina Kannada (1020) and Udupi (1094).

Inspite of favourable Sex Ratio, it has declined in Udupi (-36) and Dakshina Kannada (2).

3. Density in Karnataka: Density of Population: The number of persons’for every square Km.area is called the density of population. According to 2001 census Bengaluru Urban District has registered the highest density of 2,985 persons per sq. km and the lowest density per sq.km, was recorded in Kodagu (134) and Uttara Kannada (132) districts. The density of population of the state was 319 in 2011 as against 276 in 2001. The density of population of Bengaluru metropolitan city was 4,378 in 2011 as against to 2985 in 2001. Uttar Kannada (140) and Kodagu (135) have the lowest density of population in the State.

4. Seheduled caste population in Karnataka: The Scheduled Caste population in the State has increased from 85,63,930 in 2001 to 1,04,74,992, in 2011, registering a decennial growth rate of 22.32 per cent. The Scheduled Caste population constitutes 17.15 per cent of the total population of the State. The highest proportion of Scheduled Caste population is returned from Kolar District with 30.32 percent, followed by Chamarajanagar District with 25.42 per cent. The least proportion of Scheduled Caste population is recorded in the coastal district of Udupi (6.41 per cent) District.

5. Seheduled Tribe population in Karnataka: The Scheduled Tribe population in the state has increased from 34,63,986 in 2001 Census to 42,48,987 in 2011, registering a decennial growth rate of22.66 per cent. The proportion of the Scheduled Tribe population to total population of the State is 6.95 per cent. The highest proportion of Scheduled Tribe population is in Raichur District (19.03 per cent) and the least proportion is returned from Mandya District (1.24 per cent).

6. Literacy Rate in Karnataka: Literacy Rate of the State has increased from 66.64 per cent in 2001 to 75.36 per cent 2011. While the Male Literacy has increased from 76.10 per cent to 82.47 per cent, the Female Literacy rate has increased from 56.87 per cent to 68.08 per cent.

Among the districts, Dakshina Kannada District with overall Literacy rate of 88.57 per cent retains its top position, closely followed by Bengaluru District (87.67 per cent) and Udupi District (86.24 per cent). The lowest overall Literacy rate of 51.83 per cent is recorded in the newly created Yadgir District, preceded by Raichur District which has recorded 59.56 per cent.

2nd PUC English Textbook Answers Springs Chapter 10 Heaven, If you are not here on Earth

October 30, 2023

Students who are in search of Heaven, If you are not here on Earth Questions and Answers Pdf, Notes, Summary. First check in which chapter you are lagging and then Download Karnataka Board 2nd PUC English Textbook Answers Chapter Wise. Students can build self confidence by solving the Answers with the help of Karnataka State Board 2nd PUC. English is the scoring subject if you improve your grammar skills. Because most of the students will lose marks by writing grammar mistakes. So, we suggest you to Download Karnataka State Board 2nd PUC English Answers according to the chapters.

Karnataka 2nd PUC English Textbook Answers Springs Chapter 10 Heaven, If you are not here on Earth

Check out the topics covered in 2nd PUC before you start your preparation. Improve your grammar skills with the help of Karnataka 2nd PUC English pdf links. The Answers for Karnataka 2nd PUC English Textbook Springs Chapter 10 Heaven, If you are not here on Earth are prepared by the English experts. So, if you follow Karnataka 2nd PUC English Textbook Answers you can cover all the topics in 2nd PUC Chapter 10 Heaven, If you are not here on Earth. This helps to improve your communication skills.

Heaven, If you are not here on Earth Questions and Answers, Notes, Summary

Heaven, If you are not here on Earth Comprehension I

Heaven If You Are Not On Earth KSEEB Solution Question 1.
According to the poet, ‘heaven’ is
(a) on earth
(b) within us
(c) elsewhere.
Answer:
(a) on earth.

Heaven If You Are Not On Earth Summary KSEEB Solution Question 2.
The poet finds god in
(a) nature
(b) earth
(c) temple.
Answer:
(a) nature.

Heaven If You Are Not On Earth Notes KSEEB Solution Question 3.
What does the line ‘Heaven lies all over’ suggest?
Answer:
Heaven is not elsewhere but within us and is all over the world. If we are good, then that is heaven and if we are bad that is hell. Nature is also a heaven as we can find heaven in objects like trees, moon, sands of grains etc.

Heaven If You Are Not On Earth Summary 2nd Puc KSEEB Solution Question 4.
Who creates’heaven on earth’?
OR
Where does the poet create heaven?
Answer:
The poet creates heaven on earth.

Heaven, If you are not here on Earth Comprehension II

Heaven Is A Product Of Human Effort KSEEB Solution Question 1.
Describe the beauty in nature that makes the earth a heavenly place.
OR
How has the poet glorified heaven on earth?
Answer:
The poet Kuvempu argues that we do not need to seek heaven after death. We can enjoy the heavenly bliss in looking at Nature on this earth itself. The poet presents a beautiful description of nature’s beauty. The poet emphatically states that the bliss that one experiences while looking at the streams that are leaping down, roaring from the top of the hills, the waves that come rolling across the seas carrying surf at their edges, the tender rays of sunlight falling on the sprawling green forests and the gentle sun warming up the earth make this earth a heavenly place.

Heaven If You Are Not Here On Earth Summary KSEEB Solution Question 2.
Why does the poet feel that earth is more beautiful than heaven?
Answer:
According to Kuvempu, there is no heaven in reality. Entities like ‘God’, ‘Heaven’, and the nymphs are merely figments of man’s imagination. All the different forms of nature like the green forests, the streams that leap down the hills, the waves that roll across the seas, the moonlit night, and the splendour of harvest appear more beautiful than the imaginary descriptions of the man.

Heaven, If you are not here on Earth Comprehension III

Heaven If You Are Not Here On Earth KSEEB Solution Question 1.
How does the poet explain the illusion of heaven in the poem?
OR
How does the poem ‘Heaven, if you are not here on Earth’ visualise heaven?
Answer:
Kuvempu considers concepts like ‘God’, ‘nymphs’ and ‘heaven’ as myths created by man. He opines that if at all ‘heaven’ exists it exists only on this earth and nowhere else. In order to break the illusion of heaven in man’s mind, he presents before us charming sights of nature and argues that nothing can be more heavenly than these forms of nature.

He makes a direct address to nature and declares that if heaven does not exist on the earth where else can it be. He justifies his statement referring to the streams, the waves, and the sunlight. He says that the streams that leap down roaring from the top of the hills, the rolling surf at the edge of the waves, the tender rays of sunlight falling on the green forests, the gentle sun warming the earth all these make the earth heaven.

Heaven If You Are Not On Earth Full Poem KSEEB Solution Question 2.
How does the poem celebrate the power of the poet?
Answer:
Having argued categorically that if at all there exists an entity called heaven, it exists only on this earth, the poet Kuvempu presents before the readers’ beautiful sights of nature. In the last stanza, the poet states that one sees chunks of heaven lying all over in the splendour of harvest and of moonlight. He concludes the poem celebrating poetic talent. He says that the poet enjoys looking at Nature, imbibes the beauty of nature and spills the nectar of heaven, on the earth, through his poetry.

Heaven, If you are not here on Earth Additional Questions and Answers

I. Answer the following questions in a word, a phrase, or a sentence each:

Heaven, If You Are Not On Earth Full Poem KSEEB Solution Question 1.
Where can heaven be found, according to Kuvempu?
OR
Where, according to the poet, did he see heaven?
OR
Where does the poet create heaven in the poem ‘Heaven, If You Are Not Here On Earth’?
Answer:
On this earth itself.

Heaven If You Are Not On Earth Poem KSEEB Solution Question 2.
Where does the tender sunshine lean?
Answer:
The tender sunshine leans on gardens green with grass or other rich vegetation.

Heaven If You Are Not On Earth 2nd Puc Notes KSEEB Solution Question 3.
How does the poet create heaven on earth?
Answer:
The poet creates heaven on earth by imbibing the beauty of nature and spilling the nectar of heaven, on the earth, through his poetry.

Heaven, If You Are Not On Earth Summary In English KSEEB Solution Question 4.
What, according to Kuvempu, are only figments of man’s imagination?
Answer:
‘Heaven’, ‘God’, and ‘nymphs’ are not tangible entities but are only figments of man’s imagination.

Question 5.
When, according to the speaker, can there be no Gods?
Answer:
According to the speaker, if we ourselves cannot be gods, then there can be no gods.

Question 6.
According to the speaker, heaven is
(a) on earth
(b) not on earth
(c) beyond earth.
Answer:
(a) on earth.

Question 7.
Who creates heaven on earth?
Answer:
The poet.

Question 8.
How does the poet imbibe and spill the ‘song of nectar’?
OR
What does the poet imbibe and spill to create heaven on earth?
Answer:
The poet absorbs the beauty of nature through his eyes and spills the ‘song of nectar’ through his poetry.

Question 9.
According to the poet makes this Earth heaven.
(a) heavenly nymphs
(b) gods
(c) gentle sun.
Answer:
(c) gentle sun.

Question 10.
What could not be anywhere else but on earth, according to the speaker, in ‘Heaven, If You Are Not Here On Earth’?
Answer:
According to the speaker in the poem ‘Heaven, if You are not Here on Earth’ it is heaven. If heaven is not on the earth, it can be nowhere else.

Question 11.
According to the speaker in ‘Heaven, If You Are Not Here On Earth’, if we ourselves cannot be gods, then there can be no
(a) gods
(b) nymphs
(c) humans.
Answer:
(a) gods.

Question 12.
When can there be no nymphs, according to the speaker, in ‘Heaven, If You Are Not Here On Earth’?
Answer:
According to the speaker in the poem, there can be no nymphs if we ourselves are not heavenly nymphs.

Question 13.
According to the speaker in ‘Heaven, If You Are Not Here On Earth’ if we ourselves aren’t heavenly nymphs, the nymphs are not
(a) everywhere
(b) nowhere
(c) elsewhere.
Answer:
(c) elsewhere.

Question 14.
Who, according to the speaker, imbibes and spills the song of nectar in ‘Heaven, If You Are Not Here On Earth’?
Answer:
According to the speaker in the poem, it is the poet who imbibes and spills the song of nectar.

Question 15.
What does the poet create on earth, according to the speaker, in ‘Heaven, If You Are Not Here On Earth’?
Answer:
According to the speaker in the poem, the poet creates heaven on earth.

Question 16.
What quality of harvest causes heaven to lie all over in ’Heaven If you are not here on Earth’?
Answer:
In ‘Heaven, if you are not Here on Earth’, the splendour of harvest causes heaven to lie all over.

Question 17.
“In the splendour of harvest and of moonlight Heaven lies all over!” The phrase refers to
(a) harvest and moonlight are no match to heaven
(b) splendour is only in heaven
(c) heaven can be seen in the harvest and moonlight
Answer:
(c) heaven can be seen in the harvest and moonlight.

II. Answer the following questions in a paragraph of 80 – 100 words each:

Question 1.
Why does the speaker believe that heaven can be created only by human effort in ‘Heaven, If You Are Not Here On Earth’?
Answer:
The speaker convincingly argues that we do not need to seek heaven in the skies because this very earth is heaven-like in reality. He tries to prove this point by describing a roaring stream and the sunlit verdant garden. The poet emphatically states that the bliss that one experiences while looking at the streams that are leaping down, roaring from the top of the hills, the waves that come rolling across the seas carrying surf at their edges, the tender rays of sunlight falling on the sprawling green forests and the gentle sun warming up the earth make this earth a heavenly place.

The poet presents two more pictures of heaven on earth. They are the splendour of harvest and the beauty of the moonlit night. The poet wishes that the reader brings to mind the harvest season when in every bit of agricultural land do we see heaps of grains covering the land. Then he mentions the moonlit night on which the whole earth seems to be bathed in silvery light. Finally, the poet says that we (the poets) who enjoy such heavenly sights, imbibe the beauty of nature and spill the nectar of heaven through our poetry and thus create heaven on earth.

Question 2.
What image of heaven does the poem, ‘Heaven, If You Are Not Here On Earth’ create?
Answer:
According to the speaker, the poem creates a mesmerizing image of heaven which is reflected in the description of pristine nature created by the poet. There are roaring streams leaping down from the top of the hills. The waves come rolling across the seas carrying surf at their edges. The tender rays of sunlight falling on the vast expanse of green forests and the gentle sun warming up the earth enhance the beauty of the Earth, creating an image of Heaven on earth. The splendour of harvest and the pleasant moonlight that bathes the earth makes it all the more heavenly.

Question 3.
What are the requirements of heaven, according to ‘Heaven, If You Are NotHere On Earth’?
Answer:
As expressed in the poem, Heaven is imagined to be the abode of gods and heavenly nymphs. Besides, it must have roaring and leaping streams, waves rolling with surf at their edge, the vast expanse of green forests being clothed by the tender rays of the sunlight, warmed by the gentle sun during the day and cooled and covered by moonlight at night.

III. Answer the following questions in about 200 words each:

Question 1.
‘Heaven is a creation of one’s own mind’. Explain with reference to ‘Heaven, if you are not here on earth’.
OR
Why does the speaker in ‘Heaven, If You Are Not Here on Earth’ say that heaven is nowhere else but on earth?
Answer:
The very title of the poem suggests the intention of the poet. The poet wishes to argue that concepts like ‘heaven’, ‘god’, nymphs, etc., are not tangible entities but are only figments of man’s imagination. On the other hand, all the imaginary comforts, pleasures, and bliss one believes to enjoy in heaven, can be got in reality on this earth. The poet presents a beautiful picture of the different forms of nature which make this earth a heaven.

The poet claims that the roaring streams that tumble down fast from the top of the hills, the rolling surf at the edge of the waves that come rolling across oceans and seas, the tender rays of the sunbathing the vast green forests, and the gentle sun warming up the earth make this earth a heaven.

Question 2.
‘Heaven lies all over’. How is this brought out in ‘Heaven, if you are not here on earth’?
OR
How does Kuvempu show that heaven is here on earth?
OR
How does the poet recreate and capture the beauty and splendour of heaven on earth?
OR
Describe how the poem ‘Heaven, if you are not on earth’ visualizes heaven in nature.
OR
Why does the speaker heaven, if you are not on earth’ say that heaven is right here on earth?
OR
How, according to the speaker, does one find heaven on earth?
OR
“Heaven is not a separate entity but a part of the earth to be created by human endeavour”. How does the poem ‘Heaven, if you are not here on earth present this?
Answer:
Having convincingly argued that we do not need to seek heaven in the skies, and this very earth is heavenly in reality, the poet presents the splendour of harvest and the beauty of the moonlit night. The poet calls these chunks of heaven. The poet wishes the reader to bring to mind the harvest season when in every bit of agricultural land do we see heaps of grains, covering the land.

He then presents a beautiful picture of the different forms of nature which make this earth a heaven. The poet claims that the roaring streams that tumble down fast from the top of the hills, the rolling surf at the edge of the waves that come rolling across oceans and seas, the tender rays of the sunbathing the vast green forests, and the gentle sun warming up the earth make this earth a heaven.

Secondly, he mentions the moonlit night on which, the whole earth seems to be bathed in silvery light. Finally, the poet tells the reader that the poets who enjoy such heavenly sights imbibe the beauty of nature and spill the nectar of heaven through their poetry. This way the poet creates heaven on earth and celebrates the joys of heaven through his poetry.

Question 3.
How, according to the speaker, can we create heaven on earth?
OR
Human effort alone can create heaven on earth. How is this brought out in ‘Heaven, If you are not here on Earth’?
Answer:
The poet Kuvempu convincingly argues that we do not need to seek heaven in the skies because this very earth is heaven-like in reality. He tries to prove this point by describing a roaring stream and the sunlit verdant garden. The poet emphatically states that the bliss that one experiences while looking at the streams that are leaping down, roaring from the top of the hills, the waves that come rolling across the seas carrying surf at their edges, the tender rays of sunlight falling on the sprawling green forests and the gentle sun warming up the earth make this earth a heavenly place. The poet presents two more pictures of heaven on earth. They are the splendour of harvest and the beauty of the moonlit night.

The poet wishes that the reader brings to mind the harvest season when in every bit of agricultural land do we see heaps of grains covering the land. Then he mentions the moonlit night on which the whole earth seems to be bathed in silvery light. Finally, the poet says that we (the poets) who enjoy such heavenly sights, imbibe the beauty of nature and spill the nectar of heaven through our poetry and thus create heaven on earth.

Question 4.
Heaven is nothing but a replica of the best qualities of Earth. How does the poem ‘Heaven, If You Are Not Here On Earth’justify this statement?
Answer:
” The poem ‘Heaven, if You are not Here on Earth’, makes an attempt to argue that there is no ‘Heaven’ in reality. Entities like ‘God’, ‘Heaven’, and the ‘Nymphs’ are merely figments of man’s imagination. In response to the belief that ‘Heaven’ is mesmerizingly beautiful and is the abode of ‘Gods’ and the ‘Nymphs’, the poem asserts that ‘Heaven’ is only a replica of the resplendent and pristine Nature. When we are treated with kindness and compassion in times of distress and helplessness, we attribute it to the divinity of God. This is only a replica of ‘Man’ giving divine help to his fellow beings in times of distress and saving them.

We believe that nymphs are lesser goddesses of Nature represented as young girls living in trees, streams, mountains, etc. They are none other than a replica of the beautiful and charming girls living in the lap of nature of this earth.

Besides being the abode of the gods and nymphs, ‘Heaven’ is believed to be an incredibly beautiful place. The poem argues that the idea or concept of Heaven’s beauty is only an image of the beautiful earth which abounds in roaring streams leaping down the mountains and the rolling waves carrying surf at their edges. This enchanting beauty of the earth is enhanced by the tender sunshine clothing the vast expanse of green forests, and the warmth of the gentle sunbathing the greenery. To top all this is the moonlight, which makes our nights tranquil and pleasant.

On the whole, one can conclude that it is our human kindness and compassion that comes to be termed as ‘divinity’ and the pristine Nature that creates Heaven on earth.

Question 5.
‘It is not gods that make heaven but humans who attain divinity’. How is this brought out in ‘Heaven, If You Are Not Here On Earth’?
Answer:
The idea that ‘it is not gods that make heaven but humans who attain divinity’ is expressed in lines three and four:

“If we ourselves cannot be gods
Then there can be no gods!”

According to the poet, heaven is only a replica of the resplendent and pristine nature. There is no distinct or substantial entity called ‘God’ and it is the man who makes the earth ‘Heaven’. When ‘man’ treats his fellow beings with kindness and compassion in times of distress and helplessness, he attains divinity. He is as divine as God. This is only a replica of a man giving divine help to his fellow beings in times of distress and saving them. It is such human beings with divine kindness and compassion that make ‘Heaven’ on earth.

Question 6.
‘Heaven, If You Are Not Here On Earth’ tries to remove the traditional picture of heaven. Discuss.
Answer:
” The poem ‘Heaven, if You are not Here on Earth’, makes an attempt to argue that there is no ‘Heaven’ in reality. Entities like ‘God’, ‘Heaven’, and the ‘Nymphs’ are merely figments of man’s imagination. In response to the belief that ‘Heaven’ is mesmerizingly beautiful and is the abode of ‘Gods’ and the ‘Nymphs’, the poem asserts that ‘Heaven’ is only a replica of the resplendent and pristine Nature. When we are treated with kindness and compassion in times of distress and helplessness, we attribute it to the divinity of God. This is only a replica of ‘Man’ giving divine help to his fellow beings in times of distress and saving them.

On the whole, one can conclude that it is our human kindness and compassion that comes to be termed as ‘divinity’ and the pristine Nature that creates Heaven on earth.

Question 7.
Both human nature and the natural world play a vital role in the creation of heaven. Explain with reference to ‘Heaven, If You Are Not Here On Earth’.
Answer:
” The poem ‘Heaven, if You are not Here on Earth’, makes an attempt to argue that there is no ‘Heaven’ in reality. Entities like ‘God’, ‘Heaven’, and the ‘Nymphs’ are merely figments of man’s imagination. In response to the belief that ‘Heaven’ is mesmerizingly beautiful and is the abode of ‘Gods’ and the ‘Nymphs’, the poem asserts that ‘Heaven’ is only a replica of the resplendent and pristine Nature. When we are treated with kindness and compassion in times of distress and helplessness, we attribute it to the divinity of God. This is only a replica of ‘Man’ giving divine help to his fellow beings in times of distress and saving them.

On the whole, one can conclude that it is our human kindness and compassion that comes to be termed as ‘divinity’ and the pristine Nature that creates Heaven on earth.

Heaven, If you are not here on Earth by Kuvempu About the Poet:

K.V. Puttappa (1904-1994) is widely known by the pen name Kuvempu. He is acknowledged as the greatest poet of the 20th century Kannada literature. He is the first among eight recipients of the Jnanpith Award for Kannada.

He is the second – after M. Govinda Pai – among Kannada poets to be revered as Rashtrakavi, a national poet. His work ‘Sri Ramayana Darshanam’, the rewriting of the great ancient Indian epic ‘Ramayana’ in modern Kannada, is regarded as a revival of the era of Mahakavya (Epic poetry) in a contemporary form and charm. He is immortalized by some of his phrases, and in particular for his contribution to Universal Humanism or in his own words ‘Vishwa Manavatha Vaada’. He was conferred the Padma Vibhushan by the Government of India. He has penned the Karnataka State anthem ‘Jaya Bharata Jananiya Tanujate’. The poem has been translated into English by Dr. C. Naganna, a well-known poet, critic, and translator.

In this poem, Kuvempu urges us to take note of nature as a dynamic entity. For him, heaven is not something beyond the boundaries of this world, since the earth itself possesses all that the so-called heaven promises. The poet’s decisive inference that heaven is here on earth and nowhere else is seen from the beginning to the end. According to him, the stream, the waves, the splendour of harvest, the sunshine, the celestial eye, the moonlight – are all nothing but the reflection of that unseen paradise called heaven.

Heaven, If you are not here on Earth Summary in English

This poem is the English version of’Swargave, Bhoomiyoliradire Neenu’, a poem in Kannada, written by Kuvempu, one of the most revered poets of Karnataka.

The poem makes an attempt to give the reader a glimpse into the rationalistic outlook of the poet. The poet has tried to argue that concepts like ‘Heaven’, ‘God’, ‘Nymphs’ etc., are man’s creations. Entities like ‘Heaven’ and ‘God’ exist only on the Earth and you cannot find them anywhere else. The poet suggests that one should perceive divinity and enjoy the heavenly bliss in the company of Nature itself. The poet strongly believes that ‘Heavenliness and worldliness’ are not distinct or two separate entities and argues that there is no difference between worshipping or adoring Nature and worshipping or adoring God.

In the first two lines the poet addresses ‘Heaven’ and declares emphatically that if Heaven does not exist on the Earth where else can it be. It only means that the reader needs not look for ‘Heaven’ in the skies; if at all there is an entity called ‘Heaven’ one must find it on the earth only and nowhere else.

2nd PUC English Textbook Answers Springs Chapter 10 Heaven, If you are not here on Earth image - 1

The poet refers to our beliefs about ‘Gods’ and ‘heavenly nymphs’. The poet expresses his conviction that there is no distinct or substantial entity called ‘God’ and it is Man himself who is God. Similarly, there exist no entities called ‘heavenly nymphs’. He firmly believes that we ourselves are the nymphs, and the nymphs are to be found nowhere else but on this earth only.

The poet wants to dispel the popular notion that gods and nymphs live in heaven. The poet, who wants to dismiss such beliefs, tells the reader that we ourselves should become gods and nymphs. The poet wants us to give up our belief that we go to heaven after death where we find gods and nymphs.

The poet tries to introduce us to the different forms or parts of heaven that exist on the earth. He presents a mesmerizing picture of ‘Nature’ in its pristine form. The poet states that the ‘bliss’ that we experience when we look at the streams that are leaping down, roaring, from the top of the hills, the waves that come rolling across the seas carrying surf at their edges, the tender rays of sunlight falling on the vast expanse of green forests and the gentle sun warming up the earth make this Earth, ‘Heaven’.

The poet refers to the beauty of the harvest season and the moonlit night. He declares that one enjoys heavenly bliss when one watches the splendour of harvest and the moonlit night. The poet ends the poem saying that the poet who imbibes this heavenly bliss, spreads the nectar of Heaven through his poetry on this earth.

A poet is endowed with a higher degree of imagination and sensibility. With these qualities, the poet appreciates nature’s beauty and in turn, the poet enables others to behold heaven on earth.

To sum up, the poet argues that we do not need to seek heaven after death, but can enjoy heavenly bliss even when we are alive, if only we have the ‘eyes’ to see ‘Heaven’ on this earth. ’Heaven’ exists only on the earth and nowhere else. One is sure to enjoy the pleasures of heaven when one looks at the splendour of Nature. The poet urges the reader to perceive the tremendous energy that lies underneath the physical beauty of Nature. This idea can be taken as the message of the poem.

Heaven, If you are not here on Earth Summary in Kannada

2nd PUC English Textbook Answers Springs Chapter 10 Heaven, If you are not here on Earth image - 2

Glossary:

nymph: damsel
Froth: foam
Verdant: green with grass or other rich vegetation
Nectar: the drink of the Gods

The main aim is to share the knowledge and help the students of 2nd PUC to secure the best score in their final exams. Use the concepts of Karnataka 2nd PUC English Answers Chapter 10 Heaven, If you are not here on Earth in Real time to enhance your skills. If you have any doubts you can post your comments in the comment section, We will clarify your doubts as soon as possible without any delay.

2nd PUC English Textbook Answers Springs Chapter 13 Where there is a Wheel

October 29, 2023

Students who are in search of Where there is a Wheel Questions and Answers Pdf, Notes, Summary. First check in which chapter you are lagging and then Download Karnataka Board 2nd PUC English Textbook Answers Chapter Wise. Students can build self confidence by solving the Answers with the help of Karnataka State Board 2nd PUC. English is the scoring subject if you improve your grammar skills. Because most of the students will lose marks by writing grammar mistakes. So, we suggest you to Download Karnataka State Board 2nd PUC English Answers according to the chapters.

Karnataka 2nd PUC English Textbook Answers Springs Chapter 13 Where there is a Wheel

Check out the topics covered in 2nd PUC before you start your preparation. Improve your grammar skills with the help of Karnataka 2nd PUC English pdf links. The Answers for Karnataka 2nd PUC English Textbook Springs Chapter 13 Where there is a Wheel are prepared by the English experts. So, if you follow Karnataka 2nd PUC English Textbook Answers you can cover all the topics in 2nd PUC Chapter 13 Where there is a Wheel. This helps to improve your communication skills.

Where there is a Wheel Questions and Answers, Notes, Summary

Where there is a Wheel Comprehension I

Where There Is A Wheel KSEEB Solution Question 1.
What does cycling as a symbol of social mobility mean?
Answer:
Cycling through the word evokes minute meaning, it is broader when it comes to society. Social mobility has two meanings literally. It moves society from one place to another, which means the transportation of people, and it also connotes the progress of society. When women want to go for a market they do not want to wait for men they could go on themselves.

Where There Is A Wheel Summary KSEEB Solution Question 2.
When Jameela Bibi says, “It is my right. We can go anywhere”, she is
a. asserting her right to move freely.
b. suggesting mobility leading to liberation.
c. expressing her indifference to dirty remarks.
d. boasting about her cycling skill.
Answer:
(a) asserting her right to move freely.

Where There Is A Wheel Notes KSEEB Solution Question 3.
What does bicycle represent for the rural women?
OR
Mention any one of the symbols which bicycling represents for the women.
Answer:
For the rural women bicycle represents ‘freedom’.

Where There Is A Wheel Questions And Answers KSEEB Solution Question 4.
What is common between neo-literates and neo-cyclists?
Answer:
Cycling as a social movement was first popularized by the neo-literates. These neo-literates are the ones who became neo-cyclists.

Where There Is A Wheel 2nd Puc Notes KSEEB Solution Question 5.
‘Enforced routine’ and ‘male imposed barrier’ refer to
a. confining women to the kitchen.
b. subjecting women to drudgery.
c. status of women in a patriarchal society.
d. lack of freedom of movement
Answer:
(c) status of women in a patriarchal society.

Where There Is A Wheel Pdf KSEEB Solution Question 6.
How did the men react to women taking to cycling?
Answer:
Initially, women had to put up with vicious attacks on their character. Some of the men made filthy remarks. But later, once it got social sanction from the Arivoli organization, some menfolk became less hostile and some of them even encouraged the women to learn cycling.

Where There Is Wheel KSEEB Solution Question 7.
What do the phrases ‘flags on the handlebar’ and ‘bells ringing’ suggest?
Answer:
The phrases ‘flags on the handlebar’ and ‘bells ringing’ refer to the women’s cycle rally organized on International Women’s Day in 1992 in which 1500 female cyclists took Pudukkottai by storm.

2nd Puc English Where There Is A Wheel Notes KSEEB Solution Question 8.
Why did UNICEF sanction mopeds to Arivoli women activists?
Answer:
The UNICEF sanctioned fifty mopeds to Arivoli women activists in appreciation of their work of initiating more than 70000 women to display their cycling skills at the public ‘exhibition-cum-contests’ in a single week in 1992.

Question 9.
Why is the cycle called ‘the humble vehicle’?
Answer:
The word ‘humble’ means ‘poor’, the lowest in rank. The writer calls the cycle a humble vehicle because it is the most common and ubiquitous vehicle seen even in the most remote of places, a common man’s vehicle.

Where there is a Wheel Comprehension II

Question 1.
What is the role of Arivoli Iyakkam in liberating women?
Answer:
In Pudukkottai district there is an organization called Arivoli Iyakkam (Light of Knowledge Movement) which spearheaded the district’s vigorous literacy drive. N. Kannammal is the central coordinator of Arivoli. This literacy organization conducted literacy campaigns among rural women and created a good number of neo-literates. Later, the Arivoli literacy activists, led by N.

Kannammal pioneered the cycling learning movement and thus gave cycling a social sanction. Later, at Kilakuruchi village, the Arivoli activists conducted a cycling training camp for their neo-literates as well as other women enthusiasts. A large number of those who learnt cycling come to train new learners, as master trainers, and this way they helped nearly 70000 rural women to learn cycling. Thus, by teaching them to read and write along with ‘cycling’, the Arivoli organization liberated a vast majority of the rural women in the Pudukkottai District.

Question 2.
In what different ways has the cycle empowered rural women?
OR
Discuss how the cycling movement has empowered the rural women of Pudukkottai.
Answer:
‘Cycling’ empowers women in many ways. It gives them ‘confidence’ which results from being self-dependent and free to carry out their duties and responsibilities without depending on the menfolk. For example, womenfolk can fetch water from even the remotest source and cart provisions on their own. In this way, cycling emancipates womenfolk psychologically. Cycling also empowers women economically and politically. Cycling boosts their income. Some of the women who sell agricultural or other products within a group of villages can save a great deal of time by going to such places on their bicycles.

Secondly, it gives them much more time to focus on selling their produce. Thirdly, it enlarges the area they can hope to cover. Finally, if they choose to enjoy their leisure, cycling gives them a lot of leisure also. These women, besides performing these jobs, can also perform other chores. Finally, to top it all, it gives them self-respect. They can also participate in political work.

Question 3.
Why does the author describe the Arivoli ‘cycling training camp’s unusual experience?
Answer:
One of the pioneers in encouraging ‘cycling’ and giving it a social sanction was the Arivoli activists. These activists conducted a ‘cycling training camp’ in Kilakuruchi village. The writer, who visited the camp, calls it an unusual experience because, on that Sunday, all the prospective learners had turned out in their Sunday best. The author remarks that one could not help being struck by the sheer passion of the pro-cycling movement. The learners exuded so much enthusiasm and passion for learning cycling.

Question 4.
Do you think neo-literate women taking to cycling contributes to the literacy movement?
Answer:
Yes. Women taking up cycling is as significant as their taking up literacy because cycling gives them mobility, freedom, and independence which together give the women confidence to stand on their own legs. Initially, Sheela Rani Chunkath, a district collector, in 1991 intended to train female literacy activists so as to enable them to spread literacy among women in the interior. Therefore, she included mobility as a part of the literacy drive.

However, cycling played a much more significant role than literacy in the lives of the womenfolk. It gave them confidence, freedom, and independence to eke out an independent existence. Cycling helped them stay attached to their children and family and yet be financially independent. It also helped the women to share family responsibilities with the menfolk.

Question 5.
How does Sheela Rani Chunkath, the district collector, promote the empowerment of women?
OR
Bring out the role of Sheela Rani Chunkath in the empowerment of women in Pudukkottai.
Answer:
In 1991, Sheela Rani Chunkath was the district collector of Pudukkottai. As a part of the female literacy drive, she wanted to train female literacy activists so that literacy would reach women in the interior. She had realized that the lack of mobility among women played a big role in undermining their confidence. Therefore, she included ‘mobility’ as a part of the literacy drive. Naturally, literacy activists learned cycling first. This inspired the neo-literates also to learn cycling. This, in turn, inspired every woman in the village to learn cycling.

The literates, having become neo-cyclists, encouraged every woman in the district to learn cycling, by organizing ‘cycle training camps’. Thus, Sheela Rani Chunkath became instrumental in ‘cycling’ becoming a social movement in the Pudukkottai district. This way Sheela Rani Chunkath should be commended for promoting ‘freedom’ of women by including ‘cycling’ in her literacy project.

Question 6.
How did the women react to the shortage of ladies’ cycle?
Answer:
Once ‘cycling’ as a symbol of women’s liberation caught the imagination of the womenfolk in the Pudukkottai district, every woman in the district volunteered to learn cycling. Not surprisingly, this led to a shortage of ‘ladies’ cycles. These women enthusiasts did not hesitate. They volunteered to buy even ‘gents’ cycles and learned cycling. Later on, many women started preferring the ‘gents’ cycle because it has an additional bar from the seat to the handle and that way it enabled them to go out along with a child also. Even to this day thousands of women in Pudukkottai district ride ‘gents’ cycles. ‘

Where there is a Wheel Comprehension III

Question 1.
How does P. Sainath show that cycling brings about changes beyond economic gains?
Answer:
P. Sainath presents an eye-witness report of ‘cycling’ becoming a social movement. He tells us how tens of thousands of women in the Pudukkottai district of Tamil Nadu got initiated to learn cycling.

The Arivoli activists led by N. Kannammal pioneered the ‘cycling’ movement. As a part of their vigorous literacy drive, these activists learned cycling. Later, they organized ‘cycling training camps’ for other women. Thus, it got social sanction. Once the movement became a big force, every woman in Pudukkottai volunteered to learn cycling. Towards the end of the article, Sainath says, besides giving women freedom, mobility, and independence, it also has definite economic implications.

It boosts their family income. Sainath reports that a section of the women sells agricultural or other products within a group of villages. For them, cycling cuts down on time wasted in waiting for buses. Secondly, it gives them much more time to focus on selling their produce. Thirdly, it enlarges the area they wish to cover. Lastly, it can increase their leisure time.

In conclusion, he says, cycling brings about changes beyond economic gains also. ‘Cycling’ gives them a sense of self-respect which is vital to their sense of well-being. Thus, Sainath says that this humble vehicle has become a metaphor for freedom for these women.

Question 2.
‘O sister come learn cycling, move with the wheel of time…’ How does the song suggest that the cycle could be an instrument of social change and progress?
Answer:
In the article titled ‘Where There is a Wheel’, Sainath, the writer, reports an important social movement that took place during 1992-94 that stunned the people of the Pudukkottai district in Tamil Nadu.

There was an organization named ‘Arivoli lyakkam’ which led the female literacy movement in Pudukkottai. These Arivoli activists learned ‘cycling’ so as to enable themselves to spread the message of literacy among rural women in the interior. Once the activists learned cycling, they, in turn, taught their neo-literates cycling. These neo-literates, neo-cyclists trained women in cycling, all over the district. During this period Muthu Bhaskaran, an Arivoli activist, wrote a famous song to encourage rural women to learn cycling. The song is intended to call upon the womenfolk to learn cycling.

The song conveys a simple message and when it is sung in Tamil, the mother tongue of these rural women, its meaning is easily understood. It only calls upon the woman to set in motion her own wheel of life. Until then, the woman could not move out of the house, because her ‘mobility’ depended on her being able to find money to go by bus. Going by bus also depended on the permission of the menfolk. The woman needed the help of her menfolk even to sell the agricultural produce which grew on her land. This way the woman could not break the male-imposed barriers. Once she learned cycling she got the freedom to move to any place she wanted and the freedom to do any work she wished to.

This song invites the woman to learn cycling and set in motion the wheel of her life. Here, the ‘wheel’ refers to ‘progress’. So, it tells the woman to learn cycling and be responsible for her own progress.

Where there is a Wheel Additional Questions and Answers

I. Answer the following questions in a word, a phrase, or a sentence each:

Question 1.
Name the person who wrote the famous cycling song.
OR
Name the Arivoli activist who wrote/penned the famous cycling song.
Answer:
Muthu Bhaskaran.

Question 2.
In which district of Tamil Nadu, according to P. Sainath, has cycling been a social movement?
OR
Where was cycling started as a social movement, according to P. Sainath?
Answer:
Pudukkottai district in Tamil Nadu.

Question 3.
What is Fatima by profession?
Answer:
A secondary school teacher.

Question 4.
What is the literacy movement of Pudukkottai called?
Answer:
Arivoli Iyakkam.

Question 5.
What does Arivoli Iyakkam mean?
Answer:
Light of Knowledge movement.

Question 6.
What is Arivoli Iyakkam mentioned in ‘Where There is a Wheel’?
Answer:
Arivoli Iyakkam, which means, Light of Knowledge movement, is the literacy movement of Pudukkottai.

Question 7.
Name one of the pioneers of the cycling movement in Pudukkottai.
Answer:
N. Kannammal, the Arivoli central coordinator.

Question 8.
Who is the central coordinator of Arivoli?
OR
Who was the central coordinator of the cycling movement in ‘Where There is a Wheel’?
Answer:
N. Kannammal.

Question 9.
Who was the brain behind the cycling movement?
OR
Who is Sheela Rani Chunkath?
OR
Whose brainchild is the cycling movement, according to P. Sainath?
Answer:
Sheela Rani Chunkath, a former district collector, was the brain behind the cycling movement.

Question 10.
When and why did Sheela Rani start the cycling movement?
Answer:
In 1991, Sheela Rani Chunkath started the cycling movement in the Pudukkottai district with the intention of training female literacy activists so that literacy would reach women in the interior.

Question 11.
What did Sheela Rani add as a part of the literacy drive?
Answer:
Sheela Rani added ‘mobility’ as a part of the literacy drive.

Question 12.
Why did Sheela Rani include mobility as a part of the literacy drive?
Answer:
Since lack of mobility among women played a big role in undermining their confidence, Sheela Rani included mobility as a part of the literacy drive.

Question 13.
In which year and on what occasion was the all-women’s cycle rally held?
Answer:
The all-women’s cycle rally was held on International Women’s Day in 1992.

Question 14.
Who is the owner of Ram Cycles?
Answer:
S. Kannakarajan.

Question 15.
Who was the male Arivoli activist who encouraged the cycling movement?
Answer:
Muthu Bhaskaran was the male Arivoli activist who encouraged the cycling movement.

Question 16.
Who trains women in cycling in Kudimianmalai?
OR
Name the quarry worker and Arivoli volunteer who trains other women.
OR
Name one of the women who has trained young women from their community in the art of cycling.
Answer:
Manormani.

Question 17.
Who is Manormani?
Answer:
A quarry worker and an Arivoli volunteer.

Question 18.
Name the quarry worker and Arivoli volunteer who trains other women to learn cycling.
Answer:
Manormani is the quarry worker and Arivoli volunteer who trains other women to learn cycling.

Question 19.
Which movement helped the ‘neo-literates’ to become ‘neo-cyclists’?
Answer:
The literacy movement helped the ‘neo-literates’ to become ‘neo-cyclists’.

Question 20.
Name the organization that sanctioned fifty mopeds for Arivoli women activists.
Answer:
The UNICEF sanctioned fifty mopeds for Arivoli women activists.

Question 21.
What is the chosen medium for the rural women ofPudukkottai?
Answer:
Cycling is the chosen medium for the rural women of Pudukkottai.

Question 22.
Why did some women prefer to buy gents’ cycles?
Answer:
Some women preferred to buy gents’ cycles because there was not only a shortage of ladies’ cycles, but they could also seat a child on the additional bar.

Question 23.
Name the male Arivoli activist who wrote the famous cycling song.
Answer:
Muthu Bhaskaran, a male Arivoli activist, wrote the famous cycling song.

Question 24.
What had stunned the inhabitants ofPudukkottai on International Women’s Day?
Answer:
The all-women’s cycle rally in which 1500 female cyclists participated, had stunned the inhabitants of Pudukkottai on International Women’s Day.

Question 25.
Which vehicle is called ‘humble’ in ‘Where There is a Wheel’?
Answer:
The writer calls the bicycle a humble vehicle.

Question 26.
What is meant by ‘neo-cyclists’ mentioned in ‘Where There Is A Wheel’?
Answer:
The term ‘neo-cyclists’ in the lesson ‘Where There is a Wheel’, refers to all those rural women in the Pudukkottai district of Tamil Nadu, who have taken to bicycling as a symbol of independence, freedom, and mobility.

Question 27.
Name one of the three women who trained other women in cycling in ‘Where There Is A Wheel’.
Answer:
Jameela is one of the three women who trained other women in cycling, in the lesson ‘Where There is a Wheel’.

Question 28.
Who has benefited the most by cycling, according to P. Sainath?
Answer:
According to P. Sainath, the neo-literate rural women in the Pudukkottai district have been benefited the most by cycling.

Question 29.
Who took to ‘cycling’ as a social movement in ‘Where There Is A Wheel’?
Answer:
As stated in ‘Where There is a Wheel’, tens and thousands of neo-literate rural women in the Pudukkottai district took to ‘cycling’ as a social movement.

Question 30.
What, according to the speaker, has brought a change in the lifestyle of the women of Pudukkottai?
Answer:
According to the speaker, learning to ride a bicycle has brought a change in the lifestyle of the women of Pudukkottai.

Question 31.
In which official position was Sheela Rani Chunkath, according to P. Sainath?
Answer:
According to Sainath, Sheela Chunkath was then the district collector.

Question 32.
Who was S. Kanakarajan mentioned in ‘Where There Is A Wheel’?
Answer:
As mentioned in ‘Where There is a Wheel’, S. Kanakarajan was the owner of Ram Cycles, a shop in Pudukkottai which sold bicycles.

Question 33.
Mention one of the reasons for women buying gents’ cycles, according to P. Sainath.
Answer:
According to P. Sainath, a shortage of ‘ladies’ cycles was one of the reasons for women buying ‘Gents’ cycles.

Question 34.
What led to a shortage of ladies’ cycles in Pudukkottai, according to P. Sainath?
Answer:
According to P. Sainath, ‘cycling’ became a social movement for the rural women in Pudukkottai district. The district’s literacy activists learned cycling. Then, every one of the neo-literates became a neo-cyclist. This encouraged ‘everywoman’ in the district to learn cycling. Consequently, all the ‘ladies’ cycles stocked in the market got sold resulting in a shortage of ‘ladies’ cycles.

Question 35.
Where did the rural women display their new cycling skills, according to P. Sainath?
Answer:
According to P. Sainath, the rural women displayed their new cycling skills in an all women’s cycle rally held in Pudukkottai on International Women’s Day in 1992.

Question 36.
What did banks provide the women of Pudukkottai with, according to P. Sainath?
Answer:
According to P. Sainath, banks provided loans to the women of Pudukkottai for buying bicycles.

Question 37.
What, according to P. Sainath, played a big role in undermining women’s confidence?
Answer:
According to P. Sainath, lack of mobility among women played a big role in undermining their confidence.

Question 38.
Who gave social sanction to cycling in ‘Where there is a Wheel’?
Answer:
According to P. Sainath, it was the Arivoli that gave social sanction to cycling.

II. Answer the following questions in a paragraph of 80 – 100 words each:

Question 1.
Bring out the nature of freedom gained in Pudukkottai with the introduction of the cycle.
Answer:
The introduction of cycling has brought freedom, mobility, and independence to more than 100000 rural women in the Pudukkottai district. There were quite a few rural women who used to go by bus to sell their agricultural products within a group of villages. Now, these women have learnt cycling can go to such places on cycle, and cut down on time, and also cover a larger area.

Secondly, these women needed the help of menfolk to reach even the bus stop. Moreover, they had to rush back early to tend to the children and perform other chores like fetching water. With the introduction of cycling, they have become mobile and independent. They have a lot of freedom to plan they’re occupational as well as their domestic duties and enjoy leisure too.

Question 2.
How has the cycle transformed the lives of many women in Pudukkottai?
OR
Comment on how cycling reduced women’s dependence on men with reference to Sainath’s essay.
OR
What benefits, according to P. Sainath, has cycling brought into the lives of rural women?
OR
How has a bicycle brought a total change in the lives of rural women?
OR
How did cycling transform the lives of women in the Pudukkottai district and help them change with the times?
Answer:
The introduction of cycling in the Pudukkottai district has brought about a tremendous change in the lives of rural women. Cycling has offered a way out of enforced routines around male- imposed barriers. The rural women, in particular, have gained a great deal of confidence on account of their learning cycling. Earlier, they used to be burdened with economic as well as social or familial obligations. Many young mothers had to help the menfolk in earning the family income as well as do domestic chores like tending to children and fetching water from remote places. Even to sell their agricultural produce in nearby villages, they had to carry their produce to the bus stop with the help of their menfolk.

Secondly, they had to come back within a fixed time to tend to little kids at home and to store or fetch water from remote places. Naturally, though they were capable of doing all these duties on their own, their ‘immobility’ created a big hurdle. They had to walk long distances to fetch water and sell their produce. After learning cycling, such women became free and independent. This gave them a lot of confidence. Moreover, the ability to ride a bicycle gave them a lot of self-respect.

Question 3.
Why does P. Sainath say that visiting an Arivoli cycling training camp is an unusual experience?
Answer:
Sainath, the writer, says that visiting an Arivoli ‘cycling training camp’ is an unusual experience because one day when he went to Kilakuruchi village to see the camp he was amazed to see all the prospective learners turned out in their Sunday best. The writer tells us that one cannot help being struck by the sheer passion of the pro-cycling movement. There was a look of determination on their faces.

Question 4.
What role did Sheela Rani play in the cycling movement?
OR
How did Sheela Rani promote the cycling movement?
Answer:
Sheela Rani Chunkath was the district collector of Pudukkottai. In 1991 as a part of the female literacy drive, she wanted to train female literacy activists so that literacy would reach women in the interior. She had realized that the lack of mobility among women played a big role in undermining their confidence. Therefore, she included ‘mobility’ as a part of the literacy drive.

Naturally, literacy activists learned cycling first. This inspired the neo-literates also to learn cycling. This, in turn, inspired every woman in the village to learn cycling. The literates, having become neo-cyclists, encouraged every woman in the district to learn cycling, by organizing ‘cycle training camps’. Thus, Sheela Rani Chunkath became instrumental in ‘cycling’ becoming a social movement in the Pudukkottai district.

Question 5.
How does the author show that cycling is linked to the literacy movement in the district?
OR
How did Sheela Rani Chunkath empower the women of Pudukkottai by including cycling in the literacy drive?
Answer:
Sheela Rani Chunkath was the district collector of Pudukkottai in 1991. As a part of the female literacy drive, she wanted to train female literacy activists so that literacy would reach women in the interior. She had realized that the lack of mobility among women played a big role in undermining their confidence. Therefore, she included ‘mobility’ as a part of the literacy drive.

Naturally, literacy activists learned cycling first. This inspired the neo-literates also to learn cycling. This, in turn, inspired every woman in the village to learn cycling. The literates, having become neo-cyclists, encouraged every woman in the district to learn cycling by organizing ‘cycle training camps’. Thus, cycling came to be linked to the literacy movement in Pudukkottai.

Question 6.
What are the economic implications of cycling?
OR
Discuss how cycling has had definite economic implications on women’s status.
Answer:
Cycling brought many benefits to rural women in Pudukkottai. Besides instilling a lot of confidence in rural women, it also helped them economically. A large section of the rural womenfolk were small producers who used to wait for buses to carry their produce to sell in other villages.

Even to carry their produce to the bus stop they had to depend on fathers, brothers, husbands or sons.
Once these women learned cycling, they became mobile and independent. Learning cycling enabled them to visit a number of villages and sell their produce. Secondly, after learning cycling they were able to combine different tasks with nonchalance. Consequently, one can see many a young mother riding a cycle with a child on the bar and the produce on the carrier. She could also be seen carrying two or three pots of water hung across the back and cycling towards work or home.

Question 7.
How has cycling swept across the district of Pudukkottai?
Answer:
It is not an exaggeration to say that cycling has swept across the district of Pudukkottai. It is not surprising to see even rural agricultural workers riding a bicycle carrying water, their products, and also their children on the bicycle. In fact, cycle-riding women have become ubiquitous in the Pudukkottai district.

Apart from women agricultural workers, quarry labourers, and village health nurses have also taken to cycling. Joining the rush are balwadi and Anganwadi workers, gem cutters and school teachers, and also grama services and mid-day meal workers as well.

Ques 8.
Why was there a shortage of ladies’ cycles in the district of Pudukkottai? How was the problem overcome?
OR
How did the women react to the shortage of ladies’ cycles?
OR
Why was there a shortage of ladies’ cycles in Pudukkottai? How did the women react to it?
Answer:
Once the idea of learning cycling as a sign of women’s emancipation caught on among the womenfolk of Pudukkottai, there was a huge demand for ‘ladies’ cycles. Once the stocks of ladies cycles got exhausted there was a shortage of ‘ladies’ cycles. Consequently, women did not hesitate to buy ‘gents’ cycles as well. In fact, some women even preferred ‘gents’ cycles because the gent’s cycles have an additional bar from the seat to the handle. That way the women realized that they could seat a child on the bar. Consequently, the shortage of ladies cycles was solved by going in for gents cycles.

Question 9.
How has cycling inculcated a sense of self-respect in the women of Pudukkottai?
Answer:
The writer says that it would be very wrong to emphasize only the economic aspect of learning cycling. He says that learning cycling brings a sense of self-respect to the individual. He quotes the opinion of Fatima, a secondary school teacher. She opines that learning cycling is not economic in her case at all. She cannot afford a bicycle, yet she hires one every evening just to feel the goodness, that independence. What she means to say is, when a woman learns to ride a bicycle, she enjoys a sense of achievement.

Women do not need to depend on their husbands to help them physically in carrying their produce to the market. Secondly, nothing can bind them to their homes. They go out, fetch water and provisions, and come back home to attend to their domestic duties and responsibilities at the right time. This way they enjoy a lot of freedom. It is this feeling that gives women a sense of self-respect.

III. Answer the following questions in about 200 words each:

Question 1.
How has cycling become a metaphor for freedom in Pudukkottai?
OR
How does the speaker highlight the freedom and empowerment accomplished due to cycling?
OR
In what different ways does the bicycle help rural women in ‘Where There Is A Wheel’?
Answer:
The idea of empowering women giving them ‘mobility’ came from Sheela Rani Chunkath, the District Collector of Pudukkottai in 1991. She included ‘mobility’ as a part of the literacy drive so as to facilitate female literacy activists to reach rural women in the interior. Once the literacy activists learned cycling, they taught the neo-literates cycling. Once the neo-literates learned cycling, every other woman in the district volunteered to learn cycling. Later, the Arivoli activists organized a ‘Cycling Training Camp’ and created ‘Master trainers’ who in turn conducted several cycling training camps. This way, in a short period of time, over 100,000 rural women in the Pudukkottai district learned cycling.

The author opines that ‘cycling’ gave these women freedom, mobility, and independence. Consequently, these women stopped depending on the menfolk to transport their agricultural produce to bus stops. They themselves carried the produce and sold them in the neighbouring villages and this way covered a larger area. Secondly, they also took care of the young ones at home and completed household chores in time as a result of the proper utilization of time. They could also fetch water from far off places and cart provisions on their bicycle. Thus, ‘cycling’ empowered rural women in many ways. Furthermore, the ability to carry on all the duties and responsibilities on their own gave the women a sense of achievement and self-respect. Thus, the author who witnessed this silent revolution concludes that the humble vehicle – the bicycle – has become a metaphor for freedom, in Pudukkottai.

Question 2.
How does the essay ‘Where There is a Wheel’speak about women and empowerment?
OR
How is ‘wheel’ symbolic of a change in ‘Where there is a wheel’?
OR
A humble vehicle can be a tool for women’s empowerment. Discuss.
OR
A humble vehicle can work wonders in empowering rural women. Explain with reference to ‘Where There Is A Wheel’.
Answer:
In this essay titled ‘Where There is a Wheel’, Sainath reports how learning to ride a bicycle became a social movement and eventually became a symbol of women empowerment in the Pudukkottai district of Tamilnadu. Here, the ‘wheel’ of the cycle has become a symbol for the empowerment of women. The introduction of cycling has brought about tremendous change in the lives of rural women. Cycling has offered a way out of enforced routines around male-imposed barriers.

The rural women, in particular, have gained a great deal of confidence on account of their learning cycling. Earlier, they used to be burdened with economic as well as social or familial obligations. Many young mothers had to help the menfolk in earning the family income as well as do domestic chores like tending to children and fetching water from remote places. Even to sell their agricultural produce in nearby villages, they had to carry their produce to the bus stop with the help of their menfolk.

Apart from women agricultural workers, quarry labourers, and village health nurses have also taken to cycling. Joining the rush are balwadi and Anganwadi workers, gem cutters, school teachers, grama services, and mid-day meal workers as well.

It would be very wrong to emphasize only the economic aspect of learning cycling. Learning cycling brings a sense of self-respect to the individual. The writer quotes the opinion of Fatima, a secondary school teacher. She opines that learning cycling is not economic in her case at all. She cannot afford a bicycle, yet she hires one every evening just to feel the goodness, that independence. What she means to say is, when a woman learns to ride a bicycle, she enjoys a sense of achievement. She feels that she does not need to depend on her husband to help her physically in carrying her produce to the market.

Secondly, nothing can bind her to her home. She becomes mobile. She goes out, fetches water and provisions, and comes back home to attend to her domestic duties and responsibilities at the right time. This way she enjoys a lot of freedom. It is this feeling that gives a woman a sense of self-respect.

Question 3.
Justify the statement ‘Wheel brings progress’ with reference to ‘Where There is a Wheel’.
Answer:
In ‘Where there is a Wheel’, P. Sainath, the writer, refers to the ‘wheel’ of the bicycle to document the spectacular progress achieved by rural women in the Pudukkottai district of Tamilnadu. The title symbolically conveys the ‘progress’ achieved by rural women over a period of one and a half years. ‘Wheel’ is a commonly accepted symbol for progress or movement or mobility. ‘Wheel’ also means continuous progress or movement. While stagnation indicates decay and deterioration, mobility indicates dynamism.

The writer remarks that “people find curious ways of hitting out at their backwardness, of expressing defiance, a hammering at the fetters that hold them”. All these phrases make a reference to how the rural women of Pudukkottai district defied the challenge of the male bastion and broke the fetters that chained them to the confines of their homes by learning to ride a bicycle. These rural women, which included agricultural workers, quarry labourers, village health nurses, ballad is and anganwadis, gem cutters, school teachers, mid-day meal workers, and gram sevikas, had to depend on the male persons at-home – brother, husband, father – to go to the market to sell their produce and to go to their workplaces in addition to doing household chores like carrying water from the village well, cooking, taking care of infants and washing clothes and utensils.

Today, women can be seen doing many tasks on their bicycles. They can be seen carrying water from the well along with their small kids sitting on the bar of their cycle in the front. Many of them bicycle their way to villages to sell agricultural products or to work in the quarries.

Question 4.
People find curious ways of hitting out at their backwardness. How is this brought out in ‘Where There Is A Wheel’?
Answer:
P. Sainath, in his essay ‘Where There is a Wheel’, remarks that “people find curious ways of hitting out at their backwardness, of expressing defiance, a hammering at the fetters that hold them”. All these phrases make a reference to how the rural women of Pudukkottai district defied the challenge of the male bastion and broke the fetters that chained them to the confines of their homes by learning to ride a bicycle.

Sheela Rani Chunkath was the district collector of Pudukkottai. In 1991 as a part of the female literacy drive, she wanted to train female literacy activists so that literacy would reach women in the interior. She had realized that the lack of mobility among women played a big role in undermining their confidence. Therefore, she included ‘mobility’ as a part of the literacy drive. Naturally, literacy activists learned cycling first. This inspired the neo-literates also to learn cycling. This, in turn, inspired every woman in the village to learn cycling. The literates, having become neo-cyclists, encouraged every woman in the district to learn cycling, by organizing ‘cycle training camps’. Thus, Sheela Rani Chunkath became instrumental in ‘cycling’ becoming a social movement in the Pudukkottai district.

Cycling brought many benefits to rural women in Pudukkottai. Besides instilling a lot of confidence in rural women, it also helped them economically. A large section of the rural womenfolk were small producers who used to wait for buses to carry their produce to sell in other villages. Even to carry their produce to the bus stop they had to depend on fathers, brothers, husbands, or sons. Once these women learned cycling, they became mobile and independent. Learning cycling enabled them to visit the number of villages and sell their produce. Secondly, after learning cycling they were able to combine different tasks with nonchalance.

Consequently, one can see many a young mother riding a cycle with a child on the bar and the produce on the carrier. She could also be seen carrying two or three pots of water hung across the back and cycling towards work or home. Besides helping the women economically, cycling brought a sense of self-respect to them. He quotes the opinion of Fatima, a secondary school teacher.

She opines that learning cycling is not economic in her case at all. She cannot afford a bicycle, yet she hires one every evening just to feel the goodness, that independence. What she means to say is, when a woman learns to ride a bicycle, she enjoys a sense of achievement. Women do not need to depend on their husbands to help them physically in carrying their produce to the market. Secondly, nothing can bind them to their homes. They go out, fetch water and provisions, and come back home to attend to their domestic duties and responsibilities at the right time. This way they enjoy a lot of freedom. It is this feeling that gives women a sense of self-respect.

Where there is a Wheel Vocabulary

A. Idioms and Phrases:

An idiom is the special use of language. Idioms do not give the literal meaning of the individual words used in the idiom.
E.g. ‘a change of heart’ would literally mean a heart transplant. However, idiomatically it would mean ‘a change in one’s attitude or feelings’.
i. Look at the following pairs of idiomatic expressions. The meanings of the first pair are given in brackets. Find out the missing ones.

Question 1.
a. to the day (exactly)
b. to this day (even now)

Question 2.
a. take by storm (capture by the sudden or violent attack)
b. the calm before the storm ( ______)
Answer:
A quiet period immediately before a period of great activity or trouble.

Question 3.
a. for all one knows (considering how little one knows)
b. before one knows where one is ( _______ )
Answer:
(or before one knows it) with baffling speed.

Question 4.
a. at/behind the wheel (in control of the situation)
b. put a spoke in somebody’s wheel ( _______ )
Answer:
spoil someone else’s plans and stop them from doing something

Question 5.
a. turn out in one’s best (be well-dressed)
b. as things turned out ( _______ )
Answer:
how something develops/ends.

ii. Identify the sentences in which these expressions have been used in this lesson.

And to this day, thousands of women here ride ‘gents’ cycles.
Flags on the handlebars, bells ringing, over 1,500 female cyclists took Pudukkottai by storm.
For all he knew, I was an undercover agent of the sales tax department.
(Note: This usage is not found in the lesson.)
In Kilakuruchi village all the prospective learners had turned out in their Sunday best.

B. Phrasal verbs are expressions which have a combination of a verb and a preposition.

Some of the phrasal verbs used in this article are given below. With the help of the teacher/ dictionary find out their meanings and use them in sentences of your own.
Take to, give up, run into, hit out at, sweep across, put up with.

Take to: to start doing something as a habit
give up: stop doing something
run into: to meet someone when you did not expect to
hit out at: to fight something vigorously
sweep across: to extend or spread in a wide range
put up with: to accept unpleasant behaviour by someone or an annoying situation without complaining, even though you do not like it.

Additional Exercises

A. Passive Voice:

Question 1.
The rural women of Pudukkottai found a way of hitting out at their backwardness. Cycling ____ (choose) as a medium in doing so. Cycling ______ (learn) by over one-fourth of all rural women. The new skill _______ (display) by over 70,000 of these women.
Answer:
was chosen; was learnt; was displayed.

Question 2.
Arivoli played an important role in the cycling movement. A number of training camps _____ (arrange) by Arivoli. In order to encourage bicycling, songs _____ (sing) by neo-cyclists. The UNICEF ______ (impress) and sanctioned fifty mopeds for Arivoli women activists.
Answer:
were arranged; were sung; was impressed.

Question 3.
Cycling gained momentum gradually. Though dirty remarks _____ (make), women paid no attention. Scores of young women _____ (train) by Jameela and her friends. A direct link ______ (see) by a neo-cyclist woman between cycling and her personal independence.
Answer:
were made; were trained; was seen.

Question 4.
Now we often see a woman doing a four-kilometre stretch on her bicycle to collect water. Even carting provisions from other places ____ (do) on their own. But, when this began, filthy remarks ______ (make). Even then social sanction ______(give) to cycling by Arivoli.
Answer:
was done; were made; was given.

Question 5.
Gents’ cycles have certain advantages. These ______ (prefer) by some women as these have an additional bar from the seat to the handle. A child _____ (can, seat) on it. Carting provisions _____ (can, do) on their own.
Answer:
are preferred; can be seated; can be done.

Question 6.
It was the occasion of International Women’s Day in 1992. Pudukkottai _____ (take) by storm. The town’s inhabitants _____ (stun) by the all-women’s cycle rally. However, it ______ (approve) by all males.
Answer:
was taken; were stunned; was approved.

Question 7.
In no time cycling became a way of life in Pudukkottai. It _____ (introduce) as a tool of social change. Mobility, facilitated by cycling _____ (include) as a part of literacy drive. The idea ______ (propose) by Sheela Rani Chunkath, the district collector.
Answer:
was introduced; was included; was proposed.

Question 8.
Cycling brought a mighty change in the life of women in Pudukkottai. They started moving on their own. Dependence on men _____ (reduce) to some extent. Provisions too _____ (cart) from one place to another and a four-kilometre stretch ____ (cover) on their cycles to fetch water.
Answer:
was reduced; were carted; was covered.

Question 9.
The District Collector’s main aim was to make literacy reach the interior. So mobility _____ (include) as a part of literacy drive. Loans _____ (provide) by banks for women to buy cycles. Specific duties ______ (allot) to each block to promote this drive.
Answer:
was included; were provided; were allotted.

B. Fill in the blanks by choosing the appropriate expressions given in brackets:

Question 1.
Sheela Rani Chunkath included mobility ______ the literacy drive. People made dirty remarks but the neo-cyclists _____ to these remarks. (turned a deaf ear, to come off, as a part of)
Answer:
as a part of; turned a deaf ear.

Question 2.
A lot of women were unable to wait for ladies’ cycles. Therefore they had to _____ men’s cycles. They knew that bicycles would ______ time wasted in waiting for buses. (cut down on, put up with, go in for)
Answer:
go in for; cut down on.

Question 3.
Cycling has _____ Pudukkottai. It has become a movement. It was the ______ of Sheela Rani Chunkath who was the former District Collector. (brainchild, swept across, go out)
Answer:
swept across; brainchild.

Question 4.
In the heart of rural Pudukkottai, young women _____ the roads on their bicycles. Jameela Bibi, who has ______ cycling, told the reporter that it was her right. (taken to, give up, zip along)
Answer:
zip along; taken to.

Question 5.
In Kilakuruchi village all the prospective learners had in ______ their Sunday best. You can’t help ____ the sheer passion of the pro-cycling movement. (a way out, being struck by, turned out)
Answer:
turned out; being struck by.

C. Fill in the blanks with the appropriate linkers:

Question 1.
Cycling made women independent _______ it reduced their dependence on men. _____ it gave them a sense of freedom. _____ they had to put up with vicious attacks on their character. _____ all women in Pudukkottai learnt cycling. (moreover, as, even then, but)
Answer:
as; Moreover; But; Even then.

Question 2.
Cycling has had very definite economic implications. It boosts their income. ______ for sellers it cuts down on time wasted in waiting for buses. ______ it gives them much more time to focus on selling their produce. _______ it enlarges the area they hope to cover. _____ it increases their leisure time, too. (thus, first, furthermore, on top of that)
Answer:
First; Furthermore; On top of that; Thus.

Where there is a Wheel by P. Sainath About the Writer:

Palagummi Sainath (born 1957) is an Indian journalist and photojournalist focusing on social problems, rural affairs, poverty and aftermath of globalization in India. He is the Rural Affairs Editor of The Hindu. A regular contributor to The Telegraph in Kolkata, he also writes for the fortnightly Frontline and the daily Business Line in Chennai.

Sainath has received close to forty national and international journalism awards and fellowships in thirty years as a journalist, including the Ramon Magsaysay journalism award in 2007, the European Commission’s Natali Prize in 1994, the Boerma journalism Prize from the UN FAO in 2001, the Amnesty International global award for human rights journalism in 2000, and the B.D. Goenka Award for excellence in journalism in 2000. In June 2011, Sainath was conferred an Honorary Doctor of Letters degree (DLitt) by the University of Alberta, the university’s highest honour.

Where there is a Wheel Summary in English

This lesson is an article taken from a book titled ‘Everybody Loves a Good Drought’, by P. Sainath, a popular photo-journalist. Besides giving a brief history of ‘cycling’ as a social movement in Pudukkottai, he also reports how a group of women initiated the remaining women in the village to learn ‘cycling’ so as to use it as a symbol of independence, freedom and mobility. Finally, he also comments on the general impact of this on women’s lives in the Pudukkottai district of Tamil Nadu.

The author begins the article commenting that when people hear the caption, “Cycling as a social movement”, it may sound far-fetched to them, but it is true. Then the author remarks that “people find curious ways of hitting out at their backwardness, of expressing defiance, a hammering at the fetters that hold them”.

The author then quotes statistics to prove his point. He reports that over the past eighteen months, 100000 rural women have taken to bicycling as a symbol of independence, freedom and mobility, and their number constitutes over one-fourth of all rural Women in Pudukkottai. Among them over 70000 of them have taken part in public exhibition-cum-contests to proudly display their skills, yet the desire to learn ‘cycling’ and the ‘training camps’ continue.

2nd PUC English Textbook Answers Springs Chapter 13 Where there is a Wheel image - 1

The author then tells us how two of the participants Jameela Bibi, and Fatima, a secondary school teacher, feel about their achievement in cycling. The author quotes Jameela Bibi, who says, “It’s my right, we can go anywhere. Now I don’t have to wait for a bus”. Similarly, he quotes Fatima also. Fatima says, “There is freedom in cycling. We are not dependent on anyone now. I can never give this up!” Jameela, Fatima and their friend Avakanni, all in their early twenties, have trained scores of other young women in the art of cycling.

The author remarks that “Cycling has swept across this district; women agricultural workers, quarry labourers and village health nurses are among its fans. They are now being followed by balwadi and Anganwadi workers, gem cutters, school teachers, gramasevikas and mid-day meal workers. The vast majority are those who have just become literate”. The district’s vigorous literacy drive led by Arivoli Iyakkam (Light of Knowledge Movement) has been quick to tap this energy. The author has spoken to every one of these ‘neo-literate’, ‘neo-cyclist’ women and asserts that there is a direct link between cycling and the neo-cyclists’ personal independence.

Then he reports the opinion of the coordinator who says that the “cycling training has given confidence to women and it has reduced their dependence on men”.

The author says that he often sees a woman doing a four-kilometre stretch on her bicycle to collect water, sometimes with her children. He opines that women can cart provisions from other places on their own. Initially, these women had to put up with vicious attacks on their character. Even filthy remarks used to be made by men. He praises the ‘Arivoli’ organisation for volunteering to give social sanction to cycling.

Next, he mentions the ‘Cycle Training Camp’ that he had seen in Kilakuruchi village. He says that it was an unusual experience to see all the prospective learners who had turned out in their Sunday best. They appeared to be determined to learn cycling. The Arivoli activists produced songs for the neo-cyclists to encourage bicycling. The author quotes a line which says, “O sisters, come learn cycling, move with the wheel of time”.

Then, we learn that those who got trained in cycling came back in large numbers to help new learners. They worked free of charge for Arivoli as ‘master trainers’. Then, he comments that there is not only a desire to learn but a widespread perception among them that ‘all women ought to learn cycling’.

In the next part of the report, the writer gives a brief historical background to cycling as a social movement. He reports that in 1991 a former district collector by name Sheela Rani Chunkath hit on the idea of training female literacy activists so as to reach women in interior villages. She also included ‘mobility’ (for women) as a part of the literacy drive, because lack of mobility among women played a big role in weakening the confidence of women. It is reported that Chunkath paid personal attention to this idea and motivated the banks to give loans to women to buy cycles. Each block was assigned specific duties in promoting the drive. The district collector met with great success in her plan. Due to the initiative taken by her, the literacy activists learned cycling.

This encouraged the neo-literates, and their example was followed by every woman in the village to learn cycling. This led to a shortage of ‘ladies’ cycles. Then the women started using ‘gents’ cycles. In fact, some women even preferred ‘gents’ cycles because it has an additional bar from the seat to the handle. The author then says, even to this day thousands of women here ride ‘gents’ cycles.

On the International Women’s Day in 1992, over 1500 female cyclists with flags on the handlebars, bells ringing, took Pudukkottai by storm. The towns’ inhabitants were stunned by this all women’s cycle rally.

The writer describes the reactions of the men to this social movement. The author gives the opinion of S. Kanakarajan, owner of Ram Cycles. The cycle dealer says that he had seen a rise of over 350 per cent in the sales of ‘ladies’ cycles in one year. But the author believes that the percentage of increase mentioned by the cycle agent is incorrect because a lot of women have gone in for ‘gents’ cycles as they could not wait for ‘ladies’ cycles. Then the writer remarks that not all males were hostile and some men were even encouraging in their attitude. For instance, Muthu Bhaskaran, a male Arivoli activist, wrote the famous cycling song that has become their anthem.

The writer cites the example of Manormani to illustrate how learning to ride a bicycle can help stone quarry workers also. The twenty-two-year-old Manormani is a stone quarry worker and Arivoli volunteer. She works in Kudimianmalai’s stone quarries. According to her, it is vital for her co-workers to learn cycling because their working places are a little cut off from the main road. Those who learn cycling can be mobile (which means they can go home after work and come back the next day, otherwise, they will have to stay there alone in a new place facing a lot of problems or travel by bus every day). The writer says that in 1992, more than 70000 women displayed their cycling skills at the public ‘exhibition-cum-contests’ run by Arivoli. The UNICEF, who were impressed with the achievement of these activists, sanctioned fifty mopeds for Arivoli women activists.

The writer gives his views about cycling as a social movement. He says that cycling boosts income. Some of the women sell agricultural or other produce within a group of villages. For such people, cycling saves time. Secondly, cycling gives you more time to focus on selling your produce. Thirdly, it helps you to cover a larger area. Lastly, it can increase your leisure time too. Earlier, small producers had to carry their produce only by bus and had to depend on fathers, brothers, husbands, or sons even to reach the bus stop. They could cover only a limited number of villages to sell their produce as they had to do so on foot.

Moreover, these women had to rush back early to tend to the children and perform other chores like fetching water. Those who had bicycles now combined these difficult tasks without any anxiety or tension. Even now one can see along some remote road, a young mother, with a child on the cycle bar and, produce on the carrier. She could also be seen carrying two or even three pots of water hung across the back, and cycling towards work or home.

Finally, the author opines that for these neo-literate/neo-cyclist women, more than the economic aspect, the sense of self-respect it brings is vital. The author admits in a confessing tone that never before had he seen that humble vehicle (cycle) in that light – the bicycle as a metaphor for freedom. Before concluding, the writer quotes Kannammal who opines that for rural women it is a Himalayan achievement like flying an aeroplane.

In the last paragraph, the author adds a postscript. He says that in April 1995, when the author returned to Pudukkottai, the craze for learning cycling was still on (three years later). Then he adds that a large number of women were unable to afford bicycles which then cost around Rs. 1400 each. He concludes saying that Pudukkottai remains unique among Indian districts for the stunning proportion of women who have taken to cycling and the enthusiasm for gaining the skill among the rest.

Where there is a Wheel Summary in Kannada

2nd PUC English Textbook Answers Springs Chapter 13 Where there is a Wheel image - 2

Glossary:

neo-literates: new literates
gramsevikas: women social workers in villages
phenomenon: a fact or an event in nature or society
brainchild: an idea or invention of one person
non-chalance: indifference; unmoved

The main aim is to share the knowledge and help the students of 2nd PUC to secure the best score in their final exams. Use the concepts of Karnataka 2nd PUC English Answers Chapter 13 Where there is a Wheel in Real time to enhance your skills. If you have any doubts you can post your comments in the comment section, We will clarify your doubts as soon as possible without any delay.

2nd PUC English Textbook Answers Springs Chapter 11 Japan and Brazil through a Traveller’s Eye

October 28, 2023

Students who are in search of Japan and Brazil through a Traveller’s Eye Questions and Answers Pdf, Notes, Summary. First check in which chapter you are lagging and then Download Karnataka Board 2nd PUC English Textbook Answers Chapter Wise. Students can build self confidence by solving the Answers with the help of Karnataka State Board 2nd PUC. English is the scoring subject if you improve your grammar skills. Because most of the students will lose marks by writing grammar mistakes. So, we suggest you to Download Karnataka State Board 2nd PUC English Answers according to the chapters.

Karnataka 2nd PUC English Textbook Answers Springs Chapter 11 Japan and Brazil through a Traveller’s Eye

Check out the topics covered in 2nd PUC before you start your preparation. Improve your grammar skills with the help of Karnataka 2nd PUC English pdf links. The Answers for Karnataka 2nd PUC English Textbook Springs Chapter 11 Japan and Brazil through a Traveller’s Eye are prepared by the English experts. So, if you follow Karnataka 2nd PUC English Textbook Answers you can cover all the topics in 2nd PUC Chapter 11 Japan and Brazil through a Traveller’s Eye. This helps to improve your communication skills.

Japan and Brazil through a Traveller’s Eye Questions and Answers, Notes, Summary

Japan and Brazil through a Traveller’s Eye Comprehension I

Japan And Brazil Through A Traveler’s Eye KSEEB Solution Question 1.
‘Exquisitely well-mannered people’ refers to
a. Indians
b. Japanese
c. Americans.
Answer:
(b) Japanese.

Japan And Brazil Through A Traveler’s Eye Notes KSEEB Solution Question 2.
What behaviour substitutes privacy in Japan?
Answer:
The respect for one another’s privacy and showing courtesy are the substitutes for privacy in Japan.

Japan And Brazil Through A Traveler’s Eye Summary KSEEB Solution Question 3.
The reference to public telephone suggests:
a. how overcrowded Japan is
b. how the Japanese respect privacy
c. how busy the Japanese are.
Answer:
(b) how the Japanese respect privacy.

Japan And Brazil Notes KSEEB Solution Question 4.
Hierarchy in bowing demands
a. youngsters bow to their elders.
b. wife bow to her husband.
c. sisters bow to their brothers.
Answer:
(b) and (c) wife bow to her husband/ sisters bow to their brothers.

Japan And Brazil Through A Traveler’s Eye Textbook Pdf KSEEB Solution Question 5.
How does one show appreciation while eating soup in Japan?
OR
What is the sign of appreciation when eating soup in Japan?
OR
Why do the Japanese make a fearful noise while eating soup?
Answer:
When a person is eating soup offered by a host or hostess, he must make a fearful noise so as to show his sign of appreciation; otherwise, the host or hostess will think that the guest is ill-mannered.

2nd Puc English Notes Japan And Brazil KSEEB Solution Question 6.
How are the pavements in Brazil decorated? What does it tell us about the people there?
OR
Why do Brazilians decorate the pavements they walk on?
Answer:
The grey pavements in Brazil are often decorated with beautiful black mosaics, a unique type of decoration. From this, we can infer that these people are alive to beauty in their surroundings. Secondly, they walk very slowly and meditatively and have a lot of time for contemplation.

2nd Puc English Japan And Brazil Through A Travelers Eye KSEEB Solution Question 7.
What happens when leisurely people in Brazil get a steering wheel in their hands?
Answer:
When leisurely people get a steering wheel in their hands, they drive their vehicles so fast that you would be inclined to believe that gaining a tenth of a second is a matter of grave importance for them all the time.

Japan And Brazil Through A Traveller’s Eye KSEEB Solution Question 8.
Who do the Brazilian drivers look out for when they are driving? Why?
Answer:
The drivers are supposed to look out for pedestrians. But in Brazil, they do not care for people walking on the roads or people trying to cross the roads. The deliberately accelerate and laugh at pedestrians who run to survive. The drivers in Brazil treat pedestrians as fair prey to hunt and mow down.

Question 9.
What remarkable attitude is seen in the war between drivers?
Answer:
The war between the drivers is murderous but good-tempered. They cut in, overtake on both sides and force the other person to break violently and commit all the most heinous crimes. But they smile at the other person without any anger, hostility, or mad hooting.

Japan and Brazil through a Traveller’s Eye Comprehension II

Question 1.
Why is bowing in Japan a complicated process?
Answer:
‘Bowing’ in Japan is complicated because they follow a complicated hierarchy in bowing which they have to follow as sacrosanct. The Japanese know very clearly who should bow to whom, how deeply, and for how long. For example, they follow certain basic rules inside the family. They are: “the wife bows to her husband, the child bows to his father, younger brothers to elder brothers, and the sister bows to all brothers”. The Japanese reflect the smallest difference in rank, standing, age, social position in their bowing style in that split second.

Question 2.
Why does bowing, a natural practice in Japanese culture, look so quaint’ and puzzling to the author?
Answer:
‘Bowing’, a natural practice in Japanese culture, looks so ‘quaint’ and puzzling to the author because when the Japanese bow, they bow neither too deeply nor not deeply enough and bow to the right person at the right time. They know who should bow to whom, how deeply, and for how long. They manage it without difficulty and subtly reflect even the smallest difference in rank, standing, age, and social position. Secondly, they bow with the ceremonious serenity of a courtier yet with a great deal of natural and inimitable grace.

Question 3.
Do you think the author is finding fault with/making fun of the culture of bowing in Japanese and speeding cars in Brazil?
Answer:
No. This article is a piece of travel writing. Travel writing is no longer viewed as a product of some innocent curiosity. It is also not an attempt to understand an alien culture objectively. Rather, this narrative should be read as the author’s perspective on Japanese culture. The author is certainly not finding fault with the Japanese way of eating soup or their habit of bowing.

The author is only expressing his surprise when he looks at their cultural habits as an outsider. The language might sometimes appear to be used for a humorous effect. But, in a piece of creative writing, such liberties in their style must be accepted as natural. If the writer simply described objectively whatever he saw, the article will lose its human interest.

Similarly, the author is expressing his surprise at the way the drivers of four-wheelers move on the road in Brazil and the size of the fast-moving traffic. The author is also expressing his appreciation for the beautiful black mosaics seen on the pavements. He is also expressing his annoyance for the sluggishness of the leisurely walking pedestrians.

Japan and Brazil through a Traveller’s Eye Comprehension III

Question 1.
‘Bowing in Japan is quainter; more formal, more oriental.’ Do you agree?
OR
How does George Mikes describe bowing to be a quainter and infectious trait of Japanese people?
Answer:
Yes. In this article, the author introduces the reader to one of the most fascinating and conspicuous cultural habits of the Japanese people. He tells the reader that as soon as you land in Japan, you perceive immediately that the Japanese are exquisitely well-mannered. Very soon, you will also discover that the Japanese are very courteous and ensure that they do not violate a speaker’s privacy while talking to someone over the phone. Then you come to be a witness to people bowing to each other almost everywhere as if it is an obsession with them.

However, the author records his appreciation for their skill and style of bowing. He says that people bow to each other with the ceremonious solemnity of a courtier and yet with a great deal of natural and inimitable grace. Then he remarks that bowing is neither less nor more silly than shaking hands or kissing the cheek, but it is quainter, more formal, more oriental, and also infectious.

He says so because, while anyone can learn the art of shaking hands or kissing the cheek perfectly well, it is extremely difficult for a European to learn to do ‘bowing’ the way Japanese do because, in a split second, the Japanese manage to subtly reflect all the nuances one needs to follow while bowing. They successfully exhibit the smallest difference in rank, standing, age, and social position. On the other hand, if European attempts to bow to someone, he or she will bow too deeply or not deeply enough; they bow to the wrong man at the wrong time or they do not clasp their hands in front of them which is bad or they do in a wrong way which is considered even worse.

Question 2.
Describe how traffic in Brazil leads to humorous observations.
OR
Give an account of the crawling traffic in Brazil as mentioned by George Mikes.
OR
Write a note on traffic in Brazil.
Answer:
George Mikes makes humorous comments on the ‘traffic’ in Copacabana and Avenida Presidente Vargas in particular and Brazil in general. He opines that Brazilians are easy-going and leisurely characters. But the very same people, the moment they get a steering wheel in their hands, no speed „ is fast for them. They drive with such speed that one would be inclined to believe that gaining a tenth of a second is a matter of grave importance for all of them, all the time. The writer talks about the increasing number of vehicles in Brazil and says that the increase in the number of vehicles is making the pedestrian’s life more hazardous every day. He then narrates an interesting anecdote to give a clear idea of the number of vehicles moving on the road at any given time in Avenida Presidente Vargas.

He asks the reader to imagine that he is standing on one side of the road trying to cross the road. He will spend hours on end contemplating a fascinating problem: How can crawling traffic proceed at such a terrifying speed? He strengthens the same idea by another example. He asks the reader to imagine that a man on his side of the road suddenly catches sight of a friend of his on the other side of the road and starts waving to him. Then he shouts at him asking “How on earth did you get over there?” The other person will yell back at him, “How? I was born on this side!” The author leaves it to the readers to draw their own inferences.

Question 3.
What aspects of our social life, do you think, would appear quaint and odd to a foreign tourist?
Answer:
India is a multi-ethnic, pluricultural, and multilingual country. People of different cultural practices, customs, and traditions are spread throughout the length and breadth of the country, thus making the population heterogeneous in character.
But, foreign tourists who visit India, most often come from countries where the population is homogeneous in character. Naturally, the foreign tourists find quite a few aspects of our social life, quaint and odd.

One of the commonest habits that might appear quaint to them is the way our womenfolk create patterns on the floor in front of their houses or gates, with chalk piece, coloured powder, or flower petals, called Rangoli.

Similarly, the buntings of mango leaves and plantain tree stem that we decorate our houses with special pooja days, auspicious occasions and festivals, and lighting lamps during Deepavali appear odd to them. Secondly, Indians’ love and respect for the holy cow and monkeys as a symbol of god also appear quaint to them.

Another aspect that may appear quaint is the ritual of worshipping vehicles which are commonly seen during Ayudha Pooja. Apart from these, there are several habits which might make us bow our heads in shame. They are urinating in public, spitting chewed betel nut residues on the walls and roads,’ sneaking loudly over mobile phones in public places, jumping at railway and bus ticket counters and at bus stops, crossing roads where there are no pedestrian crossings, honking unnecessarily, parking vehicles on the footpath, disobeying signal lights, men staring at women walking on the street till she moves out of sight, putting up pandals for private functions on the road and blocking it for pedestrians and motorists, shoving garbage on the road, pedestrians jaywalking on the road, etc. Talking to strangers on trains and buses, enquiring them about their jobs and salaries, etc., are also some of the social aspects of Indians which appear quaint to foreigners.

Japan and Brazil through a Traveller’s Eye Additional Questions and Answers

I. Answer the following questions in a word, a phrase, or a sentence each:

Question 1.
What does Mikes call, ‘A man’s castle’ in Japan?
Answer:
A man’s telephone receiver.

Question 2.
How long does it take in Japan to be convinced that you are among exquisitely well-mannered people?
Answer:
Only a quarter of an hour.

Question 3.
Whom do the Japanese stores employ to welcome customers?
Answer:
Bowing girls.

Question 4.
Whatis the duty of the bowing girls in Japanese stores?
Answer:
The bowing girls have to bow deeply and deferentially to all and sundry that visit the Japanese stores.

Question 5.
Which places does the Tokaido Line connect?
Answer:
Tokyo and Osaka.

Question 6.
Where did the writer Mikes meetadeerin Japan?
Answer:
The writer met a deer in one of the parts of Nara, which is a wild deer park in Japan.

Question 7.
What are the pavements in the streets of Copacabana decorated with?
Answer:
With beautiful black mosaics.

Question 8.
What kind of people would take the trouble to decorate the pavements they walk on?
OR
Who, according to the writer, would take the trouble to decorate the pavements they walk on?
Answer:
Only a people alive to beauty in their surroundings and who have plenty of time for contemplation during their meditative walking would take the trouble to decorate the pavements they walk on.

Question 9.
Why are motor cars expensive in Brazil?
OR
What is extremely expensive in Brazil?
Answer:
Motor cars are extremely expensive in Brazil because import duties are crippling and murderous.

Question 10.
Why is the pedestrian’s life in Brazil becoming more hazardous every day?
Answer:
The pedestrian’s life in Brazil is becoming more hazardous every day because the number of motor vehicles is growing by leaps and bounds.

Question 11.
Which place in Brazil does the writer consider as the worst for pedestrians?
Answer:
The author considers Avenida Presidente Vargas as the worst place in Brazil for pedestrians.

Question 12.
Which habit of the Japanese is referred to as mania by George Mikes?
OR
What does the Japanese mania refer to, according to Mikes?
Answer:
George Mikes refers to the Japanese habit of bowing’ as mania.

Question 13.
Which place in Brazil is the worst with regard to traffic, according to George Mikes?
Answer:
Avenida Presidente Vargas, known for its terrifying speed of traffic on the road, is the worst place of all in Brazil.

Question 14.
Whose life is becoming more hazardous in Brazil every day, according to George Mikes?
Answer:
According to George Mikes, the pedestrian’s life is becoming more hazardous in Brazil every day.

Question 15.
What, according to George Mikes, do the driver and pedestrian finally do after the chase in Brazil?
Answer:
According to George Mikes, the pedestrian does not resent being chased by the driver. Both of them smile amicably at each other.

Question 16.
What does the speaker compare Japanese bowing to?
Answer:
The speaker compares Japanese bowing to the ceremonious solemnity of a courtier.

Question 17.
Who are the drivers in Brazil on the lookout for?
Answer:
The drivers in Brazil are on the lookout for any pedestrians stepping off the pavement, who they regard as a fair game.

Question 18.
Which animal created the impression that it bowed to George Mikes in Japan?
OR
Which animal bowed to the author at Nara?
Answer:
At Nara in Japan, a deer created the impression that it bowed to the author.

Question 19.
What did the deer do after bowing to Mikes at Nara?
Answer:
After bowing to the author at Nara, the deer jumped at him and snatched the little food-bag from his hand.

Question 20.
Mikes describes Japan as being
(a) overconfident
(b) overcrowded
(c) overjoyed.
Answer:
(b) overcrowded.

Question 21.
An hour in Japan convinced Mikes that he was among
(a) leisurely characters
(b) ill-mannered louts
(c) well-mannered people.
Answer:
(c) well-mannered people.

Question 22.
What do the people of Japan highly respect, according to Mikes?
Answer:
According to Mikes, the people of Japan highly respect one another’s privacy.

Question 23.
As Mikes says, after a few hours in Japan, one starts _______
(a) thanking
(b) bowing
(c) kissing
Answer:
(b) bowing.

Question 24.
Who bows to all brothers in a Japanese family, according to Mikes?
Answer:
According to Mikes, the sister bows to all brothers in a Japanese family.

Question 25.
What do mothers in Japan carry their babies in, according to Mikes?
Answer:
According to Mikes, mothers carry their babies in little saddles.

Question 26.
The Japanese stores employ ______ to greet customers.
(a) singing girls
(b) dancing girls
(c) bowing girls
Answer:
(c) bowing girls.

Question 27.
Who enters the carriage in a slightly theatrical scene as noticed by Mikes in Japan?
Answer:
According to Mikes, two conductors enter the carriage in a slightly theatrical scene.

Question 28.
Which animal bowed to Mikes in Japan?
Answer:
A deer bowed to Mikes in Japan.

Question 29.
Who is transformed into savages as soon as a bus arrives in Japan?
Answer:
As soon as a bus arrives in Japan, the bowing gentlemen are transformed into savages.

Question 30.
George Mikes compares the act of two Japanese bowing to
(a) an early American traffic law
(b) page-boys turning revolving doors
(c) Tokaido line that connects Tokyo and Osaka.
Answer:
(a) an early American traffic law.

Question 31.
What did the deer snatch from Mikes’hand in Japan?
Answer:
The deer snatched the little food-bag from Mikes’ hand.

Question 32.
In Japan, as soon as the bus arrives, the bowing gentlemen are transformed into
(a) savages
(b) slaves
(c) servants.
Answer:
(a) savages.

Question 33.
What must one do while eating soup in Japan, according to Mikes?
Answer:
According to Mikes, while eating soup one must make a fearful noise.

Question 34.
In Japan, eating soup by making a fearful noise is a sign of
(a) depreciation
(b) appreciation
(c) disregard.
Answer:
(b) appreciation.

Question 35.
Who enters the carriage on the Tokaido line in a slightly theatrical scene in Japan?
Answer:
Two conductors enter the carriage on the Tokaido line, in a slightly theatrical scene.

Question 36.
According to Mikes, bowing girls in Japan are equal to
(a) page-boys
(b) maidservants
(c) security guards.
Answer:
(a) page-boys.

Question 37.
The pavements in the streets of Copacabana in Brazil are often decorated with
(a) pink granite slabs
(b) grey cobblestones
(c) beautiful black mosaics.
Answer:
(c) beautiful black mosaics.

Question 38.
As soon as a driver notices a pedestrian step off the pavement in Brazil, he
(a) regards him as a fair game.
(b) greets him and smiles.
(c) ignores him and moves on.
Answer:
(a) regards him as a fair game.

Question 39.
‘The Avenida Presidents Vargas’ in Brazil is described by Mikes as
(a) a wonderful place.
(b) an auspicious place.
(c) the worst place.
Answer:
(c) the worst place.

Question 40.
When do the drivers of the motor cars in Brazil aim and accelerate?
Answer:
The moment a motor car driver in Brazil notices a pedestrian stepping off the pavement, he regards the pedestrian as fair game, takes aim, and accelerates his car.

II. Answer the following questions in a paragraph of 80 – 100 words each:

Question 1.
What makes Mikes feel that the drivers in Brazil are on the lookout for pedestrians
Answer:
George Mikes feels that the drivers in Brazil are on the lookout for pedestrians because as soon as a driver notices a pedestrian step off the pavement, he appears to regard him as a fair game and so he takes aim and accelerates his vehicle. The pedestrian has to jump, leap, and run for his/her dear life.

Question 2.
‘People respect each other’s privacy’. Explain with reference to Japan in Mikes’ travel writing.
OR
Explain how the people of Japan respect each other’s privacy, according to George Mikes.
Answer:
The people of Japan live on a hopelessly overcrowded island where they have no privacy. However, the people are so well-mannered that they are courteous enough not to overhear a conversation when they find anyone talking to someone else over the telephone. The speaker can consider the telephone receiver as his castle and conduct his most confidential business transactions and intimate love-quarrels in public, yet in perfect privacy without becoming apprehensive about anyone overhearing his conversation.

Question 3.
How does the writer explain the complicated hierarchy in bowing?
OR
Why is bowing in Japan a complicated process?
OR
“The Japanese follow a complicated hierarchy in bowing.” Explain with reference to ‘Japanese Manners’.
Answer:
The writer George Mikes remarks that for the Japanese people ‘bowing’ has become a mania. However, he also speaks in an appreciative tone and says that the people bow to each other with the solemnity of a courtier yet with a great deal of natural and inimitable grace. Besides, he also says that the Japanese follow a complicated hierarchy in bowing. This system decides who bows to whom, how deeply, and for how long. Though it is a little complicated to us, the Japanese manage it without difficulty and subtly and reflect in their bowing even the smallest difference in rank, standing, age, and social position in a split second.

Question 4.
How, according to George Mikes, do the bowing gentlemen turn into savages in Japan?
Answer:
The author George Mikes, having described in detail how ‘bowing’ is followed so ritualistically in Japan, towards the end of the article narrates an incident that happened in one of the parts in Nara, a deer park in Japan. The author bought a pack of food for a deer. On seeing the pack of food, the deer went up to him, looked into his eyes, and bowed to him deeply. Then, almost immediately, it jumped at him and snatched the little food-bag from his hand.

Using this incident as an analogy, George Mikes makes fun of the Japanese people, for their ugly behaviour while boarding a bus. He tells the reader in a sarcastic tone that we can often see the Japanese bowing to each other with ceremonious serenity even at bus-stops. Then he says, “as soon as the bus arrives, the bowing gentlemen are transformed into savages, they push each other aside, tread on each other’s toes and shove their elbows into each other’s stomachs”. The reader, who had all along been encouraged to develop a kind of admiration for the Japanese habit of respectful bowing, is shocked or stunned by this revelation.

Question 5.
Give an account of the Japanese mania for bowing as described by George Mikes.
OR
What is unique about bowing in Japan?
OR
What are the views of George Mikes about Japanese ‘mannerism of bowing’?
Answer:
According to George Mikes, as soon as we land in Japan, the first thing we notice is bowing is so ubiquitous in Japan. The writer comments that it is the mania of the Japanese. He remarks that everyone keeps bowing to everybody else with the ceremonious solemnity of a courtier yet with a great deal of natural and inimitable grace. If two Japanese bow, as a rule, neither is to straighten up before the other stands erect in front of him. He states that bowing is quainter, more formal, and more oriental and also infectious.

Besides, he also says that the Japanese follow a complicated hierarchy in bowing. This system decides who bows to whom, how deeply, and for how long. Though it is a little complicated to us, the Japanese manage it without difficulty and subtly and reflect in their bowing even the smallest difference in rank, standing, age, and social position in a split second.

Apart from saying that the Japanese follow a complicated hierarchy in their bowing, the author says that the Japanese follow certain basic rules inside the family. They are the wife bows to her husband, the child bows to his father, younger brothers to elder brothers, and the sister bows to all brothers of whatever age.

In Japanese stores, bowing girls stand at the top of escalators and their only duty is to bow deeply and deferentially to all and sundry.

The ticket checking conductors on the fast Tokaido Line, march to the middle of the coach and bow ceremoniously in both directions before checking the tickets.

At Nara, a deer created the impression that it bowed to the author.

Question 6.
According to George Mikes, the people of Brazil are both leisurely and speed-loving. Explain.
Answer:
According to George Mikes, the people of Brazil are both leisurely and speed-loving. He remarks that however close by or far off their destination may be, Brazilians do not seem to bother about the time it might take for them to reach their destination. They do not hurry at all; they do not mind even if they reach their destination either an hour too soon or a day late or may not reach at all. But the very same leisurely people, as soon as they get a steering wheel in their hands, no speed is fast enough for them. If one looks at their driving speed, one would be inclined to believe that gaining a tenth of a second is a matter of grave importance for all of them all the time.

Question 7.
Why does George Mikes say that eating soup has more dangers for a European?
Answer:
The author George Mikes concludes his observations with his comments on the Japanese way of eating soup. He remarks that eating soup has more dangers than almost anything else. He opines so because eating soup in a Japanese house puts an outsider in a dilemmatic situation. The Japanese host expects the ‘guest’ to make a fearful noise to Show his sign of appreciation while eating soup. If the guest is a European and if he or she does not make a fearful noise, then the host will think that their guest is an ill-mannered lout. But, having knowledge of this custom, if a European visitor makes a fearful noise while eating soup to express his appreciation, then the host will think that he must be an ill-mannered lout because the Japanese know that no reasonably well brought up European makes such disgusting noises when eating soup.

Question 8.
Bring out the culture of the Japanese as explained by George Mikes.
Answer:
In his travelogue, George Mikes narrates four anecdotes which will help any foreign visitor to understand the cultural traits of the Japanese people. The author first highlights how people’s courtesy serves a double function in Japan. He assertively states that a couple, with perfect confidence, can carry on even their intimate love quarrels in public, in perfect privacy, without being apprehensive of any passerby overhearing them. Next, he talks about the bowing mania of the Japanese people and how the Japanese manage to show even the slightest differences in their hierarchy with a great deal of natural and inimitable grace.

Then, he narrates how, the very same people who, a few minutes ago had bowed to each other with such ceremonious solemnity would behave like savages, push each other aside, tread on each other’s toes and elbow their way into the bus. Finally, he talks about soup-eating in Japan. He says that, according to the Japanese, when eating soup one must make a fearful noise so as to express his appreciation, otherwise the guest will be considered an ill-mannered lout.

Question 9.
Why is a pedestrian’s life hazardous in Brazil, according to George Mikes?
OR
Elaborate on the plight of a pedestrian in Brazil.
Answer:
According to George Mikes, the people of Brazil are both leisurely and speed-loving. Then he says that the people love driving their cars at such speed that as soon as the drivers get a steering wheel in their hands, no speed is fast enough for them. They seem to believe that gaining a tenth of a second is a matter of grave importance for them all the time. The drivers usually lookout for pedestrians who step off the pavement and regard such pedestrians as a fair game. They take aim and accelerate their vehicle. The pedestrians have to jump, leap, and run for their dear life. Naturally, on account of such speed-loving people, a pedestrian’s life is hazardous in Brazil.

Question 10.
Explain how drivers in Brazil care about pedestrians, as mentioned by George Mikes.
Answer:
According to George Mikes, the people of Brazil are both leisurely and speed-loving. Then he says that the people love driving their cars at such speed that as soon as the drivers get a steering wheel in their hands, no speed is fast enough for them. They seem to believe that gaining a tenth of a second is a matter of grave importance for them all the time. The drivers usually lookout for pedestrians who step off the pavement and regard such pedestrians as a fair game. They take aim and accelerate their vehicle. The pedestrians have to jump, leap, and run for their dear life. Naturally, on account of such speed-loving people, a pedestrian’s life is hazardous in Brazil.

Question 11.
How do Mikes portray a view of Japanese culture through their act of bowing?
Answer:
According to George Mikes, as soon as we land in Japan, the first thing we notice is the mania of bowing, which is so ubiquitous in Japan. The writer comments that it is the mania of the Japanese. He remarks that everyone keeps bowing to everybody else with the ceremonious solemnity of a courtier yet with a great deal of natural and inimitable grace. If two Japanese bow, as a rule neither is to straighten up before the other stands erect in front of him. He states that bowing is quainter, more formal, and more oriental and also infectious.

Further, he says that the Japanese follow a complicated hierarchy in bowing. This system decides who bows to whom, how deeply, and for how long. Though it is a little complicated to us, the Japanese manage it without difficulty and subtly and reflect in their bowing even the smallest difference in rank, standing, age, and social position in a split second. Apart from saying that the Japanese follow a complicated hierarchy in their bowing, the author says that the Japanese follow certain basic rules inside the family. They are the wife bows to her husband, the child bows to his father, younger brothers to elder brothers, and the sister bows to all brothers of whatever age.

Question 12.
Why does George Mikes say that nobody hurries in Brazil? What instances does he give to illustrate this?
Answer:
While recording his observations about the paradoxical behaviour of the people in time management, George Mikes says that nobody hurries in Brazil and does not seem to be worried whether they reach their destination an hour too soon, a day late, or not at all. Though his statement appears to be an exaggeration, George Mikes then tells us the reason. His inference seems to be based on his imaginative assessment of the probable time a pedestrian in Copacabana takes to move from one end of a street to the next while walking on the pavement, enjoying the beauty of the black mosaics on the pavements. George Mikes says that the natives seem to relish such beautiful decorations on the pavements and do not mind spending time meditating on the beautiful mosaics while walking on the pavements.

He then cites another instance which highlights the incredible size of the crawling traffic in Avenida Presidente Vargas. Imagining that a pedestrian standing on one side of the street, asks his friend on the other side, how he got there, his friend would reply that he was born on that side and he did not go there crossing the street. This imaginary incident would tell the reader about the size of the moving traffic in Brazil.

III. Answer the following questions in about 200 words each:

Question 1.
How does the writer bring out the dilemma of crossing the road in Avenida Presidente Vargas?
OR
How does the writer bring out the humour in crossing the road in Avenida Presidente
Vargas?
OR
Why is it difficult to cross a road in Brazil? How is this brought out in ‘Traffic in Brazil’?
OR
Describe the situation, one witness, while trying to cross the road in Brazil, as mentioned by George Mikes.
Answer:
While recording his observations about the traffic in Brazil, the author comments about the reckless driving style of the Brazilian people. Then he expresses his surprise over the growing number of motor cars in Brazil, despite the fact that import duties charged by the government for importing cars are crippling and murderous, then he gives the reader an anecdote to help him visualize to himself the number of vehicles that are there and the way they move on the roads.

He tells the reader that one witness the worst traffic problems in Avenida Presidente Vargas. If a pedestrian were to stand on one side of the road, trying to cross the road and contemplating the truly fascinating problem, ‘How can crawling traffic proceed at such terrifying speed?’, he will be spending hour after hour without a ray of hope of an auspicious crossing. Then he rounds up his anecdote telling us that, the pedestrian will witness a scene in which a man standing beside you, on your side of the Avenida Vargas, suddenly catches sight of a friend of his on the other side and starts waving to him. Then he will ask him, “How did you get there?” The other fellow being surprised by this naive question will yell back, “How? I was born on this side!” One can easily infer the author’s intention in giving us this anecdote.

Question 2.
How does George Mikes bring out the humour in the Japanese mannerism of bowing?
OR
Bowing in Japan is so infectious that it leads to a few comic situations. How does Mikes bring this out?
Answer:
George Mikes narrates two very unique habits of the people noticed by every tourist in Japan. One of them is their mannerism of bowing. However, the author’s description is quite packed with humour as well as admiration. He calls the bowing habit of the Japanese a ‘mania’ and says “everybody keeps bowing to everyone else, with the ceremonious solemnity of a courtier yet with a great deal of natural and inimitable grace”. Interspersed in his description of their bowing, there are certain statements which make the description sound humorous in a subtle way. They are:

After a few hours you start bowing yourself; you bow to the wrong man at the wrong time; you do not clasp your hands in front of you which is bad, or you do which is worse.
If two Japanese bow, neither is to straighten up before the other stands erect in front of him.
saw babies carried in Japanese style on their mothers’ backs in clever little saddles, and whenever the mother bowed, the baby bowed too, somewhat condescendingly, from his majestic height.
Bowing girls in Japanese stores stand at the top of escalators and their only duty is to bow deeply and deferentially to all and sundry.
The ticket checking conductors on the fast Tokaido Line, march to the middle of the coach and bow ceremoniously in both directions before checking the tickets.
About the deer in ‘Nara’, the author comments, “it is something genetic and is in the blood of Japanese deer”.
At the bus-stop, “the bowing gentlemen are transformed into savages” as soon as a bus arrives.

Question 3.
Bowing in Japan is quainter, formal, and oriental. Why does George Mikes say so? Discuss.
Answer:
According to George Mikes, bowing is so ubiquitous in Japan, it is the mania of the Japanese.
Everyone keeps bowing to everybody else with the ceremonious solemnity of a courtier yet with a great deal of natural and inimitable grace. If two Japanese bow, as a rule, neither is to straighten up before the other stands erect in front of him. He states that bowing is quainter, more formal, and more oriental and also infectious.

Apart from saying that the Japanese follow a complicated hierarchy in their bowing, the author says that the Japanese follow certain basic rules inside the family. They are the wife bows to her husband, the child bows to his father, younger brothers to elder brother stand the sister bows to all brothers of whatever age.

Question 4.
A natural behaviour looks peculiar when seen from an outsider% eyes. How does Mikes prove this with reference to Japanese bowing?
Answer:
The excerpt titled ‘Japanese Manners’ is a good specimen of travel writing, by George Mikes, a Hungarian-born British travel writer. In this excerpt, the author shares his experience of travelling in Japan and tries to draw the readers’ attention to what is most striking about the Japanese people as seen by a touring journalist.

Though bowing to their fellowmen in Japanese society is a normal trait of Japanese behaviour, to an outsider like George Mikes, ‘bowing’ appears to be an obsession with the Japanese. That is why George Mikes chooses to comment about the bowing patterns of the Japanese in this piece of travel writing.

According to George Mikes, as soon as we land in Japan, the first thing we notice is bowing is so ubiquitous in Japan. The writer comments that it is the mania of the Japanese. He remarks that everyone keeps bowing to everybody else with the ceremonious solemnity of a courtier yet with a great deal of natural and inimitable grace. If two Japanese bow, as a rule, neither is to straighten up before the other stands erect in front of him. He states that bowing is quainter, more formal, and more oriental and also infectious.

Question 5.
George Mikes brings out the contrast between leisurely characters and dangerous drivers in Brazil. Explain.
Answer:
George Mikes is a keen observer of people’s behaviour, customs, and traditions. In the excerpt titled Traffic in Brazil’, Mikes says very casually that nobody hurries in Brazil and remarks that it does not really matter whether you reach your destination an hour too soon, a day late, or not at all. Next, probably with the intention of finding a suitable explanation for the leisurely attitude of the people, he tells the readers about the ‘grey pavements’ in the streets of Copacabana, which are decorated with beautiful mosaics. Then he adds that ‘only a people alive to beauty in their surroundings and who have plenty of time for contemplations during their meditative, ambulatory exercises would take the trouble to decorate the pavements they walk on. Though Mikes is appreciating the people for their aesthetic sensibility, he is also satirizing the lethargic walking style and attitude of the people.

However, in the very next sentence, he makes fun of dangerous drivers in Copacabana. Mikes remarks that the very same leisurely characters, when they get behind a steering wheel, drive very fast and are reckless. Having made a comment about their time consciousness, now, he says, “gaining a tenth of a second is a matter of grave importance for all of them all the time”. Thus, by juxtaposing two different traits of these people, George Mikes brings out the contrast between leisurely characters and dangerous drivers in Brazil.

Question 6.
People display contrasting patterns of behaviour. Substantiate in the light of’Japan and Brazil Through A Traveller’s Eye’.
Answer:
In this lesson, there are two excerpts titled ‘Japanese Manners’ and ‘Traffic in Brazil’ chosen from George Mikes’ book titled ‘The Land of the Rising Yen’ and ‘How to Tango’ respectively.

In the first excerpt, the writer gives his comments about the Japanese habit of ‘bowing’ and how they manage to respect each others’ privacy even though they are living in an overcrowded island.

In the second excerpt, the author tells us in a humorous vein about their time consciousness and their craze for driving cars recklessly.

However, he makes his travelogue memorable by highlighting the contrasting patterns of behaviour of the people of Japan as well as the people of Copacabana in Brazil.

While describing the Japanese, George Mikes tells the reader that within fifteen minutes after a tourist has landed in Japan, he or she will come to the conclusion that the people of Japan are an exquisitely well-mannered people, who live on a hopelessly overcrowded island. Then, he justifies his opinion by telling the reader how the people respect each others’ privacy by being courteous enough not to overhear a telephone conversation even though they happen to pass by a telephone booth or a counter. Next, he describes the ‘bowing’ mania of the Japanese people. Though he describes their bowing patterns in an appreciative tone, he concludes his write up highlighting a contrasting behavioural trait of the Japanese.

In a humorous tone, he tells the reader that the Japanese people who bow with such ceremonious serenity even at bus-stops, exhibit flippant behaviour almost immediately. He tells the reader that as soon as a bus arrives, the bowing gentlemen become savage-like, push each other aside, tread on each other’s toes and shove their elbows into each other’s stomachs to get into the bus.

Similarly, in his travel write up titled ‘Traffic in Brazil’, while narrating his experiences as a tourist walking through the streets of Copacabana, he comments about the time consciousness of the local people. He says very casually, “Nobody hurries in Brazil”, then he adds “it does not really matter whether you reach your destination an hour too soon, a day late, or not at all”. Then he narrates how the people decorate the grey pavements in the streets with beautiful black mosaics. Then referring to their walking style he says, ‘Only a people alive to beauty in their surroundings and who have plenty of time for contemplation during their meditative, ambulatory exercises would take the trouble to decorate the pavements they walk on.

We should note that though he appears to be appreciating the aesthetic sense of the people, there is also a tone of satirising the sluggish walking style, or the lethargic attitude of the people.

Almost immediately he juxtaposes a contrasting pattern of their behaviour. He tells the reader that the very same leisurely characters when they get behind a steering wheel, drive very fast, and are reckless. He says, “gaining a tenth of a second is a matter of grave importance for all of them all the time”. The reader cannot but infer that the people of Copacabana are very lethargic only while walking but are reckless while driving a motor car. Thus, in both the essays we find George Mikes highlighting contrasting patterns of behaviour of the people.

Japan and Brazil through a Traveller’s Eye Vocabulary

Synonyms are words with the same or similar meanings.
Provide Synonyms for the following words from the lesson. You may consult a dictionary:

Intimate – personal, private
Quaint – strange, unusual, odd
Majestic – royal, kingly, princely
Deferential – respectful
Solemn – courtly, majestic, stately, dignified
Amicably – courteously, cordially
Mystify – puzzle
Murderous – brutal, fierce, cruel, inhuman
Auspicious – favourable
Hostility – bitterness, grudge
Expensive – costly, dear
Savages – uncivilized, barbarous
Accelerate – speed up, quicken
Import – bring in, ship in
Complicated – complex.

Question 1.
Note the use of the following expressions in the travelogue.

all and sundry
cut in
by leaps and bounds
listen in
fair game
get into
lookout
for dear life
be one’s castle
clear cut
easygoing.

The meaning of each expression is given below. Match the expression with its meaning of looking at the context in which it is used
(a) definite to see or identify
(b) relaxed and happy to accept things
(c) everyone
(d) to move suddenly in front of another vehicle
(e) a place where one can be private and safe
(f) very quickly; in large amounts
(g) as hard or as fast as possible
(h) to listen to a conversation that you are not supposed to hear
(i) to develop a particular habit
(j) someone or something that should be allowed to be criticized
(k) to keep trying to find something or meet somebody.
Answer:
a – 10, b – 11, c – 1, d – 2, e – 9, f – 3, g – 8, h – 4, i – 6, j – 5, k – 7.

Additional Exercises

A. Passive Voice:

Question 1.
Privacy had a double function in Japan. Mikes ______ (surprise) to notice the Japanese attitude towards privacy. Though telephones ______ (situate) in the open, confidential business transactions ______ (conduct) with ease.
Answer:
was surprised; were situated; were conducted.

Question 2.
Bowing in Japan was closely observed by Mikes. Bowing ______ (do) in an oriental and formal manner. One’s social rank and status ______ (reflect) when two persons bowed to each other. In many cases, there were clearcut rules and they _____ (observe) without difficulty.
Answer:
was done; were reflected; were observed.

Question 3.
Mikes visited a Japanese house. He _____ (offer) a bowl of soup. While eating soup it ______ (expect) that he should make a fearful noise. He knew that if Europeans made sounds, they _____ (considered) to be ill-mannered louts.
Answer:
was offered; was expected; were considered.

Question 4.
Drivers in Brazil were very dangerous. Once they ______ (seat) behind the steering wheel, no speed was fast enough for them. If a pedestrian stepping off the pavement ______ (notice), he _______ (regard) as fair game.
Answer:
were seated; was noticed; was regarded.

B. Fill in the blanks by choosing the appropriate expressions given in brackets:

Question 1.
In Brazil, the number of motor vehicles is growing by _______, almost as if cars were distributed free of charge to ______. (all and sundry, turn a deaf ear, leaps, and bounds)
Answer:
leaps and bounds; all and sundry.

Question 2.
In Brazil, the pedestrians are, in fact, on the _____ for drivers. As soon as a driver notices a pedestrian step off the pavement, he regards him as ______. (fair game, to get into, lookout)
Answer:
lookout; fair game.

Question 3.
The deer looked into Mikes’s eyes and bowed deeply. It was no ______. He thought that if they see people bowing all the time, they _______ the habit too. (get into, come up, chance gesture)
Answer:
chance gesture; get into.

Question 4.
The war between drivers is murderous but good-tempered. They ________, overtake on both sides and force you to brake violently. Therefore a pedestrian, while crossing a road, has to jump, leap and run for ______. (dear life, fair game, cut in)
Answer:
cut in; dear life.

C. Fill in the blanks with the appropriate linkers:

Question 1.
Japanese who live on a hopelessly overcrowded island have to respect one another’s privacy _____ rather, would have to _____ they had any privacy. _____ they don’t. ______ courtesy has a double function in Japan. (but, if, or, so)
Answer:
or; if; But; So.

Question 2.
Japanese are said to be well-mannered ______ they respect each other’s privacy. You can conduct your most confidential business transactions and love-quarrels in public ______ in perfect privacy. Anybody could listen in _______ nobody does. _____ a telephone-receiver is a man’s castle in Japan. (yet, therefore, but, because)
Answer:
because; yet; but; Therefore.

Question 3.
Nobody hurries in Brazil. The people of Brazil are leisurely ______ they seem to have all the time in the world ______ decorate the pavements they walk on. ______, the drivers in Brazil drive so fast _______ every tenth of a second is of grave importance. (in order to, and, however, as though)
Answer:
and; in order to; However; as though.

Question 4.
Bowing in Japan is not only quainter but infectious. ______ spending some time in Japan, one starts bowing ______ he or she has been there forever. ______ when a person bows, it is too deep or not too deep enough _____ the outsider is not familiar with the complexities of bowing. (as though, after, however, because)
Answer:
After; as though; However; because.

Question 5.
Motor cars are extremely expensive in Brazil ______ of crippling and murderous import duties. ______ almost everyone owns a car here. ______ the roads in Brazil have heavy traffic. ______ the pedestrian’s life is becoming more hazardous every day. (thus, hence, yet, because)
Answer:
because; Yet; Thus; Hence.

Japan and Brazil through a Traveller’s Eye by George Mikes About the Writer:

George Mikes (1912 – 1987) was a Hungarian-born British artist, author, publisher, illustrator, and journalist. He studied Law and received his doctorate at Budapest University. He is best known for his humorous commentaries on various countries.

His early books included ‘We Were There To Escape’ and ‘How to be an Alien’. Subsequent books dealt with (among others) Japan (‘The Land of the Rising Yen’), Israel (‘Milk and Honey, The Prophet Motive’), the U.S. (‘How to Scrape Skies’), and the United Nations (‘How to Unite Nations’), Australia (‘Boomerang’), the British again (‘How to be Inimitable, How to be Decadent’), and South America (‘How to Tango’). Other subjects include God (‘How to be God’), his cat (‘Tsi-Tsa’), wealth (‘How to be Poor’), or philosophy (‘How to be a Guru’). His autobiography was called ‘How to be Seventy’.

In this lesson, there are two excerpts titled ‘Japanese Manners’ and ‘Traffic in Brazil’, chosen from George Mikes’ book titled ‘The Land of the Rising Yen’ and ‘How to Tango’, respectively. They are two specimens of ‘Travel writing’. The writer is a travel journalist, who presents his observations about the people of Japan and Brazil in these two articles.

In the first excerpt titled ‘Japanese Manners,’ the writer gives his comments about the Japanese habit of ‘bowing’ and how they manage to respect each others’ privacy even though they are living in an overcrowded island. The author shares his experience of travelling in Japan and tries to draw the readers’ attention to what is most striking about the Japanese people as seen by a touring journalist.

Japan and Brazil through a Traveller’s Eye Summary in English

Japanese Manners

Within fifteen minutes after you have landed in Japan, you will learn that the people of Japan are an exquisitely well-mannered people, who live on a hopelessly overcrowded island. Consequently, their living space is very limited and so they do not have any privacy, yet the people respect people’s privacy in a different way. Their ‘courtesy’ serves a double function. They exhibit such polite behaviour that their ‘courtesy’ itself serves as a substitute for privacy. The writer supports his opinion-with as an example.

For example, he says, one finds red telephones in the streets, shops, halls of hotels, etc., and the instrument is placed on a table or a counter. They do not have space to spare for telephone booths. But, any person can conduct his most confidential business transactions, even intimate love quarrels in public and in perfect privacy, without being apprehensive about any passerby overhearing you. The author emphatically says that the person’s telephone receiver is his castle.

The writer then gives his observations about the Japanese obsession with ‘Bowing’. He calls it a ‘mania’ because everybody keeps bowing to everybody else. He remarks that the people bow to each other with the solemnity of a courtier with a great deal of natural and inimitable grace. He comments that though ‘bowing’ is like shaking hands or kissing the cheek, it is quainter, more formal, and more oriental but also infectious. Then he states that bowing is so commonly seen everywhere that even the onlookers start bowing though not the right way as the Japanese do. We bow too deeply or not deeply enough or we bow to the wrong man at the wrong time. Secondly, we do not clasp our hands in front of us, which is considered a bad way, or we may clasp the hands in a bad way which is considered even worse.

2nd PUC English Textbook Answers Springs Chapter 11 Japan and Brazil through a Traveller’s Eye image - 1

Next, the writer tells us that the Japanese have a complicated hierarchy in bowing: who bows to whom, how deeply, and for how long. Then the author cites an incident that happened in America. He tells us that in one of the American states, there was a traffic law which laid down that if two cars met at an intersection, neither was to move before the other had gone. The author uses this incident to tell us that, similarly in Japan, if two Japanese bows, neither are to straighten up before the other stands erect in front of him. Though it sounds a little complicated to us, the Japanese manage it without difficulty and even the smallest difference in rank, standing, age, social position will be subtly reflected in that split second; one man’s bow will be shorter than the others’. In many cases, there are clear-cut differences in position and no difficulties.

According to the Japanese culture, the wife bows to her husband, the child bows to his father, younger brother to elder brothers, the sister bows to all brothers of whatever age. The author then recollects a sight he had seen in Japan, that of Japanese mothers carrying their babies on their backs in little saddles and whenever their mother bowed, the babies bowed too. Then there are the bowing girls in Japanese stores standing at the top of escalators, bowing deeply and deferentially to everyone. Next, the writer narrates his experience on a fast train (Tokaido Line), between Tokyo and Osaka. He tells us that two conductors enter the carriage in a theatrical style, march to the middle of the coach, bow ceremoniously in both directions, and then start checking the tickets.

Later, he narrates how even an animal like the deer do ‘bowing’. He tells the reader that in one of the parts of ‘Nara’ (Nara Park is a vast wildlife park located in the city of Nara, Japan, at the foot of Mount Wakakusa, where wild deer roam about freely), he bought a pack of food for deer. The deer came up to him, looked into his eyes, and bowed deeply. The author states that it was not a chance gesture but it was a proper and courteous bow.

The author conjectures that the deer are more imitative, and having seen the people bowing all the time, probably they also get into the habit. Then he says it may be something genetic and is in the blood of Japanese deer. Finally, he ends the incident, saying that the deer, after bowing to him, jumped at him and snatched the little food-bag from his hand.

He ends his travelogue on Japan with his humorous observations about ‘soup eating’ in Japan. According to the Japanese, when eating soup you must make a fearful noise; only then will one be appreciated. If the soup eater does not make a noise, his hostess will think that the guest is an ill-mannered lout. On the other hand, if the guest makes some noise while eating soup, she will think that he is not a reasonably well brought up European because no reasonably well brought up European makes such disgusting noises when eating up the soup. The author tells jokingly that the hostess will conclude that he must be an ill-mannered lout.

Traffic in Brazil

This excerpt is taken from ‘How to Tango’, a humorous commentary on South America, by George Mikes. The author tells us in a humorous vein how the people of Brazil drive their motor vehicles. He also records his appreciation of the people’s talent for decorating their grey pavements.

The author narrates his experiences while walking as a tourist through the streets of Copacabana. The very first sentence is a comment about their time consciousness. He says very casually, “Nobody hurries in Brazil”; then he sarcastically adds, “it does not really matter whether you reach your destination an hour soon, a day late, or not at all”.

Then he turns his attention towards the grey pavements in Copacabana. He states that the grey pavements in the streets are often decorated with beautiful black mosaics which he calls ‘a unique type of decoration’. Then he gives the people of Brazil his compliments for their talent for doing such decorations. He remarks, “Only a people alive to beauty in their surroundings and who have plenty of time for contemplation during their meditative, ambulatory exercises would take the trouble to decorate the pavements they walk on”. He uses a pompous term ‘ambulatory exercises’ to refer to their walking style.

One should also note that though here he is appreciating the people for their aesthetic sense, he is also satirizing their sluggish walking style or the lethargic attitude of the people. In the very next sentence, he makes fun of their ‘driving style’. He tells the reader that the very same leisurely characters when they get behind a steering wheel, they drive very fast and are reckless. Having made a comment about their time consciousness, now he says, “gaining a tenth of a second is a matter of grave importance for all of them all the time”. The reader cannot but infer that the people of Copacabana are very lethargic only while walking and are reckless while driving vehicles.

The writer remarks that buying a motor car in Brazil is an extremely expensive event because import duty for importing cars from other countries is very high. In this context, he also compares Brazil with other countries in South America and says, “Only a few other, poorer South American states are in a worse position in this respect.” Then he remarks that “complaints are universal; hardly anyone can afford a car.” Having said this he proceeds to say that yet you find an unimaginably large number of motor cars here. Then he makes a satirical comment on the craze of the people for buying cars.

He says, “the number of motor vehicles is growing by leaps and bounds as if they were distributed free of charge to all and sundry.”The reader should be careful to note here that the author is also expressing his doubt or surprise at the capacity of the people to pay such huge import duties to buy a car.

Then he explains how reckless the people who drive motor vehicles are. He remarks that “itis, not that drivers do not care about pedestrians”; the trouble is “they are, in fact, on the lookout for them. As soon as a driver notices a pedestrian step off the pavement, the driver considers him as ‘fair game’, he takes aim and accelerates.” The pedestrian has to jump, leap, and run for dear life. In these lines, the author is trying to tell the reader how reckless the drivers are and how they chase people as hunters do while hunting an animal.

However, in the next line, he compliments the people for their sweet and sensible temperament. He tells the reader that the pedestrian does not resent being targeted by the driver. He says, “driver and pedestrian – hunter and prey smile amicably at each other, and they appear to be saying “I win today you will tomorrow”.

In the next paragraph, the author talks about the rivalry between two drivers. Though the war between two drivers appears to be murderous, yet they are good-tempered. He describes the style of their driving – they cut in, overtake on both sides, force you to brake violently and commit all the most heinous crimes on the road twenty times an hour”. Despite exhibiting such recklessness in their driving, they smile at you and do not show any anger, no hostility, and no mad hooting.

In the next paragraph, he recalls an incident he had probably witnessed in a place called Avenida Presidente Vargas. He says it is the worst place in Brazil known for its crowded and slow-moving’ traffic. His statement is paradoxical. He says, on the one hand, that driver’s drive recklessly; and here he calls the traffic ‘crawling traffic’. He says even the onlookers will be contemplating the truly fascinating problem “how can crawling traffic proceed at such terrifying speed”. One can imagine the number of vehicles moving at such terrific speed and probably it is the number of vehicles moving at a time together which makes the reader call it ‘crawling traffic’. He comments about the helplessness of the pedestrian who wishes to cross the road waiting for hours on end.

Then, he concludes narrating a jovial anecdote. He tells the reader that he might witness a situation in which a man standing beside you on your side of the road, suddenly discovers a friend of his on the other side and starts waving to him. He asks him, “How on earth did you ever get there?” The other fellow yells back, “How? I was born on this side”. The author narrates this anecdote probably to convince the reader how difficult it is to cross a busy road in Avenida Vargas.

Japan and Brazil through a Traveller’s Eye Summary in Kannada

2nd PUC English Textbook Answers Springs Chapter 11 Japan and Brazil through a Traveller’s Eye image - 2

Glossary:

Mania: obsession, a craze
Condescend: stoop, humiliate
Genetic: hereditary, inherited
Subtly: delicate, cunning, elusive
Tokaido, Osaka, and Tokyo: places in Japan
Serenity: peacefulness, calmness
Heinous: hateful, wicked
Copacabana, Avenida, Presidente Vargas: places in Brazil

The main aim is to share the knowledge and help the students of 2nd PUC to secure the best score in their final exams. Use the concepts of Karnataka 2nd PUC English Answers Chapter 11 Japan and Brazil through a Traveller’s Eye in Real time to enhance your skills. If you have any doubts you can post your comments in the comment section, We will clarify your doubts as soon as possible without any delay.

2nd PUC English Model Question Papers with Answers 2019-20 Karnataka

October 28, 2023

Expert Teachers at KSEEBSolutions.com has created New Syllabus Karnataka 2nd PUC English Model Question Papers with Answers 2019-20 Pdf Free Download of 2nd PUC English Previous Year Board Model Question Papers with Answers are part of 2nd PUC Model Question Papers with Answers. Here We have given the Department of Pre University Education (PUE) Karnataka State Board Syllabus Second Year Model Question Papers for 2nd PUC English Model Question Papers with Answers 2019-2020 Pdf. Students can also read 2nd PUC English Question Bank with Answers hope will definitely help for your board exams.

Karnataka 2nd PUC English Model Question Papers with Answers 2019-2020

Karnataka 2nd PUC English Blue Print of Model Question Paper

2nd PUC English Blue Print of Model Question Paper 1

2nd PUC English Blue Print of Model Question Paper 2

2nd PUC English Blue Print of Model Question Paper 3

We hope the given New Syllabus Karnataka 2nd PUC Class 12 English Model Question Papers with Answers 2019-20 Pdf Free Download of 2nd PUC English Previous Year Board Model Question Papers with Answers will help you. If you have any queries regarding Karnataka State Board Syllabus Second PUC Class 12 Model Question Papers for 2nd PUC English Model Question Papers with Answers 2019-2020 Pdf, drop a comment below and we will get back to you at the earliest.

2nd PUC English Textbook Answers Springs Chapter 6 When You Are Old

October 28, 2023

Students who are in search of When You Are Old Questions and Answers Pdf, Notes, Summary. First check in which chapter you are lagging and then Download Karnataka Board 2nd PUC English Textbook Answers Chapter Wise. Students can build self confidence by solving the Answers with the help of Karnataka State Board 2nd PUC. English is the scoring subject if you improve your grammar skills. Because most of the students will lose marks by writing grammar mistakes. So, we suggest you to Download Karnataka State Board 2nd PUC English Answers according to the chapters.

Karnataka 2nd PUC English Textbook Answers Springs Chapter 6 When You Are Old

Check out the topics covered in 2nd PUC before you start your preparation. Improve your grammar skills with the help of Karnataka 2nd PUC English pdf links. The Answers for Karnataka 2nd PUC English Textbook Springs Chapter 6 When You Are Old are prepared by the English experts. So, if you follow Karnataka 2nd PUC English Textbook Answers you can cover all the topics in 2nd PUC Chapter 6 When You Are Old. This helps to improve your communication skills.

When You Are Old Questions and Answers, Notes, Summary

When You are Old Comprehension I

When You Are Old Notes KSEEB Solution Question 1.
The speaker is addressing
a. a young woman he has loved
b. an old woman that he has met now
c. the woman that he has admired in his life.
Answer:
(a) a young woman he has loved.

When You Are Old Questions And Answers KSEEB Solution Question 2.
Inline two, the word ‘book’ refers to
a. memories
b. book of poems
c. an album
d. diary.
Answer:
(a) memories.

When You Are Old Poem Questions And Answers KSEEB Solution Question 3.
The words ‘glad grace’ suggest
a. her physical beauty
b. her inner beauty
c. her goodness.
Answer:
(a) her physical beauty.

When You Are Old Summary 2nd Puc KSEEB Solution Question 4.
‘Pilgrim soul’ means the soul
a. which is immortal
b. that has gone on a pilgrimage
c. which is questing for true love.
Answer:
(c) which is questing for true love.

When You Are Old Summary KSEEB Solution Question 5.
What does the phrase ‘your changing face’ suggest?
Answer:
It suggests that her youth and beauty will fade away. When she grows old her face will get shrunk and will look different.

When You Are Old Class 8 Question Answer KSEEB Solution Question 6.
‘Love fled’ connotes
a. the death of the man who loved her
b. the fleeing of her lover to the mountains
c. the loss endured by her.
Answer:
(b) the fleeing of her lover to the mountains.

When You Are Old Comprehension II

When You Are Old 2nd Puc Notes KSEEB Solution Question 1.
How is the ‘one-man’ different from the many others who loved the lady?
OR
How does the speaker distinguish/contrast his love from/with that of the others?
Answer:
The narrator/speaker asks his lady love to presume that she has grown old and grey and is sitting by the fire nodding. Then he asks her to read from her book of memories and reminisce her past when she was in her prime youth. While she is thus engaged in recalling her past, the poet reminds her that though she undoubtedly had a great many suitors who admired her beauty and elegance and professed ‘love’ which may be true or false, he alone loved her unconditionally.

He says that he loved her inner beauty and even the fading away of her youth and beauty. The phrase ‘how many’ in the first line (second stanza) stands in contrast to ‘But one man’, in the third line (second stanza). While many suitors loved her beauty and elegance, he alone loved her pilgrim soul as well as the sorrows of her changing face.

Question 2.
How does the poem bring out the transient nature of beauty as against the permanence of love?
Answer:
Yes. The poem, while making an attempt to persuade the young lady not to ignore him or his love, also incidentally highlights the transient nature of beauty as against the permanence of love. In fact, the speaker’s argument is that, whereas all her suitors are merely attracted by her youthful beauty and elegance, he is attracted by her pilgrim soul. Furthermore, he claims that the love exhibited by her many suitors may not last long and might change as she grows old.

On the contrary, his love will remain constant and unconditional. He would love her ‘pilgrim soul’ as well as the changes in her face which appear as one grows old. Thus, the poet, using the phrase ‘your moments of glad grace’ in the first line of the second stanza in contrast with the phrase ‘sorrows of your changing face’ in the fourth line suggests that ‘beauty’ is transient in nature whereas ‘love’ is permanent.

When You Are Old Comprehension III

Question 1.
Comment on the usage of the time frame by the poet in ‘When You Are Old’.
OR
‘The speaker addresses a young lady in her old age’. Comment on the time sequence used by the poet.
OR
The speaker in ‘When You Are Old’ is not addressing an old lady. Explain.
Answer:
‘When You Are Old’ is a short love poem in which the poet uses a time frame in which the speaker addresses his lady love in the present, takes her to an unreal condition in the future, and asks her to recall her past memories. He does so, in order to persuade her or warn her not to ignore him and make a wrong decision.

The poet’s point of view is the most compelling point of the poem. The narrator is calling upon a woman that is not yet through with youth to, once past her prime, recall the days he was in her life and very much in love with her. Obviously, he wants her to remember him for his unique and unconditional love for her, and how she is choosing to ignore it in the present. By writing this poem in this fashion, the woman, when the poet hopes that she grows old, will remember the days when she was young with happiness but will grow regretful that she did not take advantage of his love.

Alternatively, the woman, in the present will see what an opportunity she is missing by ignoring his love for her and leaving him to fade into the past. The speaker fears that his lady love will not act upon his love for her and that she will only remember him in the book of memories. He hopes that if, once old, she puts down the book of memories, she will grow chilly and sorrowful that she did not foresee how steadfast his love was but how foolish she was for taking no notice of it. He is already fearful that she will grow old without him, and this can be seen as he requests that she remember him a ‘little sadly’ and as a missed chance to have a happy future.

It is the time frame that the poet has used in this poem that facilitates the poet to write this sad and reminiscent poem which is not designed primarily to make an old woman regretful, but to keep a young woman from ignoring the narrator and making the wrong decision.

Question 2.
‘When You Are Old’ is a poem of contrasts. What purpose do they serve?
Answer:
‘When You Are Old’ was written to show the true and unforgettable love from the writer. The theme is a painful one of unrequited love, which the poet manipulates in an interesting manner. Instead of focusing upon the present or the past, Yeats looks to the future, a future in which the two people in the poem are destined to be forever apart. The poet visualizes an unreal condition that the woman he loved became old and felt regret for refusing his true love.

To depict such a theme, the poet deploys elegant and quiet words and builds pictures of contrasts:
In the first stanza, there is a contrast between her elegant youth and her depressing old age. In the second stanza, there is a contrast between her many suitors who professed superficial love and the speaker or the lover who promises true love for her. In the third stanza, there is a contrast between the fleeting or transient love represented by her many suitors and the personified love of the poet.

There is a contrast between ‘the sorrows of your changing face’ in the second stanza and ‘murmur a little sadly’ of the third stanza. ‘The sorrows’ indicate passion or strong emotion is seen in young people and ‘little sadly’ reflects the listlessness of old people. There is a contrast between the ‘beauty and elegance’ sans her soul sought after by the suitors and the ‘pilgrim soul’ in the same lady cherished by the speaker. The many suitors who wanted to court her were attracted by her superficial beauty whereas this lover/speaker was attracted by her pilgrim soul, which symbolizes her inner self. These contrasts serve to build a strong argument to persuade the young lady not to ignore him now and regret later.

When You Are Old Additional Questions and Answers

I. Answer the following questions in a word, a phrase, or a sentence each:

Question 1.
To whom is the poem ‘When You Are Old’ addressed?
Answer:
To a young lady with whom the speaker is deeply in love.

Question 2.
What does the speaker want his beloved to do sitting by the fire?
OR
What does the speaker want his beloved to do when she is old?
Answer:
The speaker wants his beloved to sit by the fire and reminisce her memories of the past

Question 3.
Where, according to the speaker, had love hidden his face?
Answer:
According to the speaker, ‘love’ had hidden his face amid a crowd of stars.

Question 4.
Who, according to the speaker, will be‘nodding by the fire’?
Answer:
The speaker depicts his lady love as an old woman sitting beside the fire, nodding her head.

Question 5.
What does the speaker suggest his beloved to dream of?
Answer:
When she is old and grey, the speaker wants his beloved to dream of the soft look her eyes once had and the deep shadows they now have.

Question 6.
What does ‘the sorrows of your changing face’ refer to?
Answer:
The sorrows of your changing face’ refer to the changes seen in her face as she grows older. Her face will have shrunk and wrinkles will have appeared on her forehead and face depicting the difficulties and sorrows faced by her over the years.

Question 7.
According to the speaker, in what way is his love for the lady different from that of others?
Answer:
While many suitors loved her beauty and elegance, he alone loved her pilgrim soul as well as the sorrows of her changing face.

Question 8.
Mention any one aspect that the speaker loved in his beloved.
Answer:
The speaker loved her ‘inner beauty’ and her ‘pilgrim soul’.

Question 9.
According to the speaker, what will the woman regret in her old age?
Answer:
The woman will regret that she had rejected the speaker’s, true love.

Question 10.
What is meant by’pilgrim soul’?
Answer:
The phrase ‘pilgrim soul’ means the soul which is in quest of ‘true love’.

Question 11.
What look did the woman’s eyes have once in ’When You Are Old’?
Answer:
Soft look.

Question 12.
The speaker in ‘When You Are Old’ addresses
(a) a young lady
(b) an old lady
(c) a little girl.
Answer:
(a) a young lady.

Question 13.
When, according to the speaker in ‘When You Are Old’, will the lady be ‘grey and full of sleep’?
Answer:
According to the speaker in ‘When You are Old’, the lady will be grey and full of sleep when she sits nodding by the fire.

Question 14.
According to the speaker in ‘When You Are Old’, what did many admire the lady for?
Answer:
According to the speaker in ‘When You are Old’, many suitors were mesmerized by her beauty and elegance.

Question 15.
In the poem “When You Are Old’ many loved the lady’s
(a) huge wealth
(b) physical beauty
(c) pilgrim soul.
Answer:
(b) physical beauty.

Question 16.
Who, according to the speaker in ‘When You Are Old’, loved the beloved’s pilgrim soul?
Answer:
According to the speaker in ‘When You are Old’, it was he (the speaker) who loved her pilgrim soul.

Question 17.
Whose pilgrim soul did the speaker in ‘When You Are Old’ love?
Answer:
The speaker in ‘When You are Old’, loved the pilgrim soul of a young lady whom he is addressing in the poem and whose beauty and elegance mesmerized many suitors.

Question 18.
What did the speaker in “When You Are Old’ love besides the pilgrim soul?
Answer:
The speaker in ‘When You are Old’, loved the pilgrim soul, as well as the changes that appeared in her face as she grew older.

Question 19.
Who, according to the speaker in ‘When You Are Old’, fled and hid his face?
Answer:
According to the speaker in ‘When You are Old’, ‘Love’ (personified ‘love’] fled and hid his face.

Question 20.
Where, according to the speaker in ‘When You Are Old’, did love pace upon?
Answer:
According to the speaker in ‘When You are Old’, ‘Love’ paced upon the mountains for a while and then disappeared.

Question 21.
What, according to the speaker in ‘When You Are Old’, did love hide amid a crowd of stars?
Answer:
According to the speaker in ‘When You are Old’, ‘Love’ (personified love) hid his face amid a crowd of stars.

Question 22.
The speaker loved the _____ of his beloved’s changing face in ‘When You Are Old’.
(a) grace
(b) sorrows
(c) soft look.
Answer:
(b) sorrows.

Question 23.
In ‘When You Are Old’, the speaker suggests to his beloved to slowly read his book when she is
(a) glad
(b) old
(c) proud.
Answer:
(b) old.

II. Answer the following questions in a paragraph of 80-100 words each:

Question 1.
Why does the poet ask his beloved to reflect upon the bygone days and the present moment?
OR
What does the speaker in When You Are Old’suggest to his beloved to reflect upon?
Answer:
The poet asks his beloved to reflect upon the bygone days and the present moment because he seems to be apprehensive that she will continue to ignore him, her beauty will vanish soon and she will be alone in her old age. That is why he is asking her to presume that she has grown old and grey and try to foresee her predicament in the future. He does so because he intends to persuade her to pay attention to his ‘value’ as a lover before it is too late.

Question 2.
Examine the theme of opposing stability of true love and the fickleness of false love in the light of the poem.
OR
How does the speaker express his longing for his beloved?
OR
Whose love in ‘When You Are Old’ is true and intense? Explain.
OR
How does the speaker in ‘When You Are Old’ bring out his love for his beloved against the changing circumstances and ravages of time?
Answer:
‘When You Are Old’ presents the moral dilemma faced by a sincere lover. The speaker/narrator is the sincere lover and his lady love is the one who is going to make a decision. The lady has attracted many suitors including the speaker. The speaker believes that the other suitors only love her physical charms, and not all of them are sincere in their love towards her. He means to say that they are fickle-minded and once she loses her charms they will desert her. On the other hand, he believes that he loves her truly and according to him true love is the love of the inner self and has a spiritual aspect in it. But, the lady has not responded to his love.

He feels frustrated and as a last attempt, he tries to tell her the reality. He wants her to realize that physical beauty is transient and love of the fickle-minded will also be transient. He wants to assure her that only his love will be stable and if she ignores him and makes a wrong decision she will regret it later. Thus the speaker tries to persuade his lover to make the right decision and receive his love, which is true and intense.

Question 3.
How does the poet express his feelings for his love in the poem?
OR
How is love that is not reciprocated by the speaker’s beloved expressed in the poem?
Answer:
The poet asks his love, who is still young, to imagine a time when she is past her prime youth. She would then be an old woman with grey hair and sleepy eyes. When she is in such a state, he wants her to read a book of memories from her youth. As the woman sits beside the fire, nodding her head and leaves through her memories, she would recollect the ‘soft looks’ she once had and the sorrows she had suffered until then. When she recalls her faded beauty she would also recall how she was admired by many suitors who were infatuated with her physical charms. At the same time, she would also recall how there was one man who loved her unique soul which was in search of true love. She would also realize that her true love has lingered on for a while, disappeared from the earth and hid amidst a crowd of stars in heaven.

Question 4.
The poem ’When You are Old’highlights the feelings of a true lover. Explain.
OR
How is the speaker’s passionate love for his beloved brought out in the poem?
Answer:
‘When You are Old’ highlights the writer’s true and unforgettable love for a lady. It presents the concern of sincere love for the future predicament of his lady love. Instead of focusing upon the present or the past, the poet looks to the future, a future in which the two people in the poem are destined to be forever apart. The poet imagines that the woman he loved has become old and regrets her refusing his true love.

The poem begins with the presumption that an old and grey lady is sitting beside the fire nodding her head. When she recalls her memories, she remembers the soft look that her eyes had once, and the number of suitors who tried to court her, being charmed by her elegance and beauty. While admitting that many suitors were attracted by her youthful beauty, the speaker tries to tell her that he was the only lover who loved the pilgrim soul in her.

He wants her to know that unlike others he was attracted by the beauty of her inner self and his love would remain constant even in her old age. He assures her that he loves even the sorrows of her changing face. He wants her to understand that over a period of time her beauty will have faded away and she will have grown old, with her face having shrunk and her skin has been wrinkled, indicating that she has passed through many difficulties and sorrows.

The speaker concludes visualizing that she is now bending down beside the dying fire, and she tells herself in a whisper, in a regretful tone that her true love has fled and is hiding his face amidst a crowd of stars. Thus, the whole poem is the delineation of the intense feelings of a true lover.

III. Answer the following questions in about 200 words each:

Question 1.
The poem ‘When You Are Old’ conveys the message that true love is indestructible and constant. Elucidate.
OR
The poem ‘When You Are Old’ brings out the feelings of eternal love. Explain.
OR
‘True love is related to the soul but not to the physical beauty’. How is this idea brought out in the poem ‘When You Are Old’?
OR
Whose love in ‘When You Are Old’ is immortal? How does the poem present this?
Answer:
‘When You Are Old’ depicts the frustration and anxiety of a sincere lover. At the same time, it also presents the concern of sincere love for the future predicament of his lady love.

The lover’s frustration and anxiety for her future find expression only because his love is genuine and constant. Though she has not responded to his ‘love’, the lover does not want to give up his attempts to persuade her. He tries to place before her a realistic, projected picture of her future only because he knows that she will be old, infirm, and companionless as years roll by. Furthermore, the poet seems to understand that she is not a flirt and a worldly lady who loves to indulge in the pleasures of life but one who has also been looking for someone who really loves her inner self more than her physical charms. Only someone who has a similar state of mind, and beliefs, and who is also yearning for a spiritual union with his lady love alone can write so.

From this, one can infer that true love is indestructible and constant. Had the speaker been interested in her physical charms only he would not have bothered to foresee his own future as well as the predicament of his lady love some twenty years hence. Thus it can be argued that the poem ‘When You Are Old’ conveys the message that true love is indestructible and constant and is not influenced by the vicissitudes of fortune.

Question 2.
“But one man loved the pilgrim soul in you”. How does the speaker justify this?
OR
How does the poem distinguish the speaker’s love from that of others?
Answer:
‘When You are Old’ is a love poem in which the poet addresses his lady love in the present, takes her to an unreal condition in the future, and asks her to recall her past memories. He does so, in order to persuade her or warn her not to ignore him and make a wrong decision. This timeframe is the most compelling point of the poem because he wants her to remember him for his unique and unconditional love for her.

The poet compares himself as a suitor with other suitors. He says, ‘but one man loved the pilgrim soul in you’. He tries to distinguish himself from all the other suitors who tried to woo her. He argues that all the other suitors were only attracted by her physical and external beauty and naturally they would be put off by her looks of old age and might stop showing any interest in her. On the other hand, unlike other suitors, he loved her not for her physical beauty but for her ‘pilgrim soul’.

The word ‘pilgrim soul’ has a reference to the biblical belief that every soul is a pilgrim on the way to salvation and redemption. The speaker, by referring to this aspect of the beloved rather than to her beauty and fame, seeks oneness with the inner spiritual self and not the external self.

Question 3.
‘When You Are Old’ makes the ‘beloved’ look back on her youth. Discuss.
Answer:
‘When You Are Old’ is a short love poem in which the poet uses a time frame in which the speaker addresses his lady love in the present, takes her to an unreal condition in the future, and asks her to recall her past memories. He does so in order to persuade her or warn her not to ignore him and make a wrong decision.

The poet’s point of view is the most compelling point of the poem. The narrator is calling upon a woman who is not yet through with youth to, once past her prime, recall the days he was in her life and very much in love with her. Obviously, he wants her to remember him for his unique and unconditional love for her, and how she is choosing to ignore it in the present. By writing this poem in this fashion, the woman, when the poet hopes that she grows old, will remember the days when she was young with happiness but will grow regretful that she did not take advantage of his love.

When You Are Old by W.B. Yeats About the Poet:

William Butler Yeats (1865 – 1939) is an Irish poet and one of the foremost figures of 20th-century literature. His early poetry is part of the Celtic twilight or the Irish Literary Revival and it uses the history, myths, and heroic figures of Ireland. Yeats wrote plays, was one of the founders of the Abbey Theatre in Dublin, and was witness to the revolutionary politics of Ireland. He was awarded the Nobel Prize for literature in 1923. Later, under the influence of Ezra Pound, he began to write modernist poetry. He is one of the great love poets of the world.

In this love poem, the speaker (who is the lover) imagines his beloved in the future when she is old and reading the book of poems he is now writing. Reading the book she will remember the past, her youthful beauty, and the many who admired her. While the others loved her physical beauty and grace, only he loved her soul and her soul’s search for something meaningful. However, she will also feel sad that that love also vanished.
Note how love is personified in the last lines.

Background:

Most critics opine that this poem is a real description of the poet’s love. In 1889 Yeats met his great love Maud Gonne, an actress, and Irish revolutionary. However, she married Major John Macbride in 1903, and this episode inspired Yeats to write down this heart-stirring poem. Many critics believe that the poem presents the resentment he feels towards her for not reciprocating his love. The ambiguity of the last stanza of the poem illustrates that the poem is not only about the regret the woman feels when she becomes aware of what she has lost, it also strengthens the argument that this work is actually about the resentment he wants the woman to perceive.

When You Are Old Summary in English

‘When You Are Old’ was written to express the writer’s true and unforgettable love. The theme is a painful one of unrequited love, which the poet manipulates in an interesting manner. Instead of focusing upon the present or the past, the poet looks to the future, a future in which the two people in the poem are destined to be forever apart. The poet imagines an unreal condition that the woman he loved became old and felt regret for refusing his true love.

That is why the poem begins with the presumption that an old and grey lady was sitting beside the fire nodding her head. She is imagined to be reminiscing her memories when she recalls the soft look that her eyes had once, and how many suitors tried to court her, being charmed by her elegance and beauty.

2nd PUC English Textbook Answers Springs Chapter 6 When You Are Old image - 1
While admitting that many suitors were attracted by her youthful beauty, the speaker tries to tell her that he was the only lover who loved the pilgrim soul in her. He wants her to know that he was attracted by the beauty of her inner self and his love would remain constant even after she grows old. He assures her that he loves even the sorrows of her changing face. He means to say that over a period of time her beauty will have faded away and she will have grown old with her face having shrunk and skin having been wrinkled indicating that she has passed through many difficulties and sorrows.

He concludes visualizing that she is now bending down beside the dying fire, and tells herself in a whisper in a regretful tone that her true love has fled and is hiding his face amid a crowd of stars.

Analysis of the Poem:

‘When You Are Old’ is a short, exquisite, love lyric of twelve lines. It is a sad and introspective poem and is written in a melancholic tone.

The poem is in the form of a direct address by a lover to his lady love. In the poem, there are three stanzas of four lines each with a constant rhyme. The rhyme scheme hints that the speaker/ lover tries to tell her that his love will remain constant even when she grows old.

The most important aspect of this poem is the point of view taken by the narrator. The narrator is asking a woman, who is still young, to imagine a time when she is past her prime youth.

When you are old and gray and full of sleep,
And nodding by the fire, take down this book,
And slowly read, and dream of the soft look
Your eyes had once, and of their shadows deep;

The poet tries to put her mind in the future when she is an ‘old and gray’ woman, ‘full of sleep’, to ‘slowly read’ a book of memories from her youth. As the woman is ‘nodding by the fire’ she leafs through the book (her memories) and recollects her days of’soft looks’ and ‘sorrows’ as she changed.

How many loved your moments of glad grace,
And loved your beauty with love false or true,
But one man loved the pilgrim soul in you.
And loved the sorrows of your changing face;

She remembers her faded beauty that was admired by many but then recalls the only man, the narrator, who loved her for her unique soul. He loved her even as she grew less beautiful and as her personality changed in the fullness of time. The alliteration ‘glad grace’ expresses that when she is young, beautiful and in her best moments of life many will be interested in her, but their love for her will be just false or superficial love. However, the narrator (speaker) will love her anyway no matter what happens to her beauty.

The line “and loved the sorrows of your changing face” suggests that when she gets old her face gets shrunk. So her face looks different but he will just love her with the same love he always had. There is also a contrast between ‘glad grace’ and ‘sorrows of your changing face’, which suggests that while the others love her in her happy times, he will love her every time, including the worst ones.

The phrase ‘pilgrim soul’ in the line, “but one man loved the pilgrim soul in you,” refers to the long walk that her soul has had, searching for real happiness, but really being alone. So, many lovers can love her for how she looks but only he can love her for who she really is. ‘Pilgrim Soul’ has reference to the Biblical belief that every soul is a pilgrim, on the way to salvation and redemption. The speaker, by referring to this aspect of the beloved rather than to her beauty and fame, evokes oneness with the inner and not the external self.

And bending down beside the glowing bars,
Murmur, a little sadly, how Love fled
And paced upon the mountains overhead
And hid his face amid a crowd of stars.

In this stanza, the speaker exhorts the loved one to remember him in later years as she sits beside the fire and bends over the embers of the fire. The onomatopoeic ‘murmur’ suggests a whisper that shows that she has no passion or zest left. This adds to the imagery of age and weariness. ‘A little sadly’ suggests that in later years, as she remembers the speaker, she should feel regretful. The poet uses the word ‘love’ in all the lines in the second stanza and in the third stanza, second line, he capitalizes the word ‘Love’, giving it much intensity. He personifies ‘Love’ in the second line. In the lines,

And paced upon the mountains overhead
And hid his face amid a crowd of stars

‘pacing’ suggests that she was given a chance as ‘Love’ waited for her; it also suggests the gradual diminishing of the love which may then loiter over mountains for a while and then disappear. Being on the ‘mountains overhead’ suggests that ‘Love’ waited on a higher plain than that which she inhabited. Capitalized ‘L’ for love suggests that it is not just a person that she has lost but the ultimate, true, and everlasting possibility of love. The phrase ‘how love fled’ refers to the possibility that the speaker’s love would just fly far away because she is not receptive to his love.

When You Are Old Summary in Kannada

2nd PUC English Textbook Answers Springs Chapter 6 When You Are Old image - 2

Glossary:

The pilgrim soul: questing soul
The glowing bars: the bars of the fire place full of glowing coal (‘Bars’ refers to the bars of the iron grate in the fire-place)
Nod: move one’s head up and down repeatedly
Murmur: a softly spoken or almost inaudible utterance
full of sleep: drowsy due to old age
moments of glad grace: the days of youth when she was graceful
nodding: feeling sleepy; a sign of sleepiness
pilgrim soul: a Biblical belief that every soul is a pilgrim, on the way to salvation and redemption.

The main aim is to share the knowledge and help the students of 2nd PUC to secure the best score in their final exams. Use the concepts of Karnataka 2nd PUC English Answers Chapter 6 When You Are Old in Real time to enhance your skills. If you have any doubts you can post your comments in the comment section, We will clarify your doubts as soon as possible without any delay.

2nd PUC English Textbook Answers Springs Chapter 12 The Voter

October 28, 2023

Students who are in search of The Voter Questions and Answers Pdf, Notes, Summary. First check in which chapter you are lagging and then Download Karnataka Board 2nd PUC English Textbook Answers Chapter Wise. Students can build self confidence by solving the Answers with the help of Karnataka State Board 2nd PUC. English is the scoring subject if you improve your grammar skills. Because most of the students will lose marks by writing grammar mistakes. So, we suggest you to Download Karnataka State Board 2nd PUC English Answers according to the chapters.

Karnataka 2nd PUC English Textbook Answers Springs Chapter 12 The Voter

Check out the topics covered in 2nd PUC before you start your preparation. Improve your grammar skills with the help of Karnataka 2nd PUC English pdf links. The Answers for Karnataka 2nd PUC English Textbook Springs Chapter 12 The Voter are prepared by the English experts. So, if you follow Karnataka 2nd PUC English Textbook Answers you can cover all the topics in 2nd PUC Chapter 12 The Voter. This helps to improve your communication skills.

The Voter Questions and Answers, Notes, Summary

The Voter Comprehension I

The Voter Questions And Answers 2nd PUC KSEEB Solution Question 1.
The roof was a popular young man because of he-
a. had not abandoned his village.2nd PUC English Textbook Answers Springs Chapter 12 The Voter
b. wanted to guide his people.
c. was forced to return to his village.
Answer:
(a) had not abandoned his village.

The Voter Summary 2nd Puc KSEEB Solution Question 2.
Why was Marcus considered rich and powerful?
Answer:
Marcus had two long cars and had just built himself the biggest house anyone had seen in these parts. He had entertained his people slaughtering five bulls and countless goats to feed the people. Though the village had no electricity, he had installed a private plant to supply electricity to his new home. Secondly, his house was opened by the Archbishop. Thirdly, he had been made a minister after he won the election. That is why he was considered rich and powerful.

The Voter Notes 2nd PUC KSEEB Solution Question 3.
Marcus Ibe had earlier been
a. doctor
b. schoolteacher
c. politician.
Answer:
(b) school teacher.

The Voter 2nd Puc Notes KSEEB Solution Question 4.
The fact that Marcus Ibe left the good things of the capital and returned to his village whenever he could, shows
a. his devotion to his people and love for the place
b. he enjoyed all the comforts of the city in his village.
c. he wanted to improve the amenities in his village.
Answer:
(b) he enjoyed all the comforts of the city in his village.

2nd Puc English Lesson The Voter Summary In English KSEEB Solution Question 5.
After the feasting, the villagers
a. praised Marcus’s faithfulness and generosity.
b. intended to demand more for their votes
c. realized Marcus’s wealth.
Answer:
(c) realized Marcus’s wealth.

Voter Notes 2nd PUC KSEEB Solution Question 6.
The ‘whispering campaign’ is
a. secret campaigning at night
b. bargaining for votes.
c. clandestine distribution of money.
Answer:
(c) clandestine distribution of money.

The Voter By Chinua Achebe Questions And Answers 2nd PUC KSEEB Solution Question 7.
The village eider Ezenwa tilted the lamp a little because
a. he could not see properly.
b. the place was too dark.
c. he wanted to confirm the amount paid to each.
Answer:
(c) he wanted to confirm the amount paid to each.

Question 8.
‘Firewood’ refers to
a. Roof taking advantage of the situation.
b. the advantages of being a voter.
c. the benefits the elders received.
Answer:
(c) the benefits the elders received.

Question 9.
Roof and the leader of the POP campaign team were
a. friends.
b. strangers.
c. acquaintances.
Answer:
(a, c) friends/acquaintances.

Question 10.
The roof was mesmerized by
a. the red notes on the floor.
b. the picture of the cocoa farmer.
c. the POP campaign leader.
Answer:
(a) the red notes on the floor.

Question 11.
Roof’s act of inserting the torn ballot papers in two boxes signifies
a. keeping his promise.
b. appeasing Iyi
c. absolving himself of his guilt
Answer:
(c) absolving himself of his guilt.

The Voter Comprehension II

Question 1.
Trace the change in the attitude of the villagers before the second election. Give reasons.
OR
Why did the people decide not to cast their vote for free in the forthcoming election?
OR
Explain the change that had come into the thinking of Umuofia in ‘The Voter’.
OR
Trace the reasons behind the ‘radical change’ that had come into the thinking ofUmuofia in ‘The Voter’.
Answer:
In this short story, Chinua Achebe makes an attempt to present before the reader how ‘elections’ lose their sanctity and are misused by greedy politicians for self-aggrandizement instead of bringing about improvement in the life of the people.

The people of Umuofia vote en masse in favor of the People’s Alliance Party and elect Marcus Ibe, a local teacher, as their leader. Once getting elected, people see a great many changes in Marcus’ life. Their elected representative becomes wealthy, is awarded chieftaincy titles and doctorate degrees, besides many other honours. He also builds a huge mansion in his native village and names it ‘Umuofia Mansions’. He spends most of his time in the capital and comes back to stay in his village mansion sometimes.

The villagers do not have running water and electricity, but he gets a private plant installed in his village to supply electricity to his new house. To top it all, his new house is opened by the Archbishop. On the day the new house is opened, he hosts a grand dinner to all the people of his village, slaughtering five bulls and countless goats. The common people realize that winning an election can change an ordinary mission school teacher into a wealthy and powerful man.

They also know that it is their ballot which has given him all those benefits. They recall that they had given their votes free of charge five years ago. They realize that they had underrated the power of the ballot paper and should not do so again. That is why, in the second election, they demand money for their votes.

Question 2.
What was the justification for the formation of the POP?
OR
What reasons are given for the formation of the POP?
Answer:
POP stands for Progressive Organisation Party. This party is formed by the tribes down the coast to save themselves from ‘totally political, cultural, social and religious annihilation’. The POP was a complete non-entity in the first election. Once the organizers come to know that there is no opposition party, some rich members of the tribes down the coast, form this party. They want the people to know that they will be paid pounds and not shillings if they listen to them and vote for them. In the story, we do not get any hint about the objectives of the PAP, but the organizers of the POP claim that they want to save the people from political, religious, cultural annihilation, though they appear to be no better.

From the situation described in the story, we can infer that the writer intends to tell the reader that no political party is seriously interested in improving the welfare of the people. They seem to know for sure that by winning an election, they can take the government in their hands and become rich. They seem to be unaware of their duties and responsibilities.

Question 3.
The roof is an intelligent manipulator. Justify with reference to the story,
OR
What is the role played by Roof in ‘The Voter’?
Answer:
Roof Okeke is an energetic young man and has come back to his own village Umuofia, after working as a bicycle repairer’s apprentice for two years in Port Harcourt. It is true that he would have enjoyed a rich life if he had stayed on in his job. But he comes back to the village and tells the people that he wants to guide them in difficult times. Later he becomes an ’election campaign manager’ for Marcus Ibe and over a period of five years becomes an expert in election campaigning at all levels.

In the story, we get to know more about Roof when he is on the job, engaged in one of his whispering campaigns. He tells the elders that Marcus Ibe, being a ‘son’ from their village, has been made a minister and it should be considered a great honour. Then he tries to use his rationalistic thinking with the people. He asks them, “Do you ever stop to ask yourselves why we should be singled out for this honour?” He himself answers his question. He tells them that they are favoured by the PAP leaders. Here we see Roof as a manipulator at work. He knows for sure that Marcus was perhaps the only man with some education.

Secondly, there was no opposition party. Basically, the village folk were innocent and naive and hardly realized the meaning of an election and the value of their mandate. Roof exploits this situation to his advantage.

Moreover, when he comes to learn that people had understood the benefits of entering politics and winning an election as seen in the case of Marcus Ibe and are now planning not to give their vote free of charge, he immediately informs Marcus and keeps him prepared to pay some money to the people in exchange for their votes.

Finally, we get a clear picture of Roof as a manipulator when he accepts five pounds from the election manager of POR He makes sure that they will not disclose the news of his accepting the money. He also tries to keep his conscience clear by telling them that he works for Marcus; while putting his ballot paper into the box, he cleverly manages to cast his vote for both Marcus and Maduka, without attracting the wrath of ‘iyi’.

The Voter Comprehension III

Question 1.
The POP campaign leader’s meeting with Roof shows the misuse of transparency in a democratic setup. Discuss.
Answer:
In this story, Achebe satirizes ‘politicians’ and makes an attempt to show how even in a democratic setup election can become farcical.

Unlike in dictatorship or monarchy, in a democratic setup, people have the freedom to elect a ‘person’ to work as their representative in the government and work for their upliftment. One of the hallmarks of democracy is its insistence on transparency. Every person is subject to scrutiny by the public in the election process. A person is free to vote for a person of his choice from among the contestants. ‘Transparency’ in this refers to Roof’s informing the POP campaign leader that he is working for Marcus.

Secondly, whether Roof informs him or not, it is well known to everyone that Roof is Marcus’ election campaign manager. The candidates are permitted by law to make known to the people all such appointments and maintain transparency in their dealings.

However, no candidate can strike a deal with the people and buy their votes. But this is done clandestinely. The POP campaign leader knows that Roof is working for Marcus. Therefore, they want to buy Roof’s vote first so that they will let him know that the POP leaders will pay in pounds and not in shillings. This is the message they want to put across to the people through Roof. Probably, next time, Roof who has made a name as an efficient election campaign manager for Marcus, will be bold enough to demand ‘pounds’ instead of shillings from Marcus and if he refuses to pay in pounds, he might go and work for the POP leader. That way he will be able to motivate his people to vote for POP instead of the circus. Thus, the POP campaign managers’ meeting with Roof is daring misuse of transparency in a democratic setup.

Question 2.
To every human comes a time of reckoning. How does Roof’s dilemma on the day of the election reflect this?
OR
Why did Roof face a dilemma while he was inside the voting booth? How did he resolve it?
OR
“There comes a time in every man’s life to decide”. Explain with reference to ‘The Voter’.
Answer:
In ‘The Voter’, Roof is an energetic young man who has the reputation of sacrificing a bright future for the sake of the people of his village. The roof has worked in a city for two years and has experience in dealing with both literates and illiterates. Based on this knowledge of general human behaviour, Roof builds for himself a career as an election campaign manager for Marcus Ibe.

When the story opens, we learn that Marcus Ibe is Chief the Honourable Minister of Culture in the outgoing government and is seeking people’s mandate for a second term. We also learn that Marcus considers Roof a real expert in election campaigning at all levels. Roof knows how politicians make money. He also knows that the people of Umuofia have now decided to vote for Marcus for a price. Roof conveys this news to Marcus and prepares him beforehand.

Like an efficient manager, he tries to highlight the strong points of PAP and finally strike a bargain with the voters paying them four shillings per vote. Everything is now going according to his plan and is happy.

But, one evening, the leader of the POP campaign team comes to his house to meet him. He tempts Roof to vote for Maduka paying him five pounds. Roof’s greed tempts him to accept the offer. But, he also gets trapped. The leader asks him to swear on the ‘Iyi’, that he would vote for Maduka.

The roof cannot go back on his promise. So he swears to vote for Maduka. Finally, on the day of the voting, Marcus wants to make sure that every one of his people casts his vote without fail. Therefore, Roof being his election manager, Marcus sends him to the booth first to vote. Now, Roof is caught in a moral dilemma. All along he has worked for Marcus and his conscience does not permit him to cheat Marcus. Now, when Marcus asks him to cast his vote he is in two minds. He feels that he cannot betray Marcus. For a moment, his mind tells him that he should vote for Marcus and go back and return the money he had received from the POP election manager.

Secondly, he remembers that he had sworn on that ‘lyi’. In a few seconds, his mind hits on a new idea. He folds the ballot paper in the middle and tears it into two halves at the crease. He drops the first half into the box meant for Maduka and confirms his vote saying that he votes for Maduka. Since he has not taken an oath to vote for Marcus, there is no fear of ‘lyi’ in him but only his guilt. Since he has worked as his manager for money and rewards, he drops the other half into the box meant for Marcus. Thus, he ensures that he does not cheat either. By tearing the ballot paper and casting his vote for both of them he overcomes his moral dilemma.

Question 3.
What comment does the story offer on the electoral system? Is it relevant?
Answer:
In ‘The Voter’, Achebe tries to present a true picture of the drama that is witnessed during an election held in a small town in Nigeria. There are two main characters and two political parties in the story and the voters are the villagers of Umuofia.

The roof is an energetic young man, who is now working as the election campaign manager for Marcus Ibe, who was once a local mission school teacher and was on the verge of dismissal from service on the basis of a female teacher’s complaint.

Marcus Ibe joins the People’s Alliance Party, contests the election, and gets elected. He becomes a minister and his government completes a five-year term and he is now seeking re-election. During his term as a minister, he amasses a lot of wealth, comes to own two big cars, builds a huge mansion in his native village, and wins several chieftaincy titles and doctorate degrees.

But his people remain the same – poor, illiterate, ignorant, and naive. The situation in his village also does not show any improvement. The same old problems of lack of running water and electricity continue to plague the people and prevent them from improving economically and politically. When the story opens, we learn that Marcus Ibe is seeking a second term and everyone is sure that he is going to get a landslide victory because there is no opposition party worth taking into consideration.

But there is a change in the perception of the people. After feasting in Marcus’ new house and noticing his wealth and power, the poor people of Umuofia realize that ‘elections’ do have a meaning and their votes which they had underrated before, are of high value. Therefore, they demand some value for their votes and Roof manages to convince them that they should vote for Marcus again by giving four shillings each.

Some tribes down the coast had set up a new political party called POP with the objective of saving the people from ‘totally political, cultural, social and religious annihilation’. Their campaign leader comes to Roof’s house and offers to buy his vote for five pounds. Roof initially is enamoured of the five-pound notes and agrees to vote.

But the POP leader cleverly gets him to swear on the ‘iyi’ that he will vote for Maduka. Finally, on election day, his conscience pricks him to a little extent and Roof feels like voting loyally for Marcus and returning the money to the POP election manager. But the oath he has taken before ‘iyi’ does not allow him to do so and there is a moral dilemma. However, Roof cuts his ballot paper in two, drops one half into the box meant for Maduka and drops the other half into the box meant for Marcus, and saves his conscience and his life too. This is the story in a nutshell.

There are lessons to learn from this story. We must note that merely naming our government as ‘democracy’ and setting up all the things needed for holding elections do not guarantee that people will be able to exercise their true mandate and will elect competent members to the government which will strive for the upliftment of the people socially, educationally, economically and culturally. We cannot guarantee democratic governance by merely holding elections regularly. First, we must educate the people about their rights, duties, and responsibilities in any election.

Secondly, we must ensure that people have the right to recall their representatives if they do not strive to improve a lot of people. Thirdly, elected representatives must be forced to account for their earnings after joining the government. Finally, there should be an authority which should oversee that sanctity of election procedures is not tampered with.

Also, those who get elected are self-centered and exploit the people and the situation to their benefit. On the whole, one can argue that by merely holding elections regularly we cannot ensure that a democratic government will come into place.

Secondly, the electoral system that is now in place in the story is not foolproof. As regards the relevance of the story to our own situation, one can argue that it is highly relevant even today. Even after nearly seven decades of independence, we have not been able to ensure free and fair elections, the main reason being the lack of education and moral values. We are witness to every type of corrupt practice in elections.

Question 4.
‘Democracy is more than holding elections regularly.’ Do you think the story supports this statement?
Answer:
In ‘The Voter’, Achebe tries to present a true picture of the drama that is witnessed during an election held in a small town in Nigeria. There are two main characters and two political parties in the story and the voters are the villagers of Umuofia.
The roof is an energetic young man, who is now working as the election campaign manager for Marcus Ibe, who was once a local mission school teacher and was on the verge of dismissal from service on the basis of a female teacher’s complaint.

But his people remain the same – poor, illiterate, ignorant, and naive. The situation in his village also does not show any improvement. The same old problems of lack of running water and electricity continue t<3. plague the people and prevents them from improving economically and politically. When the story opens, we learn that Marcus Ibe is seeking a second term and everyone is sure that he is going to get a landslide victory because there is no opposition party worth taking into consideration.

Also, those who get elected are self-centred and exploit the people and the situation to their benefit. On the whole, one can argue that by merely holding elections regularly we cannot ensure that a democratic government will come into place. Secondly, the electoral system that is now in place in the story is not foolproof.

As regards the relevance of the story to our own situation, one can argue that it is highly relevant even today. Even after nearly seven decades of independence, we have not been able to ensure free and fair elections, the main reason being the lack of education and moral values. We are witness to every type of corrupt practice in elections.

The Voter Additional Questions and Answers

I. Answer the following questions in a word, a phrase, or a sentence each:

Question 1.
Where did Roof work as a bicycle repairer’s apprentice?
OR
What did Roof do in Port Harcourt?
Answer:
Roof worked as a bicycle repairer’s apprentice in Port Harcourt.

Question 2.
How long had Roof worked as a bicycle repairer’s apprentice?
Answer:
Roof worked as a bicycle repairer’s apprentice for two years.

Question 3.
The roof had given up being a bicycle repairer’s apprentice in order to
(a) contest elections
(b) guide his people
(c) campaign for Marcus.
Answer:
(b) and (c) guide his people/campaign for Marcus.

Question 4.
Who had formed the Progressive Organisation Party?
Answer:
The Progressive Organisation Party (POP) had been formed by the tribes down the coast.

Question 5.
To which village did Roof belong?
Answer:
To Umuofia.

Question 6.
Who was supposed to be very popular in his village?
Answer:
Roof.

Question 7.
According to the villagers, what was Roof’s motivation for leaving a promising career?
Answer:
The villagers believed that a strong desire to guide the people of Umuofia in difficult times was the motivation behind Roof’s leaving a promising career.

Question 8.
Which political party did the people of Umuofia support?
Answer:
People’s Alliance Party.

Question 9.
To which party did Marcus belong?
Answer:
To the People’s Alliance Party.

Question 10.
What was Marcus in the outgoing government?
Answer:
Minister of Culture.

Question 11.
Who did the Roof campaign for in the elections?
Answer:
For Marcus Ibe.

Question 12.
What was Marcus Ibe before he joined politics?
Answer:
A mission school teacher.

Question 13.
Why did Marcus Ibe face the threat of being dismissed from service as a school teacher?
Answer:
A female teacher had complained against Marcus Ibe. Hence, his dismissal was imminent.

Question 14.
What had Marcus named his new house?
Answer:
‘Umuofia Mansions’.

Question 15.
Who was the most trusted campaigner of Marcus?
Answer:
Roof.

Question 16.
How much salary had Marcus withdrawn in advance?
OR
How much had Marcus Ibe drawn in advance for the election?
Answer:
Five months’ salary.

Question 17.
How much did Marcus offer the people at first to vote for him?
Answer:
Two shillings to each voter.

Question 18.
How much did Marcus offer the people finally to vote for him?
OR
How many Shillings was finally accepted for the votes by the elders?
Answer:
Four shillings.

Question 19.
Who was the enemy Roof referred to when he spoke to the elders?
Answer:
The Progressive Organisation Party.

Question 20.
Who is the leader of the Progressive Organisation Party?
OR
Who had formed the POP?
Answer:
Maduka.

Question 21.
How much money did the POP offer Roof to vote for Maduka?
OR
How much does Roof get from the representatives of Maduka?
OR
How much did the POP campaign team offer Roof for his vote?
Answer:
Five pounds.

Question 22.
What was the election symbol of the People’s Alliance Party?
OR
What is the symbol used by Marcus in the election?
Answer:
A motor car.

Question 23.
What was the symbol of the Progressive Organisation Party?
Answer:
A man’s head.

Question 24.
What is ‘Umuofia Mansions’?
OR
Where did Marcus Ibe build his big mansion in ‘The Voter’?
Answer:
‘Umuofia Mansions’ is the name of the biggest house in Umuofia, built by Marcus Ibe.

Question 25.
Where, on the election morning, did Marcus Ibe sit shaking hands with the villagers?
OR
Where was Marcus Ibe seated on the morning of the election?
Answer:
On the election morning, Marcus Ibe sat in the ‘owner’s corner’ of his enormous green car, shaking hands with the villagers.

Question 26.
What was Rufus Okeke called as?
OR
Who was addressed as Roof, for short?
Answer:
Rufus Okeke was called Roof for short by the people of his village.

Question 27.
What had Marcus promised the voters if he was elected in the forthcoming election?
OR
Mention the promise made by PAP if they were voted to power.
Answer:
Marcus had promised to give pipe-borne water to the village if he was elected in the forthcoming election.

Question 28.
Who competed against Marcus in the elections?
Answer:
Maduka, the leader of the POP, competed against Marcus in the elections.

Question 29.
What warning did Roof give Marcus about the coming elections?
Answer:
The roof had warned Marcus about the radical change that had come into the thinking of Umuofia since the last national election.

Question 30.
Why did Marcus Ibe join politics?
Answer:
Marcus had wisely joined politics just in time to avoid imminent dismissal arising from a female teacher’s complaint.

Question 31.
What does PAP stand for?
Answer:
PAP stands for the People’s Alliance Party.

Question 32.
What does POP stand for?
Answer:
POP stands for Progressive Organisation Party.

Question 33.
How much did Roof give the villagers to cast their votes for Marcus?
Answer:
The roof gave four shillings to each of the villagers to cast their votes for Marcus.

Question 34.
What was Roof made to swear on to ensure his vote for Maduka?
OR
What was Roof asked to swear on by the POP team?
Answer:
The roof was made to swear on the Iyi of Mbanta to ensure his vote for Maduka.

Question 35.
Who was PAP’s most illustrious son referred to in ‘The Voter’?
Answer:
Marcus Ibe was PAP’s most illustrious son referred to in ‘The Voter’.

Question 36.
Who was the Minister of Culture in the outgoing government in ‘The Voter’?
Answer:
Marcus Ibe was the Chief Honourable Minister of Culture in the outgoing government mentioned in the voter.

Question 37.
Name the man of high traditional title in Umuofia mentioned in ‘The Voter’.
Answer:
Ogbuefi Ezenwa is the man of high traditional title in Umuofia mentioned in ‘The Voter’.

Question 38.
Mention one of the honours/benefits politics had brought to Marcus Ibe in ‘The Voter’.
Answer:
Some of the honours/benefits politics had brought to Marcus Ibe were wealth, chieftaincy titles, and doctorate degrees.

Question 39.
Opposition to Marcus Ibe in ‘The Voter’ was like
(a) a fly trying to move a dunghill.
(b) the bird challenging his personal spirit
(c) a mortar turning its back on the ground.
Answer:
(a) a fly trying to move a dunghill.

Question 40.
Who conducted the whispering campaign in ‘The Voter’?
Answer:
Marcus Ibe’s stalwarts conducted the whispering campaign in ‘The Voter’.

Question 41.
The roof offered _____ shillings initially to the elders for their votes in ‘The Voter’.
(a) four
(b) two
(c) five
Answer:
(b) two.

Question 42.
Where does the iyi come from, according to the POP campaigner, in ‘The Voter’?
Answer:
According to the POP campaigner in ‘The Voter’, the ‘iyi’ comes from Manta.

Question 43.
Whose election symbol was ‘man’s head’ in ‘The Voter’?
Answer:
In ‘The Voter’, ‘Man’s head’ was the election symbol of the Progressive Organisation Party (POP).

Question 44.
What precaution did Roof take while inserting the ballot paper in ‘The Voter’?
Answer:
In ‘The Voter’, while inserting the ballot paper, Roof took the precaution of putting the first half into Maduka’s box.

Question 45.
When did Marcus’s boys conduct whispering campaigns in ‘The Voter’?
Answer:
In ‘The Voter’, Marcus’s boys conducted whispering campaigns at night.

Question 46.
How many elders were there when Roof conducted the whispering campaign in ‘The Voter’?
Answer:
There were five elders besides Roof and his assistant when Roof conducted the whispering campaign in ‘The Voter’.

II. Answer the following questions in a paragraph of 80 – 100 words each:

Question 1.
The roof was a very popular man in his village. Give reasons.
Answer:
Roof Okeke was an energetic young man and had come back to his own village Umuofia, after working as a bicycle repairer’s apprentice for two years in Port Harcourt. It was true that he would have enjoyed a rich life if he had stayed on in his job. But he came back to the village and told the people that he wanted to guide them in difficult times. Later he became an ‘election campaign manager’ for Marcus Ibe and over a period of five years became an expert in election campaigning at all levels. Hence he was a very popular man in his village.

Question 2.
How did the POP campaign team trap Roof?
OR
Describe the meeting held by the POP campaign team with Roof in ‘The Voter’.
OR
Describe the meeting held by the POP campaign team with Roof in ‘The Voter’.
Answer:
In the story ‘The Voter’, Roof is the election campaign manager for Marcus Ibe, a candidate of the People’s Alliance Party. On the night before the election, the leader of the POP campaign team visits Roof’s house. He places five pounds on the floor and tells Roof that he wanted his vote. Initially, Roof did not know what to say or do. But, when he gets up from his chair, closes the door, and comes back, he gets enough time to weigh the proposition. He craves to make such a huge amount, his own.

However, his conscience pricks him and he tells in a feeble voice that the other person knows that he works for Marcus and it will be very bad on his part to accept a bribe, vote for Maduka, and deceive his own master. But when the other person tells him that Marcus will not know who he has voted for, Roof throws away his moral scruples to the wind and asks him whether anyone will talk about him outside. When he assures him that they are only interested in votes and not gossip, Roof’s greed tempts him to accept the offer. But, he also gets trapped. The leader asks him to swear on the Tyi’, that he would vote for Maduka. The roof cannot go back on his promise. So he swears to vote for Maduka.

Question 3.
Describe the last-minute election campaign in Umuofia.
OR
Give a picture of the activities on the election morning.
Answer:
On the day of the election, Chief the Honourable Marcus Ibe was doing things in a grand style. He had hired a highlife band from Umuru and stationed it at such a distance from the voting booths as just managed to be lawful. Many villagers danced to the music, their ballot papers held aloft, before proceeding to the booths. Marcus sat in the ‘owner’s corner’ of his enormous green car and smiled and nodded. Some villagers came up to the car, shook hands with the great man, and said in advance ‘Congrats!’

Roof and the other organizers were prancing up and down, giving last-minute advice to the voters and pouring with sweat. Roof tried to guide illiterate women saying “Our sign is the motor-car”. Then he told them, “Don’t look at the other with the man’s head: it is for those whose heads are not correct”. Finally, he shouted, “Vote for the car and you will ride in it!”

Question 4.
How is Roof’s dilemma brought out in ‘The Voter’?
OR
Roof’s dilemma on the day of the election is the result of his own misdeeds. Do you agree? Give reasons.
Answer:
On the day of the election, we see Roof in a moral dilemma. For the first time, his moral conscience pricks him not to betray Marcus. But it is only a momentary impulse and vanishes almost immediately when Roof recalls the picture of iyi and the cocoa farmer harvesting his crop. Then he hits on an idea of deceiving both Marcus and Maduka. He folds the ballot paper, tears it in two at the crease, and drops the first half into the box meant for Maduka and the other half into the box meant for Marcus. Thus, the writer successfully delineates how ‘money politics’ can corrupt people at all levels. It also reveals the contagious nature of an immoral attitude as the villagers who feel they “have climbed the iroko tree today and would be foolish not to take down all the firewood needed” blatantly ask for bribes.

It is Roof who is responsible for this moral degradation. The fall of Roof demonstrates the deepening level of corruption and the dimming of moral principles. Although Roof overcomes his personal dilemma on election day by tearing his ballot paper in two – one-piece for Maduka, the opponent, and one for Chief Marcus Ibe, his own employer his act illustrates the basic unreliability and political dishonesty of people working for elections. The plot of the story hinging on this man who bribes the electorate and who is bribed in turn drives home the deviant tendency of the political scene.

Question 5.
What changes did politics bring in Marcus Ibe?
OR
Describe how Marcus Ibe in ‘The Voter’ had managed to become a ‘successful’ politician.
OR
Everyone is full of praise for Marcus Ibe. What qualities and achievements attracted the people to praise him?
Answer:
Marcus Ibe was a not-too-successful mission school teacher. When he was almost on the verge of dismissal on the basis of a female teacher’s complaint, he joined politics, got elected as a representative of Umuofia, and was made Minister of Culture. People referred to him as Chief the Honourable Minister of Culture. In a period of five years Marcus became very wealthy, was given many chieftaincy titles and doctorate degrees and several other honours.

He got a huge mansion built in Umuofia and named it ‘Umuofia Mansions’ and got it opened by the Archbishop. On the day of the opening, he hosted a grand lunch to the people of his village slaughtering five bulls and countless goats. After feasting in his house, people were full of praise for him. They opined that he was a great man and does his things like a great man. He also owned two big cars.

Question 6.
Account for Roof’s popularity in ‘The Voter’.
OR
The roof was a very popular man in his village. Give reasons.
Answer:
Rufus Okeke, called Roof for short, was a very popular man in his village. His popularity was due to the fact that even after spending two years as a bicycle apprentice in Port Harcourt, Roof had come back to Umuofia of his own free will with the intention of guiding his people in difficult times. If he had stayed on in his job he would have enjoyed a rich life. By this gesture, he wins the gratitude and admiration of his people.

Question 7.
What was the whispering campaign and how did Roof conduct it in ‘The Voter’?
Answer:
A ‘whispering campaign’ refers to secret meetings held between the group of elders of a village and the election campaign manager of a political party. The election manager goes to such campaigns armed with money bags and woos the voters offering money for votes.

In ‘The Voter’, Roof, the most trusted campaigner of the PAP, conducted a whispering campaign in the house of Ogbuefi Ezenwa, a man of the high traditional title. Initially, Roof tried to kindle the pride of the people telling them that the PAP had made Marcus Ibe, one of their own sons, Honourable Minister of Culture in the outgoing government, and offered them 2 shillings each initially. But, Ezenwa rejected it saying that two shillings were shameful and Marcus was a great man, who did his things like a great man. Later, when Roof offered them 2 more shillings each, they accepted it and agreed to cast their vote for Marcus Ibe. Thus, Roof conducted his whispering campaign.

Question 8.
Why was Roof in a fix when putting in his ballot paper, in ‘The Voter’?
Answer:
As soon as Roof went into the voting booth, he was confronted by the ‘car’ on one box and the ‘head’ on the other. He took out his ballot paper from his pocket and looked at it. For a moment he found himself caught in a moral dilemma. He could not decide whether he should vote for Marcus Ibe of the PAP or Maduka of POP.

As the election campaign manager for Marcus Ibe, he had worked enthusiastically urging the people to vote for PAP. He had also taken a lot of money and other gifts for his service. But, now he had put himself in a fix because he had accepted five pounds from the POP and had sworn before the ‘iyi’ that he would vote for Maduka. So he did not want to cheat, because his fear of ‘iyi’ from Mbanta troubled him seriously. On the other hand, he felt guilty for betraying Marcus Ibe. For a moment he felt like going back to the campaign manager and returning his five pounds.

Finally, the moment he thought of the red notes, he visualized the cocoa farmer busy at work and his swearing on that ‘iyi’. Therefore, he folded the ballot paper, tore it into two along the crease, and put one half in each box. He put the first half into Maduka’s box confirming his action verbally, “1 vote for Maduka”.

Question 9.
Trace the behaviour of Roof on the day of voting.
Answer:
On the day of the election, Roof appears to be seriously interested in ensuring that the voters know who to vote for and how to exercise their choice. Along with others Roof prances up and down giving last-minute advice to the voters. As soon as the first rush of voters is over, Marcus promptly asks his campaign boys to go one at a time and put in their ballot papers. He asks Roof to go first. Roof’s spirits fall, but he lets no one see it. Roof dashes off in his springy fashion towards the booth.

When Roof goes into the booth, he faces a moral dilemma. But he overcomes his moral dilemma, cuts the vote into two, and drops the two halves in the boxes, one half for Marcus and the other half for Maduka. While coming out, he looks as happy as he was when he went in. Thus, by putting on a happy face and walking with a lot of energy in his gait while going into the booth as well as while coming out of the booth, Roof is able to conceal his betrayal of Marcus in the election.

III. Answer the following questions in about 200 words each:

Question 1.
Do you think Roof is right in tearing the ballot paper into two? Explain.
OR
Do you agree or disagree with how Roof resolves his dilemma regarding how to cast his
vote?
OR
In the story, Roof has to make a difficult decision about how to cast his vote. Do you agree with the way he resolves this dilemma? Why or why not?
OR
Why does Roof tear the ballot paper into two?
Answer:
The main focus of Achebe in ‘The Voter’ is the satirisation of money politics and the contagious nature of corruption. Roof, the protagonist of the story, is an energetic young man of Umuofia, who has come back to his village giving up a bright future in the city. People believe that he has come back to guide them in difficult times and so hold him in high esteem.

The village Umuofia already belonged en masse to the People’s Alliance Party and had elected Chief the Honourable Marcus Ibe as their representative in the previous election and nobody doubted his re-election this time also. The man behind Marcus’ success is Roof. He was his election campaign manager and had become a real expert in election campaigning at all levels.

But towards the end of the story, we see that Roof is offered five pounds by the leader of the election campaign for a POP on condition that Roof votes for Maduka. A roof does not hesitate at all but tries to tell them that he is working for Marcus. But they allay his fears telling him that Marcus will not be there to watch him when he drops his vote in the box. Roof accepts their offer and swears to vote for Maduka in the presence of ‘iyi’.

On the day of the election, Marcus wants to make sure that every one of his people votes for him. Therefore, he asks Roof to cast his vote first. When Roof goes into the booth, he faces a moral dilemma. His conscience tells him that he ought not to betray Marcus. There is an impulse in him to run to the leader of the POP election campaign manager and return his money. But, when he recalls that he had sworn to vote for Maduka in the presence of ‘iyi’, he changes his decision in a split second and folds the ballot paper in two. He tears it into two halves and drops the first half into the box meant for Maduka and the other half into Marcus’ box. He also confirms his vote for Maduka by saying aloud, “I vote for Maduka”, and then goes out happily.

This act of Roof, of tearing the ballot in two, invalidates his vote. When a person tears it in two, it is clear that he has not chosen any person at all, though he may have dropped the two halves of the paper into different boxes. When Roof tears the ballot paper in two it is only to resolve the moral dilemma he is facing and not with the intention of exercising his choice. If he puts two halves in two different boxes, it does not mean that he has voted in favour of both. Actually it is an invalid vote. This is against the spirit of elections and is condemnable.

Question 2.
How does Roof conceal his betrayal of Marcus in the election? Elucidate.
Answer:
On the day of the election, Roof appears to be seriously interested in ensuring that the voters know who to vote for and how to exercise their choice. Along with others Roof prances up and down giving last-minute advice to the voters and pouring with sweat. As soon as the first rush of voters is over, Marcus promptly asks his campaign boys to go one at a time and put in their ballot papers. He asks Roof to go first. Roof’s spirits fall, but he lets no one see it. Roof dashes off in his springy fashion towards the booths. When Roof goes into the booth, he faces a moral dilemma.

But he overcomes his moral dilemma, cuts the vote into two, and drops the two halves in the boxes, one half for Marcus and the other half for Maduka. While coming out, he looks as happy as he was when he went in. Thus, by putting on a happy face and walking with a lot of energy in his gait while going into the booth as well as while coming out of the booth, Roof is able to conceal his betrayal of Marcus in the election.

Question 3.
Give an account of Roof’s role as an election campaigner.
OR
The roof is an expert election campaigner. Substantiate the statement.
Answer:
The roof is an energetic young man of Umuofia, who has come back to his village giving up a bright future in the city. People believe that he has come back to guide them in difficult times and hence hold him in high esteem. When we read about the election, the writer tells us that the village of Umuofia already belonged en masse to the People’s Alliance Party and had elected Chief the Honourable Marcus Ibe as their representative in the previous election and nobody doubted his re-election this time also. The man behind Marcus’ success is Roof.

The roof was his election campaign manager and had become a real expert in election campaigning at all levels. Having already seen a change in Marcus’ status and wealth, Roof is able to gauge the people’s mood and the change in people’s perception of Marcus Ibe and had conveyed to his leader that a radical change had come in them. This way he prepares Marcus to plan his strategy to woo the voters in the coming elections.

After feasting in Marcus’ house on the day of the opening of his new house, people had realized what good things politics had showered on Marcus. They also regretted to a little extent that they had given their vote to Marcus free of charge in the previous election. Therefore, this time, they decided to demand a price for their vote. As expected, Roof, being an expert in election campaigning, comes armed with money to his whispering campaigns.

During his whispering campaigns, Roof uses a lot of political rhetoric and tells the people convincingly that PAP favours the people of Umuofia. He says, “What greater honour can a village have? Do you ever stop to ask yourselves why we should be singled out for this honour? Think of the pipe-borne water they have promised us …” This way Roof manages to mesmerize the naive people into believing that Marcus is a great man and is going to do great things for their village.

Besides, Roof satisfies their desire for a share of Marcus’ earnings by giving them four shillings each. On the day of the election, Marcus entertains the people hiring a highlife band from Umuru. He sits in an enormous car and tries to impress the people with his wealth and eminence. He coins catchy slogans like ‘Vote for the car, and you will ride in it’. It is these strategies planned by Roof that ensure Marcus’ victory in the elections.

Question 4.
‘The Voter’ ridicules the power and selfishness of Marcus Ibe. Explain.
Answer:
‘The Voter’ highlights what kind of people become people’s leaders, how politics bestows wealth and power on them, and because of such people how elections become farcical. The two main characters in the story are Marcus and Roof, and the voters are the people of Umuofia. The whole focus of the plot is to ridicule greedy and selfish politicians and to show how they manage to corrupt the society also.

Marcus was a not-too-successful mission school teacher. When he was almost on the verge of dismissal on the basis of a female teacher’s complaint, he joined politics, got elected as a representative of Umuofja, and was made Minister of Culture. In about five years, people see a great many changes in Marcus’ life. Their elected representative becomes wealthy, is awarded chieftaincy titles and doctorate degrees besides many other honours. He also builds a huge mansion in his native village.

In a satirical tone, the writer says that Marcus had christened his new house ‘Umuofia Mansions’ in honour of his village and had slaughtered five bulls and countless goats to entertain the people on the day it was opened by the Archbishop. Marcus believed that, by entertaining people thus, people would respect him and would hold him in high esteem.

But the people are not naive and ignorant. They know where his ‘power’ and money come from. The people know that he had acquired all this only after they had elected him their representative giving their votes free of charge five years ago. Even after five years, the people and their village remained the same as before but Marcus had changed completely.

When Roof tells Marcus that a radical change had come into the thinking of Umuofia since the last election, like any other corrupt politician Marcus prepares himself to bribe the people. The people come prepared to demand their share of his wealth. Instead of demanding developmental work for their village, the people tell Roof that two shillings are too shameful and settle the deal for four shillings each. It is Marcus and Roof who blatantly offer money, tacitly admitting that they have used the public money selfishly for their own development, that the voter ridicules them by demanding more money for their vote, sending the message that real power to change the face of a village, town or country is in the hands of the voter and not selfish politicians like Marcus.

Question 5.
‘Roof was the most trusted of the whispering campaigners’. To what extent is this true in ‘The Voter’?
OR
To what extent was Roof the most trusted of the whispering campaigners in ‘The Voter’?
Answer:
When the story opens, we learn that Marcus is seeking re-election as a representative of Umuofia. Secondly, we also know that it was Roof who had worked for his success in the previous election. As a trusted whispering campaign manager of Marcus, he had been able to convince the people of Umuofia that Marcus would work for the welfare of the people and they would get many amenities like running water and electricity. Then, he had worked hard to get Marcus elected and had kindled the expectations of the people.

But, during the five years as people’s representative, Marcus proved to everyone how joining politics can be lucrative. He had also given gifts to Roof to retain him as his election campaigner for the coming election also. However, Roof was clever enough to perceive a change in the mood of the people and warned Marcus about it. That is why, in the whispering campaign, he goes armed with money bags to woo the voters.

Until the last day of the campaigning, Roof remains loyal and the most trusted of Marcus’ whispering campaigners. But, the visitor from POP cleverly lures Roof to promise his vote for Maduka offering a huge sum of money. It is at this juncture that we see a dent in his loyalty towards Marcus. Even though he knows that a half ballot paper is invalid as a person’s mandate, he tears the vote in two and puts one half into each box, thus resolving the moral dilemma in his mind. This way he is only comforting his conscience and does not remain functionally loyal to Marcus.

Question 6.
Do you think ‘the voter’ is right in demanding money to vote for Marcus Ibe? Explain.
Answer:
The people of Umuofia give their mandate to Marcus and get him elected as their representative believing that he would work for their welfare and upliftment. However, as his term rolls by, they witness a substantial change in Marcus’ status and wealth, while the people’s status remains the same. People realize how politics can make someone rich and powerful. They regret that they had underrated the power of their mandate, and so decide to demand a share of his earnings to cast their vote.

That is why, in the whispering campaign, when Roof offers each one of the two shillings, they tell him that it was too shameful to accept just two shillings from Marcus for their vote because Marcus was no longer a poor man and was doing great things like a great man. Then they agree to cast their vote taking four shillings each.

An election process in any democratic set up has high intrinsic value because it symbolizes people’s mandate and the elected representative becomes the voice of those people. Naturally, when the voters of Umuofia demand money to vote for Marcus, it only means that their real mandate has not given them what they needed and they remain in abject poverty as before. They have lost faith in elections as a means to achieve their welfare. The previous election may have failed them but they ought not to become corrupted. That will pave the way for further deterioration by building obstacles in the path to their own development. They are not right in demanding money.

Question 7.
‘The Voter’ is a comment on the awareness of voters. Discuss.
Answer:
In the story ‘The Voter’, Chinua Achebe satirizes ‘politicians’ and makes an attempt to show how even in a democratic setup election can become farcical because of ignorance, illiteracy, and corruption. The story also highlights how deep-rooted corruption in society can be.

Marcus Ibe, who was once a local mission school teacher, was now Chief the Honourable Minister of Culture in the outgoing government. During his term as a minister, he had amassed a lot of wealth. Thus, ‘politics’ had brought about a great change in the life of Marcus and Roof as well. But, the people of Umuofia had remained the same – poor, illiterate, ignorant, and naive – and their villages remained dry and wretched without even the basic amenities.

Marcus, who is seeking a second term, is sure that he is going to get a landslide victory because of the absence of any strong opposition party worth taking into consideration. But, this time, there is a change in the perception of the people. After feasting in Marcus’ new house and noticing the increase in his wealth and power, the poor people of Umuofia realize that elections do have a meaning and it is during elections that their votes have value.

They also know that it is ‘politics’ that has brought wealth and power to Marcus. They strike a bargain with Roof and agree to sell their vote for four shillings each. This way, the people of Umuofia shed their moral scruples and make up their minds to be satisfied with just a little share of the thief’s booty. Eventually, it is this strain in the people’s minds that helps a corrupt politician like Marcus to win elections with the least resistance.

Like Marcus, Roof also realizes how people’s elected representatives can become rich in a very short time. Having worked as his election manager, Roof extracts enough money as well as other benefits from Marcus during his first term. In the second term. Roof’s greed for wealth is kindled by another political party – the POR They know that it is Roof’s expertise in election campaigning that woos the voters of Umuofia and Roof is amply compensated by Marcus for his efforts.

The POP, with a view to making a dent in Marcus’ electorate, lures Roof with an offer of five pounds just to cast his vote for Maduka. Their intention is only to win over Roof to their side with the’ promise that he can make much more money from POP than from PAP. Once Roof bites the bait, they ensure that he is in their clutches, by forcing him to swear on the ‘iyi’. Though Roof is caught in a dilemma, he solves the conflict in his mind by tearing the ballot paper into two and casting his vote for both parties.

Thus, both the episodes prove how ‘corruption’ can ruin both the literate and the illiterate, the educated and the uneducated, and make democratic processes ineffective and farcical. The voters, who have been given the power to elect a leader who can help them improve their lives, use the very process of election to settle for a pittance and build obstacles in the path to their own development. This way they make a mockery of the electoral system.

Question 8.
Demanding money to vote for a particular person is not wrong. How is this brought out in ‘The Voter’?
Answer:
‘The Voter’, a short story by Chinua Achebe, happens in a village called Umuofia. It is a satirical and realistic portrayal of how corrupt politicians deceive people in elections and what mediatory strategies they use to woo the voters and subvert the power of the people’s mandate in choosing a leader df their choice. Incidentally, the author also seems to question the very basis of societal and ethical norms, socio-economic conditions of the people, moral decadence, etc. The corrupt and greedy political leader in the story is Marcus Ibe.

He is the Chief Honourable Minister of Culture in the outgoing government and is seeking re-election as a candidate of the PAR Soon after becoming the minister, Marcus had made use of his political status for his self-aggrandizement. In a period of five years, he had managed to amass a great deal of wealth, power, status, and public adulation. He had two long cars and had just built himself the biggest house in those parts. To top it all, on the day of the opening of his mansion, Marcus Ibe held a grand banquet for the people of the village slaughtering five bulls and countless goats. Thus, politics had made him richer.

On the other hand, the status of the people remained the same. They lived in abject poverty without even the basic amenities like electricity and drinking water. Though they had been watching how politics had brought wealth and power to Marcus, it had not dawned on them that it was the power of their vote which had given him all the comforts. After the feasting was over, the villagers told themselves that they had underrated the power of the ballot paper before and should not do it again. His election manager Roof was shrewd enough to understand the change in the attitude of the people. Therefore, he went to the whispering campaign armed with money and successfully bought their vote paying four shillings each to the leaders.

The question that arises now, is, whether demanding money to vote for a particular person is right? The author seems to argue that as long as politicians in a democratic set up fulfill their promises to the people and take care of their welfare, the question of buying votes does not arise at all. When politicians themselves flout all democratic norms and ideals and work only for their aggrandizement, there is nothing wrong with people demanding some value for their votes. This is brought out clearly in the story.

Ezenwa, the highest traditional title holder, says, “Marcus is a great man and does his things like a great man. We did not ask him for money yesterday; we shall not ask him tomorrow. But today is our day; we have climbed the iroko tree today and would be foolish not to take down all the firewood we need”. Thus, one can rightly conclude that demanding money to vote for a particular person is not wrong.

Question 9.
‘The Voter’ presents the role of money and ridicules its power in an election. Explain.
Answer:
‘The Voter’, a short story by Chinua Achebe, happens in a village called Umuofia. It is a satirical and realistic portrayal of how corrupt politicians deceive people in elections and what mediatory strategies they use to woo the voters and subvert the power of the people’s mandate in choosing a leader df their choice. Incidentally, the author also seems to question the very basis of societal and ethical norms, socio-economic conditions of the people, moral decadence, etc. The corrupt and greedy political leader in the story is Marcus Ibe. He is the Chief Honourable Minister of Culture in the outgoing government and is seeking re-election as a candidate of the PAR Soon after becoming the minister, Marcus had made use of his political status for his self-aggrandizement.

In a period of five years, he had managed to amass a great deal of wealth, power, status, and public adulation. He had two long cars and had just built himself the biggest house in those parts. To top it all, on the day of the opening of his mansion, Marcus Ibe held a grand banquet for the people of the village slaughtering five bulls and countless goats. Thus, politics had made him richer.

This is brought out clearly in the story. Ezenwa, the highest traditional title holder, says, “Marcus is a great man and does his things like a great man. We did not ask him for money yesterday; we shall not ask him tomorrow. But today is our day; we have climbed the iroko tree today and would be foolish not to take down all the firewood we need”. Thus, one can rightly conclude that demanding money to vote for a particular person is not wrong.

The Voter Vocabulary

Use suitable prefixes to form antonyms:
A prefix is an affix which is placed before the stem of a word. Adding it to the beginning of one word changes it into another word.
Prefix – In-, Un-, Im-, Dis-

Question 1.

Gratitude
Certain
Install
Personal
Honour
Disputed
Correct
Mask
Lawful
Grateful
Wavering
Regard
Perturbed
Tolerant.

Answer:

Ingratitude
Uncertain
Uninstall
Impersonal
Dishonour
Undisputed
Incorrect
Unmask
Unlawful
Ungrateful
Unwavering
Disregard
Unperturbed
Intolerant.

Additional Exercises

A. Passive Voice:

Question 1.
The roof was a young man. He ______ (know) to everyone in Umuofia. The POP campaigner met him at night. No words _____ (waste) between them. Roof _____ (give) five pounds to vote for Maduka.
Answer:
was known; were wasted; was given.

Question 2.
Marcus Ibe was the PAP candidate. He ______ (warn) about the radical change in the thinking of Umuofia. So five months’ salary _______ (draw) in advance. His campaign boys _____ (arm) with eloquent little jute bags.
Answer:
was warned; was drawn; were armed.

Question 3.
Marcus Ibe was extremely generous to Roof. He ______ (ask) to lend one of his many robes by Roof. When Marcus Ibe’s wife objected, she ______ (rebuke) publicly by him. Roof won a land case because he _____ (drive) by a chauffeur to the disputed site.
Answer:
was asked; was rebuked; was driven.

Question 4.
The roof received a strange visit from the POP campaigner. Though the campaigner and Roof ______ (know) to each other, his visit was cold and business-like. No words _____ (exchange) between them. When the visitor placed five pounds on the floor, Roof ______ (mesmerize) by the picture of the cocoa farmer.
Answer:
were known; were exchanged; was mesmerized.

Question 5.
Marcus was doing things in a grand style. A high life band from Umuru ______ (hire) by him and it ______ (station) at a distance from the voting booth. Marcus sat in his car when last-minute advice ______ (give) to voters by Roof and others.
Answer:
had been hired; was stationed; was given.

Question 6.
The man nudged his companion and he brought forward an object that ______ (cover) with a red cloth. It was a fearsome little affair. It _____ (keep) in a clay pot and feathers ______ (stick) into it.
Answer:
was covered; was kept; were stuck.

B. Report the following conversation:

Question 1.
Roof: I work for Marcus Ibe.
POP campaigner: We have plenty of work to do tonight Are you taking this or not?
Roof: Will it not be heard outside this room?
POP campaigner: We are after votes, not gossip.
Answer:
Roof informed the POP campaigner that he worked for Marcus Ibe. The POP campaigner remarked that they had plenty of work to do that night. He further asked Roof whether he was taking that or not. Roof asked cautiously whether it would be heard outside that room. The POP campaigner stated that they were after votes and not gossip.

Question 2.
Roof: Do not forget. Our sign is the motor-car.
Woman: Is it like Marcus’s car?
Roof: It is the same car. Don’t look at the other with the man’s head.
Answer:
Roof implored the woman not to forget that their sign was the motor-car. The woman wanted to know whether it was like Marcus’s car. Roof replied that it was the same car. He also asked the woman not to look at the other with the man’s head.

Question 3.
POP campaigner: The iyi comes from Mbanta. Swear on it.
Roof: I will cast my paper for Maduka. But Maduka has no chance against Marcus.
POP campaigner: Maduka gives out pounds, not shillings.
Answer:
The POP campaigner informed Roof that the iyi came from Mbanta. He asked him to swear on that. Roof told him that he would cast his paper for Maduka. But he added that Maduka had no chance against Marcus. In response, the POP campaigner remarked that Maduka gave out pounds, and not shillings.

C. Fill in the blanks by choosing the appropriate expressions given in brackets:

Question 1.
Marcus knew that he would win but didn’t want _______ a single vote. So he asked his campaign boys to go one at a time and put in their ballot papers. At this time Roof was weighed down by guilt but pretended ______. (to be in high spirits, to throw away, pass by)
Answer:
to throw away; to be in high spirits.

Question 2.
The roof was ______ as he had sworn on iyi that he would vote for Maduka. However, on the day of the election, he wanted to hide it. Therefore he did not ______ his calm and confidence. (give up, face lit up, in a fix)
Answer:
in a fix; give up.

Question 3.
In Umuofia, everyone was _____ for Marcus Ibe. He was not like the mortar which as soon as food comes its way ____ on the ground. (looks down at, turns it is back, full of praise)
Answer:
full of praise; turns its back.

Question 4.
It was the time of the election. The campaign in Umuofia was ____. All knew that the honourable minister would have a _____. (in a soup, landslide victory, in full swing)
Answer:
in full swing; landslide victory.

Question 5.
The roof was disturbed on the morning of the election. Suddenly a thought _______ his mind and he _______ in his springy fashion towards the booth. (leapt into, gave out, dashed off)
Answer:
leapt into; dashed off.

Question 6.
Roof’s heart nearly ______ when he saw the iyi. Indeed he knew the fame of Mbanta in these things. What could a single vote cast in secret for Maduka ______ from Marcus’s certain victory? (get a few, few out, take away)
Answer:
flew out; take away.

D. Fill in the blanks with the appropriate linkers:

Question 1.
The roof was a young and energetic man. He never left his village _______ to seek work in the towns _____ decided to remain to guide his people. _____ he was very popular. ______ he was an expert in election campaigning. (moreover, but, in order to, so)
Answer:
in order to; but; So; Moreover.

Question 2.
Everything was moving according to the plan. ______ Roof had received a strange visit from the leader of the POP campaign team. ______ he and Roof were well-known ______ might even be called friends, the visit was business-like. He placed five pounds on the floor before Roof. ______ Roof spoke, his eyes never left the red notes on the floor. (and, although, then, as)
Answer:
Then; Although; and; As.

Question 3.
The roof was a very popular man in his village. ______ the villagers did not explain it in so many words, Roof’s popularity was a measure of their gratitude to an energetic young man ______ unlike most of his fellows nowadays, had not abandoned the village _____ seek any work in the towns. And Roof was not a village lout ______. (who, although, either, in order to)
Answer:
Although; who; in order to; either.

Question 4.
Roof’s heart nearly flew out _____ he saw the iyi ______ he knew the fame of Mbanta well. ____ he was certain that a single vote would not defeat Marcus. _____ he agreed to swear on the iyi. (hence, when, however, as)
Answer:
when; as; However; Hence.

The Voter by Chinua Achebe About the Writer:

Chinua Achebe (1930-2013) is a Nigerian novelist, poet, professor, and critic. Achebe’s novels focus on the traditions of Igbo society, the effect of Christian influences, and the clash of Western and traditional African values during and after the colonial era. His style relies heavily on the Igbo oral tradition and combines straightforward narration with representations of folk stories, proverbs, and oratory. He also published a number of short stories, children’s books, and essay collections. Some of his works are: ‘Things Fall Apart’, ‘Arrow Of God’, ‘No Longer At Ease’,’ Enemy Of The People’ and ‘Girls At War’.

The Voter Summary in English

In this short story, Achebe offers a satirical picture of how politics and elections generally work in modern African countries like Nigeria.

The protagonist of the story is Rufus Okeke – Roof for short. The majority of the Igbo people in the village are illiterate, backward, and poor. Though the administration of the town is in the hands of a democratic government comprising elected representatives of the people, the people have not yet realized the value of their mandate and are also not yet aware of their rights. It is election time now. The ruling party PAP (People’s Alliance Party) is seeking re-election. There is hardly any other party to obstruct or prevent the re-election of the existing government. One important aspect of this election is the re-election of Marcus Ibe, who is Minister of Culture in the outgoing government. The only other party, which appears to be a non-entity until now, is the POP – Progressive Organisation Party, and Maduka is its representative. The POP is making a strong attempt to garner some votes in its favour.

The focus of the author seems to be to capture the human drama in which a poor, pathetically innocent and naive people make a brave attempt to derive some monetary benefit during the election from a prospective candidate using the mediatory strategies of a literate young man of their town in when they have reposed a great deal of trust.

However, the crux of the plot is how this very same trusted representative of the people manages to strike a rich bargain with the opposition party as well and yet remain loyal to both the parties.

When the story opens, we are introduced to Rufus Okeke. He is a very popular man in the village. His popularity is due to the fact that unlike people of his age, Roof has not abandoned his village seeking work in the towns. Secondly, he is also not a village lout. People like him because they believe that he has given up a bright future and come back to their village on his own after spending two years as a bicycle repairer’s apprentice in Port Harcourt with the intention of guiding the people of the village.

In the next stage, the author tells us about the voters and the government. We learn that the whole village had voted en masse in favour of the People’s Alliance Party and elected Honourable Marcus Ibe of their village, who had become Minister of Culture in the outgoing government. The author tells us in a satirical tone that Marcus Ibe was sure to be re-elected because there was hardly any other opposition party worth considering. From this situation, the reader can infer the predicament of the innocent people pitted against greedy and power-thirsty politicians. In a tone veiled in mild satire, the author says that Roof, the trusted representative of the people of Umuofia, was working as the election campaign manager for the Honourable Minister Marcus Ibe, who was seeking re-election from Umuofia.

2nd PUC English Textbook Answers Springs Chapter 12 The Voter image - 1

The roof was more intelligent and cleverer than the common people of Umuofia. He had become a real expert in election campaigning at all levels – village, local government or national, that is why he was able to gauge the mood and temper of the electorate at any given time. This time, he has been intelligent enough to warn Marcus Ibe that a radical change has come into the thinking of the people in Umuofia since the last election. Thus he kindles the interest of the reader.

The villagers had come to realize that in five years, politics had brought wealth, chieftaincy titles, doctorate degrees and other honours readily to the man whom they had given their votes free of charge five years ago. It is also a paradox that the people who had empowered a person to enjoy such benefits themselves remained poor and ignorant. They did not even know that a doctorate degree holder is not a medical doctor. Anyhow, the people were now ready to try the value of their votes in a different way.

The author then narrates the expectations of the people in Umuofia. The people had now witnessed the ‘good’ things done by politics to their own elected representative Marcus Ibe. Before getting elected, he was only a fairly successful mission school teacher and was on the verge of getting dismissed on the basis of a female teacher’s complaint. Just at that moment, politics had come to their village and at that opportune moment Marcus Ibe had wisely joined up. By doing so he had escaped dismissal.

Secondly, he got elected and became ‘Chief the Honourable’ in the government. Consequently, he got two long cars and had built himself the biggest house in that village. But, he remained a devoted leader of his people. In a satirical tone, the author says that whenever he could, he left the good things of the capital and returned to his village which had neither running water nor electricity, but he had lately installed a private plant to supply electricity to his new house in the village. The writer comments that Marcus knew the source of his good fortune hinting that it is the people of Umuofia who are responsible for his prosperity.

Marcus Ibe had christened his new house ‘Umuofia Mansions’ in honour of his village and on the day the house was opened, he had hosted a grand lunch to his people slaughtering five bulls and countless goats. Moreover, the house was opened by the Archbishop. Thus the writer gives a rosy picture of the eminence and prosperity of Marcus which politics had bestowed on him.

The author describes the reactions of the people of Umuofia after they had enjoyed Marcus’s hospitality. There is subtle irony in the description. The people are full of praise for Marcus’ hospitality. But, they also know that Marcus owes his riches to his getting elected and joining the government. We can perceive a tone of regret when the people conclude after the feasting was over that they had underrated the power of the ballot paper earlier and that they should not do it again. This is the radical change in people’s attitude towards casting their ballot paper in an election free of charge without expecting any benefits.

Since Roof had already warned Marcus Ibe about it, Marcus had also taken suitable measures to meet the expectations of the people. “He had drawn five months’ salary in advance and changed a few hundred pounds into shining shillings and had armed his campaign boys with eloquent little jute bags”. Having sensed that the people of Umuofia will not cast their ballot paper in his favour, free of cost, Marcus had sent money to bribe and persuade the voters to vote for him. The contesting candidate would make his speeches in the morning and at night his expert election managers would conduct their whispering campaign.

Here ‘whispering campaign’ refers to the way election managers visit voters’ houses in the evening after sunset and tell the voters about the prospect of their candidate becoming minister. Thereby they appeal to people’s self-esteem and the honour that is going to be bestowed on their town and finally bribe them into casting their vote in favour of their political party.

We see how the radical change in people brings about a change in the nature of the election process and affects the sanctity of the people’s mandate.

We witness a whispering campaign conducted by Roof in the house of Ogbuefi Ezenwa, a man of the high traditional title. Roof addresses a group of elders and tells them that his party PAP has made a man of their village a minister in the outgoing government. Roof tries to argue that it is a great honour for one of their sons to be singled out for this honour. Then he tells them that PAP leaders look upon Umuofia with a favourable attitude and whether they cast their vote in his favour or not, PAP will form the government. He also tries to hint at the promise made by PAP to the people of Umuofia that they will give pipe-borne water to their village.

After Roof had finished talking, Ogbuefi Ezenwa spoke to Roof. He tells him that they believe as true every word he has said and every one of them would cast his vote for Marcus. He also promises to get their wives’ votes too in his favour. But, he then tells him straightaway that it is shameful to accept two shillings for their vote. He then says that if Marcus were a poor man they would give their vote free as they had done it before. Then the old leader argues that Marcus is a great man and does his things like a great man. Then he tells Roof that they did not demand money before and they will not ask him in future.

The writer also uses the same language to mock at Roof. He says that Roof had also lately been taking down a ‘lot of firewood’ from Marcus. The previous day, he had taken a rich robe from Marcus. Moreover, Marcus himself had rebuked his wife when she objected to Roof taking his fifth bottle of beer from the refrigerator. Furthermore, Roof had been chauffeur-driven to the disputed site, about a land case which he won. Having enjoyed all such benefits from Marcus, Roof understood the demands of the elders. Therefore, he finally drops two more shillings in front of each one of them and tells them in a tone of finality that he is through with it, and pretends to be defiant. Then he ends his campaign with the sentence, “Cast your paper for the enemy if you like!” The elders quickly calm him down with a placatory speech agreeing to vote for Marcus, and pick up the coins on the floor, with a feeling that they have not lost their decorum and dignity in the bargain.

The author has until now introduced the reader to the existing situation. Now he is going to talk about the opposition party. The author here satirises how opposition parties come into being and how sincere they are in their objectives and in their fighting strategies.

In his ‘whispering campaign’, Roof had asked the elders, in the end, to give their vote to the enemy. The enemy of PAP was a new party called the Progressive Organisation Party (POP). It had been formed by the tribes down the coast. The founders of the party claimed that they had founded the party to save themselves from “totally political, cultural, social and religious annihilation”. The party organizers (of POP) knew that they had no chance of winning against the PAP, yet they had taken the plunge for a straight fight with PAP. They had provided cars and loudspeakers to a few rascals and thugs to go around and make a lot of noise. The author hints that they had also spent a lot of money in Umuofia. The writer comments that such money will only make the local campaigners very rich.

The action now reaches the climax. The writer tells the reader that as the election day was approaching nearer, for Roof “everything was moving as planned”.

One evening the leader of the POP campaign team comes to visit Roof. Although they were well known to each other, his visit is cold and businesslike. He places five pounds on the floor before Roof and tells him that they want his vote. Roof immediately gets up from his chair, closes the outside door carefully and comes back to his chair. Within that short time Roof has weighed the proposition. Roof tries to give a reason to the other person for not accepting his proposition. Roof tells him that he was working for Marcus and it would be very bad to accept it. But the other person had come prepared for such an answer. So he tells him that Marcus would not be there when Roof puts his ballot paper in the box. Then, in a dismissive tone, he tells Roof that they have plenty of work to do that night, and asks him whether he is going to accept it or not.

Roof asks him whether anyone would talk about it outside. The other man tells him categorically that they wanted votes and not gossip. Roof accepts the money. Then they get Roof to swear that he would vote for Maduka in front of a little object called ‘iyi’, which had been brought from Mbanta. However, Roof does not hesitate but says aloud that he would cast his vote for Maduka, failing which the ‘iyi’ will take note. The other man is satisfied and leaves. Before he leaves Roof tells him that Maduka has no chance of winning against Marcus. But the other person tells him that it would be enough if he gets a few votes that time, and he “would get more in the following election. All that they wanted was to make the people know that Maduka will give pounds and not shillings”.

The narrator describes the town and the mood of the people. We learn that Chief the Honourable Marcus Ibe was doing things in grand style. He hires a highlife band from Umuru and stations it at a distance considered lawful. Many villagers dance to the music before proceeding to the booths. Some people shake hands with the great man ‘Marcus’ and congratulate him in advance. Roof and his campaign boys give last-minute advice to the people and try to win Marcus’ appreciation. The writer then tells us that Marcus was a stickler for details. He wants to ensure that not a single vote goes to the other party. Therefore, as soon as the first rush of voters is over, he promptly asks his campaign boys to go one at a time and put in their ballot papers. He asks Roof to go first.

Roof dashes off towards the booths without any hesitation. After the electoral officer has explained to him about the two boxes, he goes in and sees the two boxes one of which has the picture of the car and the other, the head. Roof brings out his ballot paper and looks at it. He does not like to betray Marcus even in secret. For a few seconds, he feels like going back to the other man and returning his five pounds. Then he realizes that it is impossible because he has sworn on that ‘iyi’. Then he recalls the red five-pound notes. Roof’s mind works quick as lightning. He folds the paper, tears it in two along the crease and puts one half in each box. He puts the first half into Maduka’s box and says to himself aloud, “I vote for Maduka”, and comes out. The election officials mark his thumb with indelible purple ink and he walks out of the booth as jauntily as he has gone in.

The Voter Summary in Kannada

2nd PUC English Textbook Answers Springs Chapter 12 The Voter image - 2

Glossary:

Port Harcourt: the capital of Rivers State, Nigeria.
Umuofia: one of a fictional group of nine villages in Nigeria, inhabited by the Igbo people
en masse: in one group or body; altogether
ozo feast: highest and most important magico-religious festival in the Igbo clan of Nigeria.
iroko tree: is a large hardwood tree from the west coast of tropical Africa; it is a tree which grows very high and which is very difficult to climb but which yields very good quality timber; Native deity; something with magical powers – anyone swearing an oath on an iyi would be afraid to violate his oath because if he did so he would be cursed and punished terribly
landslide victory: a victory by a large margin; a very substantial victory, particularly in an election
mesmerize: to hold the complete attention bf; spellbind
a village lout: a rude, young man (derogatory); a simpleton
chieftaincy titles: titles given to important people in a village
naivety: without the experience of social rules or behaviour
eloquent: expressing or showing something very strongly without words
the host: the holy bread eaten in the Christian service of the Communion
deign: to lower oneself to do something one considers unimportant
decorum: behaviour/appearance showing proper respect for the manners and customs of society
annihilation: complete destruction
Mbanta: the name of a place known for people who could prepare very powerful iyis
sidled: to move uncertainly or secretively as if ready to turn and go the other way
Abina pickinim de born?: Is he giving birth to a child?
indelible: marks that cannot be rubbed out; permanent
jauntily: confident and pleased with life
lout: a clumsy, crude person
complete non-entity: entirely unimportant person
christened: named
stickler: a person who absolutely insists on something
muffled: deadened or suppressed
non-entity: a person without much ability, character or importance
Ozo feast: ‘Ozo’ is the name of a title conferred on a man for meritorious deeds; Ozo feast is a grand feast during which such a title is conferred on a man

The main aim is to share the knowledge and help the students of 2nd PUC to secure the best score in their final exams. Use the concepts of Karnataka 2nd PUC English Answers Chapter 12 The Voter in Real time to enhance your skills. If you have any doubts you can post your comments in the comment section, We will clarify your doubts as soon as possible without any delay.

2nd PUC English Textbook Answers Springs Chapter 7 The Gardener

October 28, 2023

Students who are in search of The Gardener Questions and Answers Pdf, Notes, Summary. First check in which chapter you are lagging and then Download Karnataka Board 2nd PUC English Textbook Answers Chapter Wise. Students can build self confidence by solving the Answers with the help of Karnataka State Board 2nd PUC. English is the scoring subject if you improve your grammar skills. Because most of the students will lose marks by writing grammar mistakes. So, we suggest you to Download Karnataka State Board 2nd PUC English Answers according to the chapters.

Karnataka 2nd PUC English Textbook Answers Springs Chapter 7 The Gardener

Check out the topics covered in 2nd PUC before you start your preparation. Improve your grammar skills with the help of Karnataka 2nd PUC English pdf links. The Answers for Karnataka 2nd PUC English Textbook Springs Chapter 7 The Gardener are prepared by the English experts. So, if you follow Karnataka 2nd PUC English Textbook Answers you can cover all the topics in 2nd PUC Chapter 7 The Gardener. This helps to improve your communication skills.

The Gardener Questions and Answers, Notes, Summary

The Gardener Comprehension I

The Gardener Notes KSEEB Solution Question 1.
What qualities of the old man impressed the narrator?
Answer:
The old man impressed the narrator by his eyes suffused with strange memories and native intelligence. He had strong muscular arms, grey hairs and a beak-like nose. He had a newspaper tucked under his arm and a spade in his hands. The narrator felt the old man was a labourer, overseer and philosopher all rolled into one – a multi-dimensional personality.

The Gardener Summary KSEEB Solution Question 2.
Is it a significant factor that the old man came to the garden after walking hundreds of miles?
Answer:
Yes. The narrator learns from the old man that he had come to that garden after he had walked hundreds of miles. This fact becomes significant because, when he left his place, his intention was to die but once he came there the coconut grove and the place probably convinced him that he could live there comfortably. Moreover, before reaching this place he may have received the news of Basavaiah’s death and must have realized that life or death had no meaning for him. This transformation in his mindset may have prompted him to settle there.

The Gardener 2nd Puc Notes KSEEB Solution Question 3.
The owner of the garden became lethargic because
a. the income of the garden improved dramatically.
b. he had become dependent upon the gardener.
c. there was nothing much left for the owner to do.
Answer:
(c) there was nothing much left for the owner to do.

The Gardener Summary 2nd Puc KSEEB Solution Question 4.
Why did the owner’s wife start worrying about the strange ways of her husband?
Answer:
The owner of the coconut plantation was quite normal. He was working hard to bring about improvement in his earnings. Probably he had little expertise in managing agricultural workers. Therefore, he was looking for someone who would help him. That is why, the moment he spoke to the old man (Tamanna) he felt that he had got the kind of man he wanted. His expectations proved right and the old man helped him in every way and solved all his problems, which eventually resulted in increase in his income.

Once his worries disappeared and he had hardly any work to engage himself in, his personal attention went towards acquiring property and social prestige. It is quite natural that with social prestige also follow certain vices among which adultery was one. Adultery affects any woman. All along, her husband had been faithful to her and once his wife came to know that he was spending his money and time with other women, she got seriously worried. She found it hard to decide whether the arrival of the old man had done good or bad to her husband.

The Gardener Question Answer KSEEB Solution Question 5.
When did the old man decide to narrate his story?
Answer:
The old man, who had settled there in the coconut plantation for good, had watched the activities of the owner. Initially, the owner used to show interest in improving his plantation. Once the old man had solved all the problems, the owner had plenty of leisure and he became lazy. Secondly, he had more money which led him to expand his plantation. Then he had turned his gaze towards acquiring property and social prestige. Then he cultivated certain vices among which adultery was one.

One day, he saw the owner’s wife in the plantation. He saw her looking worried. He guessed that her husband’s activities had given her the worries. Therefore, when he saw her in the plantation he thought he should narrate his own story and try to caution her about her husband. He had understood the way we face ups and downs in our life. By doing so, he probably believed that she might be able to convince her husband about his mistakes and set his life right.

The Gardener Kseeb Solutions KSEEB Solution Question 6.
Tammanna considers his rival, Sangoji/Basavaiah, an important possession because
a. competition helps in the development of an individual.
b. Sangoji/Basavaiah leads a more colourful life.
c. rivalry offers new possibilities of life for him.
Answer:
(c) rivalry offers new possibilities of life for him.

2nd Puc English The Gardener Notes KSEEB Solution Question 7.
“No, his name was not Sangoji, but Basavaiah,” said the old man because
a. he had really forgotten the name.
b. he wanted to keep the identity of his rival a secret
c. he was fictionalizing his past.
Answer:
(c) he was fictionalizing his past.

Question 8.
What unique strategy did Tammanna conceive to annihilate Basavaiah?
Answer:
When Tammanna came to know that Basavaiah had forcibly acquired two hundred acres of his land, he could not tolerate this invasion. Though his supporters explained to him all the means available to him, he was not satisfied with them because he knew that sooner or later Basavaiah would again try to outdo him by hook or by crook. Tammanna did not want Basavaiah to trouble him again. So, he hit on a unique plan. He took recourse to singing ballads and telling the people through them about the cruelty and the meanness of Basavaiah. This way he thought he could annihilate him completely.

Question 9.
Why does Basavaiah start inviting scholars and musicians to his place?
OR
How did Basavaiah try to overcome his humiliation?
Answer:
Once Tammanna started making mention of Basavaiah’s cruelty and meanness in his songs, Tammanna became very popular among scholars of folklore and critics. Basavaiah watched all this in humiliation. Basavaiah tried to redress his humiliation by acquiring material wealth. He bedecked himself with gold, diamonds and other precious stones, and started living in a palatial mansion. But the visitors to his house told him that his house looked dull and empty because Tammanna’s books were not there. Basavaiah thought of investing his home with meaning by inviting scholars, poets and musicians to his place.

Question 10.
What was Basavaiah’s ray of hope in his attempts to outwit Tammanna?
Answer:
When Basavaiah failed in all his attempts to outwit Tammanna, he came to know that Tammanna had fallen ill. This news cheered up Basavaiah’s spirits. This gave him a ray of hope that by keeping himself healthy he could outwit Tammanna who was ill. Tammanna’s disease became Basavaiah’s health.

Question 11.
Tammanna decides to give up everything and leave the place because of
a. he sees no purpose in living there.
b. he wants to create an impression that he is dead.
c. he wants to put an end to the rivalry.
Answer:
(b) he wants to create an impression that he is dead.

Question 12.
Tammanna forgets his songs and ballads because
a. he finds them futile.
b. he doesn’t need them anymore.
c. he avenges himself.
Answer:
(b) he doesn’t need them anymore.

The Gardener Comprehension II

Question 1.
How did the owner’s lifestyle change after the arrival of the old man?
OR
“The old man’s stay on the farm led to many changes in the owner’s lifestyle.” Illustrate with details from the short story to support this statement.
Answer:
The owner had been working hard to improve his plantation, but there were many problems which he had not been able to solve. However, when the old man met him, he came to know that the old man was well-versed in agriculture and had native intelligence. Therefore he appointed him immediately. His expectations proved right. The old man proved his worth in solving all the problems. Consequently, the income from the garden improved dramatically. This caused a perceptible change in the lifestyle of the owner. He expanded his plantation.

However, he became lethargic and shied away from hard work. His wealth and social prestige also increased. He acquired a number of friends in the next town as well as in his own village. Even though he had precious little to do, his life became crowded with colourful events. He cultivated umpteen vices including adultery.

Question 2.
What advice did the supporters of Tammanna give for getting back his land?
Answer:
When Basavaiah acquired Tammanna’s two hundred acres forcibly, Tammanna could not tolerate this invasion. Tammanna’s supporters advised him about the various methods available for getting back his land. They told him that he could go to the court of law. He could also take recourse to the police. If he did not want to do either, there were any number of persons ready to attack Basavaiah and using force and violence to wrest his land from him.

Question 3.
How did Tammanna react to Basavaiah’s encroachment of his land?
Answer:
When Tammanna-came to know that Basavaiah had forcibly taken away two hundred acres of his land, he could not tolerate this invasion. When his supporters advised him to either go to the court or seek the help of the police or ask some persons to attack Basavaiah and take back his land forcibly, Tammanna hit on a unique idea, of annihilating Basavaiah. He thought of getting all his experiences composed in the form of ballads and telling the people through them about the cruelty and the meanness of Basavaiah.

Question 4.
How did Basavaiah try to overcome his humiliation?
Answer:
When Tammanna started singing ballads through which he told the people about Basavaiah’s cruelty and his meanness, he became very popular. Many scholars of folklore and literary critics translated his songs and earned their share of the fame. All this made Basavaiah shrink in humiliation. However, he tried to redress his humiliation by acquiring more material wealth and luxuries. He got a palatial mansion built for himself. He appointed a number of persons just to praise him. He bedecked himself with gold, diamonds, and other precious stones. He even started inviting scholars, poets and musicians to his place with the intention of investing his home with meaning.

The Gardener Comprehension III

Question 1.
The rivalry between Tammanna and Basavaiah keeps moving from the visible domain to the invisible. Comment.
Answer:
In the beginning, there appeared to be a healthy competition between Tammanna and Basavaiah. Tammanna did not take Basavaiah as his rival at all. Therefore, when Basavaiah acquired fifteen admirers to outdo Tammanna’s ten friends, it did not come to Tammanna’s notice at all. Tammanna did whatever he wanted without bothering about Basavaiah. But Basavaiah did not keep quiet. When he came to know that Tammanna possessed one thousand acres of land, and he had only eight hundred, he could not tolerate this. He sent word to Tammanna asking him to sell two hundred acres to him. Tammanna did not agree.

On the contrary, he offered to buy all the land that belonged to Basavaiah. Therefore, Basavaiah went along with his people and acquired two hundred acres of Tammanna’s land forcibly and got a fence built around it. Tammanna could not tolerate this invasion. Later, when his supporters suggested to him that he could go to the court of law or the police or use his own people to attack him and forcibly wrest his land from him, Tammanna did not accept their suggestion. Tammanna probably thought that competing with Basavaiah by physical means has no end to it because it depends on who is able to muster more muscle power. Muscle power has its own limitations. Secondly, muscle power needs the involvement of many more people apart from Tammanna.

Moreover, as long as both of them were fighting by visible means people will not know who was trying to compete with whom. Until then, Basavaiah was the first one to show to the people he had more land, more friends, more wealth, etc. Tammanna never did anything to spite Basavaiah. Whatever Tammanna did, was on his natural inclination and not to spite Basavaiah. Therefore, Tammanna realized the limitations of competing with Basavaiah by physical means. That is why he thought of putting an end to the unhealthy rivalry of Basavaiah by taking recourse to something invisible. He took recourse to singing ballads and telling the people through them about the cruelty and the meanness of Basavaiah.

Question 2.
How does Tammanna adopt a counter-strategy to challenge the material wealth of Basavaiah?
Answer:
When Tammanna came to know that the rivalry between him and Basavaiah had reached a peak and that two hundred acres of his land had been forcibly taken away from him and had been even fenced up, Tammanna hit on a plan of annihilating Basavaiah completely. He got all his bitter experiences with Basavaiah composed in the form of ballads and started singing them before the people, announcing to everyone Basavaiah’s cruelty and his meanness. This way his reputation as an artist started spreading fast and critics and scholars of folklore thronged him and translated his songs.

Question 3.
Tammanna turns reflective in the course of his life. What does this tell us about human nature?
Answer:
‘The Gardener’ is the story of Tammanna, an old man, now employed in a coconut plantation. Though the story is initially narrated by the author, Tammanna himself becomes the narrator later. Tammanna is the protagonist in the story and he tells the story of the rivalry between two farmers Tammanna and Basavaiah. Though Tammanna is one of the characters in the story, the narrator does not disclose his identity till the end. The second narrator tells us that Tammanna was a farmer, had ten acres of land, a comfortable house and people too ready to carry out his orders. Then he tells us about his rival Basavaiah.

We learn from the narrator that Tammanna did not perceive Basavaiah as his rival initially. Tammanna led a normal life and became prosperous gradually and came to possess 1000 acres of land. Until some point whatever Basavaiah did to keep himself on par with Tammanna was seen as healthy competition.

But, one day, Basavaiah asks Tammanna to sell him his two hundred acres of land and Tammanna refuses. Basavaiah takes the land forcibly. Though there were various options available for getting his land back, Tammanna searches for a method that could annihilate Basavaiah completely. Instead of proving might is right or seeking justice from the court of law, Tammanna uses a different strategy. He composes and sings ballads about Basavaiah’s meanness and cruelty. Very soon Tammanna becomes very popular and Basavaiah has no answer to his brainy ideas.

Secondly, Tammanna having found meaningful engagement in ‘art’ forgets Basavaiah’s bad deeds. Just when Basavaiah is contemplating what to do next to spite Tammanna, he comes to know that Tammanna is ill. Basavaiah is pleased with the news. But their rivalry does not end there. Tammanna decides to outbeat him by manipulating the situation itself. He gives up everything and goes away to Chennarayapatna so as to spread the news that Tammanna is dead. Later Basavaiah dies a natural death. When Tammanna comes to know about his death, he becomes reflective. Though there is no cause-effect relationship between the rumour of Tammanna’s death and Basavaiah’s real death, Tammanna is shaken out of his senses.

Until then both Basavaiah and Tammanna indulged in rivalry to satisfy their ego. With the death of Basavaiah, Tammanna loses his identity and he becomes a non-entity. This makes Tammanna reflect over human nature and comes to the conclusion that man needs some issue to fight for or cling on to. In this game, when the loser dies it is natural for the winner to feel guilty. He suffers from a sense of guilt that he was responsible for Basavaiah’s death. Naturally, when Tammanna is accused by his own conscience, he becomes reflective. When one becomes reflective, one tends to review one’s own actions objectively. We do not normally attach emotions to our thinking and then we discover what went wrong and where. Here, both Tammanna and Basavaiah have not done anything ‘bad’ intentionally. They only fought imaginary battles and lived in a dream world of their own.

Man is mortal and all the glory that man believes to enjoy is created by our mind. Man is a dreamer and lives in a dream world of his own. Since man’s life has its own limitations, man’s dream and reality rarely go in unison. When reality overtakes man, the man comes to realize that he is only a puppet in the scheme of things and man is in reality ‘nothing’, but builds up his own image to boost his confidence so that life becomes meaningful as long as he is alive.

Question 4.
How does the reference to Russia and America provide another dimension to the story?
Answer:
‘The Gardener’ presents the story of two farmers who get actively involved in rivalry and each one tries to overtake the other in earnings, wealth and social prestige. But, at one point, Basavaiah takes recourse to force and forcibly occupies a part of Tammanna’s landholdings. Until now both of them had tried to upstage the other using tangible means. But now Tammanna realizes the limitations of muscle power and so uses his ‘intellect’ to unleash a strategy by which he wishes to annihilate Basavaiah completely. He gets all his bitter experiences with Basavaiah composed in the form of ballads and songs and sings them in public.

Thus, Tammanna tries to give a fitting reply to Basavaiah’s use of physical force and so their fight takes a psychological dimension. Basavaiah fails to match up to the manipulatory tactics of Tammanna and shrinks in humiliation. However, he tries to console his wounded pride by indulging in luxurious living. No matter what he does, he does not succeed in upstaging Tammanna. It is at this stage higher forces play their own role. Tammanna falls ill and Basavaiah enjoys psychologically telling himself that Tammanna’s disease is Basavaiah’s health. This way, Basavaiah gets the satisfaction of finding a reason to keep himself happy. So, here the story has now passed from the physical dimension to the psychological dimension.

At this stage, Tammanna comes out with another plan. He plans to use something beyond man’s life. Tammanna thinks that as long as Basavaiah knows that he is alive, Basavaiah will continue to take him as his rival. So Tammanna hits on an idea which works at a level higher than the physical and psychological level. His plan is to beat Basavaiah on another plane, which is beyond man’s limits. He knows for sure that if Basavaiah comes to think that Tammanna is dead, Basavaiah will stop treating him as his rival. Tammanna goes away to Chennarayapatna and spreads the news that Tammanna is dead. It is sheer coincidence that Basavaiah dies a natural death. But Tammanna comes to feel guilty that he was the cause of his death. This incident brings him back to his senses and he reflects over his life.

Now, after the death of Basavaiah, Tammanna has become a non-entity. When he reflects over their mutual game plans, Tammanna realizes that man invents several reasons to make his life meaningful. He also realizes that man can go on indulging in whims and fancies until a certain age only. Later, when the man comes to confront reality, he comes to see the truth. Then he becomes spiritual. That is when a man realizes his limitations. Thus we see the plot taking several dimensions from physical to psychological and then to spiritual.

But, this story also takes another dimension. Earlier, stories that used to focus on man’s vices like cruelty, meanness, greed, jealousy, rivalry, etc., used to be portrayed on a smaller canvas and the stories used to be confined to people living in towns, cities and kingdoms. We are now in the post-modern society and the common man is now being influenced by global forces. In traditional societies, solutions to man’s mundane, existential problems used to be found locally. A king or a Lord or a chieftain or a zamindar used to dispense justice. But this story is situated in a post-modern society.

When Basavaiah acquires Tammanna’s land forcibly, Tammanna’s followers offer three solutions – seek the help of the police; seek a legal solution; or take recourse to use muscle power. The three strategies suggested by Tammanna’s followers saturate this story in the post-modern scenario. Their thinking exhibits a modern world where police, law court and mafia exist. Even at the national and international levels, big countries like Russia and America follow such strategies.

The fact that Tammanna makes a reference to Russia and America, is only a hint by the author that the problems of ‘man’ in the modern society cannot be confined to the war between ‘virtues and vices’ of the yesteryears but man’s problems are complex and are reflected at the global level also.

Similarly, when Tammanna makes a reference to Russia’s declaration to America that America is not their enemy and she will not wage a war against America, it is only a strategy by the writer to suggest that Tammanna and Basavaiah belong to post-modern society. One can also infer that a literary artist in the modern world cannot be blind to man’s problems in general and try to suggest solutions at the local level. Man’s problems are deep-rooted and have implications at different levels. Man’s problems go beyond a nation and though problems of human society are the same, they assume different dimensions as human society becomes more and more complex every day.

Question 5.
Observe how the story employs multiple narratives. How does this technique unveil the mystery of human relationships?
Answer:
In the short story ‘The Gardener’ by P. Lankesh, there is a story within a story and there are two narrators.
In the first story, the author in the persona of the first-person narrator introduces the protagonist Tammanna and the story of the owner of the coconut plantation. Then, the first narrator makes way for the second narrator to take over. Interestingly, in the story, the narrator is the protagonist and tells his own story to the second owner’s wife. However, the narrator employs the third-person narrative style and distances himself from the story. Thus ‘The Gardener’ is an example of the use of multiple narratives.

Multiple narratives describe a type of story that follows several protagonists rather than focusing on one main character. In some cases, writers choose this structure to show the individual perspectives of characters in a larger ‘macro story’ and how they relate to each other. ‘The Gardener’ is an example of the second type. Tammanna is the protagonist in both stories.

In the first story, we learn how Tammanna uses his own biography to indirectly give a message to the owner’s wife. He gives to the lady his own perspective of human life and experience. The author has used this technique of multiple narratives to reveal the mystery of human relationships.

In his own life, Tammanna has learnt lessons of being proactive in a rivalry with Basavaiah. He plays his game with Basavaiah, only until he dies. Basavaiah’s death comes as a revelation to him about human nature. Only then does he theorise saying ‘man does not live with the intention of acquiring wealth, or education or art but for some kind of unbearable vengefulness’. Until then, both Basavaiah and Tammanna showed a keen interest in fighting and a zest for life. But once Basavaiah dies, Tammanna loses all enthusiasm for life. He says he is a non-entity now.

Now he has become philosophical. Therefore, when he finds the owner of the coconut plantation cultivating all kinds of vices and the lady getting desperate about her husband, the ‘guilt’ about Basavaiah’s death that was pricking his conscience gets transformed into a virtue or a positive force and that motivates him to tell his own story objectively to the owner’s wife.

Had not the author used multiple narrative structures, Tammanna’s story could not have come out as a story to educate the owner’s wife. Thus, multiple narrative structures is able to unveil the mystery of human relationships.

The Gardener Additional Questions and Answers

I. Answer the following questions in a word, a phrase, or a sentence each:

Question 1.
When, according to the narrator, does man lose his name?
Answer:
After a particular age.

Question 2.
When did Tammanna forget all his songs and ballads?
OR
Whose death made Tammanna forget his songs and ballads in ‘The Gardener’?
Answer:
After the death of Basavaiah/ Death of Basavaiah.

Question 3.
Where was the coconut grove where the narrator met the old man?
Answer:
Near Chennarayapatna.

Question 4.
How big was the plantation when the old man took over?
Answer:
Ten acres.

Question 5.
What was the most important possession ofTammanna?
OR
Whom did Tammanna consider as the most important among all his possessions?
Answer:
His rival Sangoji or Basavaiah.

Question 6.
Who was Tammanna’s rival?
Answer:
Basavaiah.

Question 7.
How much of Tammanna’s land did Basavaiah acquire forcibly at first?
OR
How many acres of land did Basavaiah encroach?
Answer:
Two hundred acres.

Question 8.
When did the rivalry between Tammanna and Basavaiah move from the visible to the abstract level?
Answer:
When Tammanna composed ballads mentioning Basavaiah’s cruelty and his meanness and sang them, the rivalry between Tammanna and Basavaiah moved from the visible to the abstract level.

Question 9.
Mention one of the ways suggested by Tammanna’s followers to get back his encroached land.
Answer:
‘Going to the court of law seeking justice’ was one of the ways suggested by Tammanna’s followers to get back his encroached land.

Question 10.
What was the theme of Tammanna’s songs?
OR
Whose cruelty and meanness did Tammanna’s songs make mention of?
Answer:
The theme of Tammanna’s songs was cruelty, meanness and the injustice done to him by Basavaiah.

Question 11.
Tammanna’s disease was Basavaiah’s
(a) health
(b) weakness
(c) sorrow.
Answer:
(a) health.

Question 12.
When, according to Tammanna, did Basavaiah have no more reason to live?
Answer:
According to Tammanna, the moment he left the town and disappeared from Basavaiah’s sight, Basavaiah did not have any reason to live.

Question 13.
What became the main reason of Tammanna’s life?
Answer:
Punishing or annihilating Basavaiah for the injustice, cruelty, and meanness showed by him was the main reason of Tammanna’s life.

Question 14.
Where did the narrator meet the old man by chance?
OR
Where did the author notice the old man standing with a spade in one hand and a newspaper tucked under his arm?
Answer:
The author or the narrator noticed the man standing in a coconut grove near Chennarayapatna.

Question 15.
What had the old man tucked under his arm when the narrator met him?
Answer:
The old man had a newspaper tucked under his arm.

Question 16.
What came to an end after the old man’s arrival in the garden?
OR
When did the petty thefts come to an end?
Answer:
The petty thefts in the garden came to an end after the old man’s arrival.

Question 17.
Who became apprehensive about the plantation owner’s vices?
Answer:
The owner’s wife became apprehensive about the owner’s adultery and his umpteen other vices, cultivated lately.

Question 18.
How many acres of land did Tammanna finally have?
Answer:
Tammanna finally had only eight hundred acres of land.

Question 19.
What did Basavaiah do to counter Tammanna’s fame as a poet?
Answer:
To counter Tammanna’s fame as a poet, Basavaiah started inviting scholars, poets and musicians to his place.

Question 20.
Who became lethargic after the arrival of the old man in ‘The Gardener’?
Answer:
In The Gardener’, the owner of the plantation in Chennarayapatna became lethargic after the arrival of the old man.

Question 21.
Whose name is said to be Sangoji and later corrected as Basavaiah in ‘The Gardener’?
Answer:
In The Gardener’, it is Tammanna’s rival whose name is said to be Sangoji and later corrected as Basavaiah.

Question 22.
Who hit upon the idea of composing and singing ballads in ‘The Gardener’?
Answer:
In The Gardener’, Tammanna hit upon the idea of composing and singing ballads.

Question 23.
Who was felicitated as the best poet of his times in ‘The Gardener’?
Answer:
In The Gardener’, it was Tammanna who was felicitated as the best poet of his times.

Question 24.
Who appointed a number of persons to praise him in ‘The Gardener’?
Answer:
In The Gardener’, it was Basavaiah who appointed a number of persons to praise him.

Question 25.
Whose palatial mansion looked dull and empty without Tammanna’s books in ‘The Gardener’?
Answer:
In The Gardener’, it was Basavaiah’s palatial mansion which looked dull and empty without Tammanna’s books. ‘

Question 26.
When did Basavaiah find the means of surpassing Tammanna in ‘The Gardener’?
Answer:
In The Gardener’, it was only when Tammanna fell ill, did Basavaiah find a means of surpassing Tammanna.

Question 27.
Whose disease was Basavaiah’s health, according to the narrator of ‘The Gardener’?
Answer:
According to the narrator of The Gardener’, Tammanna’s disease was Basavaiah’s health.

Question 28.
When would Basavaiah go on offering stiff competition, according to Tammanna, in ‘The Gardener’?
Answer:
According to Tammanna, in The Gardener’, Basavaiah would go on offering stiff competition at the level of the body, until he died.

Question 29.
According to the old man, when did he conceive the story of Tammanna and Basavaiah?
Answer:
According to the old man, he conceived the story of Tammanna and Basavaiah, one day, when he found the wife of the plantation owner in a fix, perturbed and confused.

Question 30.
Who thought his death alone could destroy Basavaiah, in ‘The Gardener’?
Answer:
In The Gardener’, Tammanna thought that his death alone could destroy Basavaiah.

Question 31.
When did Basavaiah pass away, according to Tammanna, in ‘The Gardener’?
Answer:
According to Tammanna in The Gardener’, Basavaiah passed away after Tammanna had left his village.

Question 32.
Who says he avenged himself by becoming a non-entity in ‘The Gardener’?
Answer:
In The Gardener’, Tammanna says that he avenged himself by becoming a non-entity.

Question 33.
Who, according to Tammanna the old man, is not amenable to any advice in ‘The Gardener’?
Answer:
In ‘The Gardener’, according to Tammanna the old man, the owner of the coconut grove (where he was a labourer), was not amenable to any advice.

Question 34.
How long does a man go on living for some revenge, according to the old man, in ‘The Gardener’?
Answer:
According to the old man in ‘The Gardener’, the man goes on living for some revenge or the other till the day of his death.

Question 35.
Who paints well, according to the old man, in ‘The Gardener’?
Answer:
According to the old man in ‘The Gardener’, the young child of Lokya paints well.

Question 36.
What happened to Basavaiah after Tammanna gave up everything and went away?
Answer:
Basavaiah died a few days after Tammanna had left the town giving up everything.

Question 37.
Who narrated his story to the wife of the plantation owner in Gardener’?
Answer:
In ‘The Gardener’, Tammanna narrated his story to the wife of the p. .tation owner.

Question 38.
What was the old man well versed in ‘The Gardener’?
Answer:
Agriculture.

Question 39.
When did Tammanna forget his songs and ballads?
Answer:
Tammanna forgot all his songs and ballads after Basavaiah’s death.

II. Answer the following questions in a paragraph of 80-100 words each:

Question 1.
Why does Tammanna feel that human nature can be strange?
OR
‘Man goes on living for some revenge’. How is this presented in ‘The Gardener’?
Answer:
One afternoon, Tammanna finds the owner’s wife coming towards the coconut grove. She looked worried and anxious. However, Tammanna knew why she looked so. Then, he narrates the story of Tammanna and Basavaiah and finally confesses that he was Tammanna, Basavaiah’s rival, and how he had given up all his property and come to Chennarayapatna. Before telling her that Basavaiah had died, Tammanna tells her he had come to realize that human nature is very strange. He offers an explanation of why he thinks so.

According to Tammanna, though man needs wealth, education, and many more things, they do not give him a compelling reason to live. In his opinion ‘Man lives for some kind of unbearable vengefulness’. He arrives at this inference based on his own experience of life. As long as he was staying in his village, Basavaiah had considered him his rival and had gone on trying to out beat him in wealth, health, art, and so on. The very fact that there was a rival to him and he had to strive to compete with him in every aspect, gave him sufficient reason to live. It is here that one finds human nature strange.

All through his life, though man struggles to earn wealth, education, food, etc., he does not find real happiness in these things. But he derives a kind of pleasure when he finds that there is someone competing with him in these areas. Though it is the making of his own imagination yet he finds pleasure accepting his imaginary rival as real and fighting to out beat him. This gives him the real reason for his existence.

Having come away from Basavaiah, to punish him with the news of his death, Tammanna realizes that human nature is very strange. After the death of Basavaiah, he realises that he had become a non-entity and had lost his name and fame. He tells his own story along with the truth that he had realized, only to convince the owner’s wife that she needs to mend her husband.

Question 2.
What measures did Tammanna adopt to humiliate Basavaiah? Explain.
OR
Give an account of the strategies used by Tammanna to destroy Basavaiah in ‘The Gardener’.
Answer:
When Tammanna came to know that the rivalry between him and Basavaiah had reached a peak and that two hundred acres of his land had been forcibly taken away from him and had been even fenced up, Tammanna hit on a plan of annihilating Basavaiah completely. He got all his bitter experiences with Basavaiah composed in the form of ballads and started singing them before the people, announcing to everyone Basavaiah’s cruelty and his meanness. This way his reputation as an artist started spreading fast and critics and scholars of folklore thronged him and translated his songs.

As days rolled by, Tammanna’s popularity increased, and Basavaiah began to shrink in humiliation. Basavaiah tried to undo the damage to his self-esteem by showing more interest in acquiring all kinds of material wealth. He got a palatial mansion built for himself. Then he bedecked himself with gold, diamonds, and other precious stones. Then he started inviting scholars, poets, and musicians to his place and tried to invest his home with meaning. However, one-day Tammanna suddenly took ill. This news cheered up Basavaiah’s spirits. Tammanna’s disease became Basavaiah’s health. Tammanna thought of yet another method of punishing Basavaiah.

Tammanna thought Basavaiah could no longer compete with him if he came to know that Tammanna had died. Therefore, Tammanna avenged himself by leaving his town, abandoning all his property, and walking away hundreds of miles. When Basavaiah came to know that Tammanna was not there in the village, he had no more reason to live and he passed away.

Question 2.
Why did the plantation owner’s wife find it hard to decide whether the old man’s arrival was for the better or for the worse?
Answer:
Before the arrival of the old man, the owner had only ten acres of land. Though the owner himself was in charge of the work in the plantation, there used to be petty thefts and he could not prevent them. Secondly, he was very busy and hard-working and hardly had any time to spend with his friends. So, he had hardly any friends at all.

Once the old man was appointed as an overseer on the farm, the old man being well-versed in agriculture, understood the problems of the workers and solved all the problems. His efficient supervision resulted in a dramatic increase in the earnings of the farm. Consequently, the owner expanded his farm, became lethargic, and shied away from hard work, leaving the plantation in charge of the old man.

Furthermore, the owner’s wealth and social prestige also increased. Along with that, he acquired a number of friends in the next town as well as in his own village. Even though he had precious little to do, his life became crowded with colourful events. He also cultivated umpteen vices including adultery. These changes in her husband’s lifestyle made the wife wonder whether the old man’s arrival was for the better or for the worse.

Question 3.
How did the rivalry between Tammanna and Basavaiah move towards an invisible, abstract domain?
OR
Trace the course of the rivalry between Tammanna and Basavaiah that moved from a visible domain to an abstract domain.
OR
Give an account of the strategies used by Tammanna to destroy Basavaiah.
OR
Bring out the rivalry between Tammanna and Basavaiah.
OR
The rivalry between Tammanna and Basavaiah started moving from the visible to the invisible domain. Explain.
OR
Explain the methods adopted by Tammanna to humiliate Basavaiah.
Answer:
In the beginning, there appeared to be a healthy competition between Tammanna and Basavaiah. Tammanna did not take Basavaiah as his rival at all. Therefore, when Basavaiah acquired fifteen admirers to outdo Tammanna’s ten friends, it did not come to Tammanna’s notice at all. Tammanna did whatever he wanted without bothering about Basavaiah. But Basavaiah did not keep quiet.

When he came to know that Tammanna possessed one thousand acres of land, and he had only eight hundred, he could not tolerate this. He sent word to Tammanna asking him to sell two hundred acres to him. Tammanna did not agree. On the contrary, he offered to buy all the land that belonged to Basavaiah. Therefore, Basavaiah went along with his people and acquired two hundred acres of Tammanna’s land forcibly and got a fence built around it. Tammanna could not tolerate this invasion. Later, when his supporters suggested to him that he could go to the court of law or the police or use his own people to attack him and forcibly wrest his land from him, Tammanna did not accept their suggestion.

Tammanna probably thought that competing with Basavaiah by physical means has no end to it because it depends on who is able to muster more muscle power. Muscle power has its own limitations. Secondly, muscle power needs the involvement of many more people apart from Tammanna.

Therefore, Tammanna realized the limitations of competing with Basavaiah by physical means. That is why he thought of putting an end to the unhealthy rivalry of Basavaiah by taking recourse to something invisible. He took recourse to singing ballads and telling the people through them about the cruelty and the meanness of Basavaiah.

Question 4.
How did Basavaiah try to surpass Tammanna? Why wasn’t he successful?
OR
How did Basavaiah try to surpass his rival in ‘The Gardener’?
OR
How did Basavaiah react to Tammanna’s popularity?
OR
How did Basavaiah start filling his life with all kinds of material wealth?
Answer:
When Tammanna came to know that the rivalry between him and Basavaiah had reached a peak and that two hundred acres of his land had been forcibly taken away from him and had been even fenced up, Tammanna hit on a plan of annihilating Basavaiah completely. He got all his bitter experiences with Basavaiah composed in the form of ballads and started singing them before the people, announcing to everyone Basavaiah’s cruelty and his meanness. This way his reputation as an artist started spreading fast and critics and scholars of folklore thronged him and translated his songs.

Basavaiah tried to surpass Tammanna’s fame by filling his life with all kinds of material wealth. He got a palatial mansion built for himself. He appointed a number of persons just to praise him and bedecked himself with gold, diamonds, and other precious stones. But he was not successful. We can conclude so because the visitors to his house told him that without Basavaiah’s books his house looked dull and empty.

Question 5.
How does Tammanna take revenge on Basavaiah through invisible means?
OR
Explain the invisible means by which Tammanna decided to destroy Basavaiah.
OR
What invisible means did Tammanna use to annihilate Basavaiah completely? Explain.
Answer:
When his supporters advised him to either go to the court or seek the help of the police or ask some persons to attack Basavaiah and take back his land forcibly, Tammanna hit upon a unique idea of annihilating Basavaiah through invisible means. He thought of getting all his experiences composed in the form of ballads and singing them before the public.

When Tammanna started singing ballads through which he told the people about Basavaiah’s cruelty and his meanness, he became very popular. Many scholars of folklore and literary critics translated his songs and earned their share of fame. All this made Basavaiah shrink in shame. This way, Tammanna took revenge on Basavaiah through invisible means.

Question 6.
How did Tammanna and Basavaiah manage their rivalry in the beginning in ‘The Gardener’?
Answer:
In the beginning, there appeared to be a healthy competition between Tammanna and Basavaiah. Tammanna did not take Basavaiah as his rival at all. Therefore, when Basavaiah acquired fifteen admirers to outdo Tammanna’s penfriends, it did not come to Tammanna’s notice at all. Tammanna did whatever he wanted without bothering about Basavaiah. But Basavaiah did not keep quiet. When he came to know that Tammanna possessed one thousand acres of land, and he had only eight hundred, he could not tolerate this. He sent word to Tammanna asking him to sell two hundred acres to him. Tammanna did not agree.

On the contrary, he offered to buy all the land that belonged to Basavaiah. Therefore, Basavaiah went along with his people and acquired two hundred acres of Tammanna’s land forcibly and got a fence built around it Tammanna could not tolerate this invasion. Later, when his supporters suggested to him that he could go to the court of law or the police or use his own people to attack him and forcibly wrest his land from him, Tammanna did not accept their suggestion. Tammanna realized the limitations of competing with Basavaiah by physical means. That is why he thought of putting an end to the unhealthy rivalry of Basavaiah by taking recourse to something invisible.

Question 7.
What did Basavaiah do to invest his home with meaning in ‘The Gardener’? Explain.
Answer:
As Tammanna’s popularity increased, Basavaiah began to shrink in humiliation. Basavaiah tried to undo the damage to his self-esteem by showing more interest in acquiring all kinds of material wealth. He got a palatial mansion built for himself. He appointed a number of persons just to praise him. Then he bedecked himself with gold, diamonds, and other precious stones. Then he started inviting scholars, poets, and musicians to his place and tried to invest his home with meaning.

Question 8.
Describe the circumstances that led Tammanna to become a non-entity in ‘The Gardener’.
Answer:
Tammanna is the protagonist in the story and he tells the story of the rivalry between himself and Basavaiah. Tammanna was a farmer, had ten acres of land, a comfortable house, and people too ready to carry out his orders. Besides, he also had a rival. It was Basavaiah. Tammanna did not perceive Basavaiah as his rival initially. Tammanna led a normal life and became prosperous gradually and came to possess 1000 acres of land. Until some point whatever Basavaiah did to keep himself on par with Tammanna was seen as healthy competition.

But, one day, Basavaiah asks Tammanna to sell him two hundred acres of his land and Tammanna refuses. Basavaiah takes the land forcibly. Though there were various options available for getting his land back, Tammanna searches for a method that could annihilate Basavaiah completely. Instead of proving might is right or seeking justice from the court of law, Tammanna uses a different strategy. He composes and sings ballads about Basavaiah’s meanness and cruelty. Very soon Tammanna becomes very popular and Basavaiah has no answer to his brainy ideas.

Just when Basavaiah is contemplating what to do next to spite Tammanna, he comes to know that Tammanna is ill. Basavaiah is pleased with the news. But their rivalry does not end there. Tammanna decides to out beat him by manipulating the situation itself. He gives up everything and goes away to Chennarayapatna so as to spread the news that Tammanna is dead. Later Basavaiah dies a natural death. Though there is no cause-effect relationship between the rumour of Tammanna’s death and Basavaiah’s real death, Tammanna is shaken out of his senses. With the death of Basavaiah, Tammanna loses his identity and he becomes a non-entity.

Question 9.
What circumstances led to the unhappiness of the owner’s wife in ‘The Gardener’?
Answer:
The owner of the coconut plantation was quite a normal person. He was working hard to bring about improvement in his earnings. Probably he had little expertise in managing agricultural workers. Therefore, he was looking for someone who would help him. That is why the moment he spoke to the old man [Tammanna) he felt that he had got the kind of man he wanted. His expectations proved right and the old man helped him in every way and solved all his problems, which eventually resulted in increasing his income.

All along, her husband had been faithful to her and once his wife came to know that he was spending his money and time with other women, she got seriously worried and was very unhappy. She found it hard to decide whether the arrival of the old man had done good or bad for her husband.

III. Answer the following questions in about 200 words each:

Question 1.
How did Tammanna and Basavaiah try to outdo each other?
OR
Describe the rivalry between Tammanna and Basavaiah.
OR
How did Tammanna avenge himself?
OR
How does Tammanna successfully outsmart his rival Basavaiah?
Answer:
In the beginning, there appeared to be a healthy competition between Tammanna and Basavaiah. Tammanna did not take Basavaiah as his rival at all. Therefore, when Basavaiah acquired fifteen admirers to outdo Tammanna’s ten friends, it did not come to Tammanna’s notice at all. Tammanna did whatever he wanted without bothering about Basavaiah. But Basavaiah did not keep quiet. When he came to know that Tammanna possessed one thousand acres of land, and he had only eight hundred, he could not tolerate this. He sent word to Tammanna asking him to sell his two hundred acres to him. Tammanna did not agree. On the contrary, he offered to buy all the land that belonged to Basavaiah.

Therefore, Basavaiah went along with his people and acquired two hundred acres of Tammanna’s land forcibly and got a fence built around it. Tammanna could not tolerate this invasion. Later, when his supporters suggested to him that he could go to the court of law or the police or use his own people to attack him and forcibly wrest his land from him, Tammanna did not accept their suggestion.

However, one-day Tammanna suddenly took ill. This news cheered up Basavaiah’s spirits. Tammanna’s disease became Basavaiah’s health. Tammanna thought of yet another method of punishing Basavaiah. Tammanna thought Basavaiah could no longer compete with him if he came to know that Tammanna had died. Therefore, Tammanna avenged himself by leaving his town, abandoning all his property and walking away hundreds of miles. When Basavaiah came to know that Tammanna was not there in the village, he had no more reason to live and he passed away.

Question 2.
‘Without vengefulness, there would be no reason for man’s existence’. How does ‘The Gardener’ bring this out?
Answer:
Tammanna did not take Basavaiah as a rival at all. But, Basavaiah took him as his rival seriously and tried to outdo Tammanna in everything. Therefore, when Tammanna bought four more acres adjacent to his land, Basavaiah also did the same. If Tammanna had ten friends, Basavaiah would acquire fifteen admirers. Gradually, it rose to such a pitch that there was no land left in the village for them to buy. All land belonged to either Tammanna or Basavaiah. Tammanna owned one thousand acres and Basavaiah eight hundred. Basavaiah could not tolerate this. He sent word to Tammanna asking him to sell two hundred acres.

Tammanna did not agree. He was prepared to buy all the land that belonged to Basavaiah. Basavaiah went mad with rage. He went along with his people and acquired two hundred acres of Tammanna’s land forcibly. A fence was built around that land. Tammanna could not tolerate this invasion. Though his supporters explained to him all the means available to him, he was not satisfied with them because he knew that sooner or later Basavaiah would again try to outdo him by hook or by crook. Tammanna did not want Basavaiah to trouble him again.

Question 3.
The arrival of the old man to the garden caused both good and bad things. How is this brought out in ‘The Gardener’?
Answer:
The owner of the coconut plantation was quite a normal person. He was working hard to bring about improvement in his earnings. Probably, as he did not have much experience and expertise in managing agricultural work, he was not able to reap the benefits of his hard work. Often, there used to be thefts and worker-related problems. He also felt that he needed the assistance of a person well- versed in dealing with such problems. Once he had spoken to the old man for a few minutes, he was convinced that he had found the right person and so hired him immediately. Thus the old man became an employee in the coconut grove and stayed on.

The old man was so experienced in agriculture that he easily understood the problems of workers. The petty thefts in the garden came to an end, and naturally, the income from the garden increased dramatically. Consequently, the increase in income brought a perceptible change in the lifestyle of the owner. The plantation expanded, but the owner became lazy and shied away from hard work. Once his worries disappeared and he had hardly any work to engage himself in, his personal attention went towards acquiring property, and fame. His life became crowded with colourful events.

On account of his newly acquired clout, he cultivated umpteen other vices including adultery. He became a source of worry to his wife. The owner’s wife found it hard to decide whether the old man’s arrival was for the better or for the worse. On the whole, one can conclude that the arrival of the old man to the garden caused both good and bad.

Question 4.
Rivalry can make one both aggressive and reflective. How does Tammanna’s narrative in The Gardener” prove this?
Answer:
Yes. “Rivalry can make one both aggressive and reflective”.
In ‘The Gardener*, Tammanna is both the protagonist as well as the narrator. He narrates the story of the rivalry between two farmers Tammanna and Basavaiah. From his narration, one can easily infer how aggressively he has fought with Basavaiah for saving his self-esteem.

In the beginning, he did not even imagine that he had a rival. But he becomes aware of the rivalry between himself and Basavaiah when the latter takes his land forcibly and he is made to feel helpless. He becomes alert and starts planning strategies to out beat Basavaiah’s moves. He composes and sings ballads and publicizes Basavaiah’s meanness and cruelty. Very soon Tammanna gains popularity and Basavaiah is made to feel that he has been defeated in his own game. Soon after that, when Tammanna falls ill, Basavaiah is pleased with the news. But their rivalry does not end there. Tammanna’s next move shows how aggressive and vengeful he can be. He gives up everything and goes away to Chennarayapatna so as to spread the news that Tammanna is dead.

Later, Basavaiah dies a natural death. Though there is no cause-effect relationship between the rumour of Tammanna’s death and Basavaiah’s real death, Tammanna is shaken out of his senses. He becomes reflective. He understands that with the death of Basavaiah, he had lost his identity and had become a non-entity. Finally, he realizes that both he and Basavaiah had indulged in rivalry only to satisfy their ego.

The Gardener Vocabulary

An antonym is a word opposite in meaning to a given word.

Note the use of antonyms for the following words found in the lesson:

Impoverish – enrich
Elaborate – concise
Petty – grand
Suffused – removed
Lethargic – active
Annihilate – preserve
Vengeful – benevolent
Agony – ecstasy
Flourish – languish
Wealth – poverty
Famous – obscure
Cruelty – kindness
Best – worst
Enemy – friend
Stiff – flexible

Question 1.
What do the following expressions from the lesson mean?

in a flash
flesh and blood
out of hand
vanish into thin air

Answer:

very suddenly or quickly.
the human body or nature.
out of control.
go away suddenly, unexpectedly and without a trace.

Additional Exercises

A. Passive Voice:

Question 1.
Tammanna came to the plantation after walking hundreds of miles. He _____ (appoint) to look after the garden. The workers were happy as their problems _____ (solve). The income improved dramatically but a change in the owner’s lifestyle _____ (notice) by his wife.
Answer:
was appointed; had been solved; was noticed.

Question 2.
Basavaiah had 200 acres less land than Tammanna. So Tammanna _____ (ask) to sell his 200 acres to Basavaiah. This _____ (reject) by Tammanna. Instead, a demand _____ (place) that Tammanna was ready to buy all his land.
Answer:
was asked; was rejected; was placed.

Question 3.
Basavaiah tried to outshine Tammanna in his own way. A palatial house _____ (build) by him. A number of persons ____ (appoint) just to praise him. Scholars, poets and musicians _____ (invite) to his place.
Answer:
was built; were appointed; were invited.

B. Fill in the blanks by choosing the appropriate expressions given in brackets:

Question 1.
The quarrel between Tammanna and Basavaiah rose to such a pitch that it started to ______ all their supporters. When Basavaiah forcibly acquired Tammanna’s land, Tammanna was advised by his supporters to ______ to the police. (take recourse, push in, suck in)
Answer:
suck in; take recourse.

Question 2.
Tammanna ______ everything and went to a far off place. Basavaiah was left with no reason to live. After some time Basavaiah ______. (gave up, chance upon, passed away)
Answer:
gave up; passed away.

Question 3.
‘Tammanna was ______ in agriculture. As a result, the owner of the garden started to _____ from hard work. (shy away, well versed, better at)
Answer:
well versed; shy away.

Question 4.
The narrator says he conceived the story The Gardener’ ______. The old man he met in a coconut grove had come to the garden seeking work. The owner needed a man exactly like him and asked the old man to ______. (in a flash, stay on, vanish into thin air)
Answer:
in a flash; stay on.

Question 5.
Tammanna thought of yet another method of punishing Basavaiah. He wanted to separate his songs from his own _____. He wanted his fame to ______. Therefore he gave up everything and became a non-entity. (flesh and blood, vanish into thin air, out of hand)
Answer:
flesh and blood; vanish into thin air.

C. Fill in the blanks with appropriate linkers:

Question 1.
The gardener started narrating the story of Tammanna and Basavaiah to the owner’s wife. ______ proceeding with the story, he started fumbling for words ______ he had made a mistake. The owner’s wife was not interested in the story. She felt like going away ______ stayed back ______ she did not want to hurt the old man. (but, after, as though, as)
Answer:
After; as though; but; as.

Question 2.
The rivalry between Tammanna and Basavaiah looked like healthy competition in the beginning. _____ it rose to such a pitch _____ they started competing in buying each acre of land in the village. _____ no land in the village was left for buying. Even then Basavaiah was not happy ______ he had 200 acres less than Tammanna. (because, finally, gradually, that)
Answer:
Gradually; that; Finally; because.

Question 3.
Basavaiah sent word that he was prepared to buy Tammanna’s 200 acres of land. This made Tammanna furious. _____ he said he was prepared to buy all the land ______ belonged to Basavaiah. _______ Basavaiah forcibly acquired 200 acres of Tammanna’s land. ________ the enmity between them became fierce and sucked in all their supporters. (as a result, in turn, which, instead)
Answer:
In turn; which; Instead; As a result.

The Gardener by P. Lankesh About the Writer:

P. Lankesh [1935-2000) is an Indian writer and journalist who wrote in Kannada. After graduating with an honours degree in English from Central College at Bangalore, he completed his Master of Arts degree in English from Maharaja College, Mysore. P. Lankesh’s first work was ‘Kereya Neeranu Kerege Chelli’, a collection of short stories published in 1963. This was followed by several collections of short stories and poetry, three novels, critical essays, translations (including Charles Baudelaire’s ‘Les Fleurs du Mai’, ‘Oedipus Rex’, and Sophocles’ ‘Antigone’), as well as several plays and films. His 1976 film ’Pallavi’ – a cinematic narration, told from the female protagonist’s point of view and based on his novel ‘Biruku’ – won India’s national award for best direction.

The Gardener Summary in English

[This short story is the translated English version of ’Thotadavanu’, taken from ‘When Stone Melts and Other Stories’, a collection of short stories published by Sahitya Academi. It is translated into English by H.S. Raghavendra Rao.]

It is said that in these short stories Lankesh shows his preoccupation with human meanness and attempts to explore the evolution of a post-Emergency political and cultural scenario. The title ‘When Stone Melts’ refers to the mystery at the heart of every transformation, the invisible and inescapable play of history and location that engender the process of change.

The narrator addresses the reader directly in the first person asking for an apology for being brief. He says that the story was conceived in a flash and hence if he elaborates it, the story will lose its vitality. There are only four characters, besides the narrator: Tammanna, Basavaiah or Sangoji, and the owner of the coconut grove and his wife.

There are two stories in this story. The first story is narrated by the author in the first person and the second story is narrated by Tammanna who is also the protagonist in the first story. In the second story, the narrator/protagonist tells his own story to the lady distancing himself from the main story.

The narrator says that this story originated in his chance encounter with an old man who was standing in a coconut grove near Chennarayapatna. The old man (who had been employed in the coconut grove), was a labourer, overseer and philosopher, all rolled into one.

2nd PUC English Textbook Answers Springs Chapter 7 The Gardener image - 1

One day the old man came to the coconut garden after walking hundreds of miles. Since the owner of that plantation needed a person of his qualifications, he hired him immediately after talking to him for a few minutes. Thus the old man became an employee in the coconut grove and stayed on. The old man did useful work. He was so well-versed in agriculture that he easily understood the problems of workers. The petty thefts in the garden came to an end, and naturally, the income from the garden improved dramatically. Consequently, the increase in income brought a perceptible change in the lifestyle of the owner. The plantation expanded, but the owner became lazy and shied away from hard work.

The owner’s wife found the owner’s behaviour strange and puzzling. She found it hard to decide whether the old man’s arrival was for the better or for the worse. Her husband’s wealth and social prestige had risen higher, and he had acquired a great number of friends in his own village and in the next town as well. Even though he did not do any useful work, his life became crowded with colourful events. On account of his newly acquired clout, he cultivated umpteen other vices including adultery. Though their farm was initially merely ten acres, it had grown beyond their imagination.

Therefore, the owner’s wife realized that financially they had been doing well but her only source of worry was that along with financial improvement, their life was also gradually getting out of hand. Thus, one day when she was in a fix like this, the old man met her. He smiled at her, brought down an offering offender coconuts from a nearby tree, and sat on the embankment of the well. She had no alternative and so she sat next to him. The old man now begins his narrative and takes the action or plot to its climax.

The old man says that, in a far off place, once there lived a man called Tammanna. He had everything: ten acres of land, a comfortable house, and people too ready to carry out his orders or instructions. Besides these possessions, he also had a rival and his name was Sangoji. However, soon after uttering the name Sangoji, the old man started fumbling for words as if he had committed a mistake. The coconut grove owner’s wife, who was listening, felt that it was none of her concern and felt like going away immediately. But, not wishing to hurt the old man, she continued to sit there quietly.

The old man continued his story. He corrected himself once, saying his name was not Sangoji but Basavaiah. [At this moment in the story, the narrator gives a hint to the reader that the old man is telling a true account of his own experience disguising it in the form of a story].

Both Tammanna and Basavaiah were rivals. If Tammanna bought four more acres adjacent to his land, Basavaiah would also do the same. If one of them had ten friends, the other would acquire ‘ fifteen admirers. Though initially, all this looked like healthy competition, it took a nasty turn later.

Their rivalry rose to such a pitch that there was no land left in the village for them to buy. All land belonged to either Tammanna or Basavaiah. Tammanna had one thousand acres and Basavaiah eight hundred. Basavaiah could not tolerate this. His men asked Tammanna to sell two hundred acres but Tammanna refused to do so. On the other hand, Tammanna offered to buy all the land that belonged to Basavaiah. Basavaiah became furious. He went along with his people and acquired two hundred acres of Tammanna’s land forcibly, and got it fenced up all around. Tammanna could not put up with this invasion.

Tammanna’s advisers told him that there were three ways by which Tammanna could get back his land. He could go to the court of law or he could also take recourse to the police. If he did not like to do, either way, he could also use muscle power to get his land back. There was any number of persons ready to attack Basavaiah and wrest his land from him. But Tammanna was in search of a method that could destroy Basavaiah completely. Tammanna got all his experiences composed in the form of ballads and sang them in public. Their rivalry moved away from the visible to the invisible.

Basavaiah could not do the same way. He tried to show his rivalry in doing agricultural tasks more diligently, but that was also in vain. Meanwhile, Tammanna’s reputation started spreading all around. His songs started making mention of Basavaiah’s cruelty and his meanness. Scholars and critics went after his songs and earned their share of fame. Basavaiah became desperate and angry and retaliated by encroaching on more and more of Tammanna’s land. But Tammanna was ignorant of all this and blissfully enjoyed his singing. Art had become the raison d’etre of his life. He was even felicitated as the best poet of his times.

Basavaiah felt humiliated, which he tried to hide by acquiring all kinds of luxuries. He got a palatial mansion built for himself; appointed a number of people to praise him and bedecked himself with gold, diamonds, and other precious stones. But his house looked dull and empty because Tammanna’s books were not there. He attempted to fill the lacuna by inviting scholars, poets, and musicians to his place. This way, he tried to invest his home with meaning.

One day, Basavaiah came to know that Tammanna was ill. The news made him happy. At that point, Basavaiah found the means of surpassing Tammanna. Health is wealth. Tammanna’s disease was Basavaiah’s health. But Tammanna thought differently. He had thought of yet another method of punishing Basavaiah. Tammanna contemplated ‘death’. As long as he continued his rivalry at the level of the body, Basavaiah would go on offering stiff competition. But, if he died, Basavaiah could do nothing to defeat him. The old man ends his storytelling the coconut garden owner’s wife that wishing to destroy Basavaiah completely, Tammanna gave up everything and ran off from his village.

As long as Tammanna was there, Basavaiah had a reason to be alive, but once Tammanna left the place Basavaiah passed away. The old man tells the lady that Basavaiah died because he had no reason to live. Then he confesses to her that he is Tammanna himself. After Basavaiah’s death, Tammanna tells the lady that he forgot all his songs and ballads, lost his fame, and became a non-entity. He concludes telling her that, that way he avenged himself.

Tammanna tells the woman that the experiences of his life had made him realize that human nature is very strange. He sums up his experiences in one sentence. He tells her that though man works to fulfill his many needs like wealth, education, art, and many more things, yet those things do not give him the right, compelling reasons to live. All through his life man lives for some kind of unbearable vengefulness. It is in this vengefulness that he finds a reason for his existence.

Finally, using his autobiographical account as an example, the old man. tries to covertly give her a message. He tells her that her husband was flourishing as a rich man and was not amenable to any advice. Man is so complicated that till the day of his death, he goes on living for some revenge or the other, confronting one challenge or the other. He wants her to understand that she had better try to understand why her husband is living like that.

Finally, he asks her to take the whole story as a dream and hot to take his words seriously. We can infer here that he is saddened by the coconut grove owner’s lifestyle and wants to put an end to it by cautioning the lady about her husband and do something to find out why her husband was doing so. As soon as the old man finishes his story, the first narrator reappears and tells the reader that he had seen all this in a dream and hence he is unable to elaborate.

The Gardener Summary in Kannada

2nd PUC English Textbook Answers Springs Chapter 7 The Gardener image - 2

Glossary:

Perceptible: visible, noticeable
Lethargic: lazy, sluggish
Diligent: hard-working
Raison d’etre (n) (French): reason, the reason for existence
Annihilate: destroy

The main aim is to share the knowledge and help the students of 2nd PUC to secure the best score in their final exams. Use the concepts of Karnataka 2nd PUC English Answers Chapter 7 The Gardener in Real time to enhance your skills. If you have any doubts you can post your comments in the comment section, We will clarify your doubts as soon as possible without any delay.

Category: 2nd PUC

Karnataka 2nd PUC Sociology Question Bank Chapter 3 Inclusive Strategies

2nd PUC Sociology Inclusive Strategies One Mark Questions and Answers

2nd PUC Sociology Inclusive Strategies Two Marks Questions and Answers

2nd PUC Sociology Inclusive Strategies Five Marks Questions and Answers

2nd PUC Sociology Inclusive Strategies Ten Marks Questions and Answers

Karnataka 2nd PUC Sociology Question Bank Chapter 2 Social Inequality, Exclusion and Inclusion

2nd PUC Sociology Social Inequality, Exclusion and Inclusion One Mark Questions and Answers

2nd PUC Sociology Social Inequality, Exclusion and Inclusion Two Marks Questions and Answers

2nd PUC Sociology Social Inequality, Exclusion and Inclusion Five Marks Questions and Answers

2nd PUC Sociology Social Inequality, Exclusion and Inclusion Ten Marks Questions and Answers

Karnataka 2nd PUC Sociology Question Bank Chapter 1 Making of Indian Society and Demography

2nd PUC Sociology Making of Indian Society and Demography One Mark Questions and Answers

2nd PUC Sociology Making of Indian Society and Demography Two Marks Questions and Answers

2nd PUC Sociology Making of Indian Society and Demography Five Marks Questions and Answers

2nd PUC Sociology Making of Indian Society and Demography Ten Marks Questions and Answers

Karnataka 2nd PUC English Textbook Answers Springs Chapter 10 Heaven, If you are not here on Earth

Heaven, If you are not here on Earth Questions and Answers, Notes, Summary

Heaven, If you are not here on Earth Additional Questions and Answers

Heaven, If you are not here on Earth Summary in English

Heaven, If you are not here on Earth Summary in Kannada

Karnataka 2nd PUC English Textbook Answers Springs Chapter 13 Where there is a Wheel

Where there is a Wheel Questions and Answers, Notes, Summary

Where there is a Wheel Additional Questions and Answers

Where there is a Wheel Summary in English

Where there is a Wheel Summary in Kannada

Karnataka 2nd PUC English Textbook Answers Springs Chapter 11 Japan and Brazil through a Traveller’s Eye

Japan and Brazil through a Traveller’s Eye Questions and Answers, Notes, Summary

Japan and Brazil through a Traveller’s Eye Additional Questions and Answers

Japan and Brazil through a Traveller’s Eye Summary in English

Japan and Brazil through a Traveller’s Eye Summary in Kannada

Karnataka 2nd PUC English Model Question Papers with Answers 2019-2020

Karnataka 2nd PUC English Textbook Answers Springs Chapter 6 When You Are Old

When You Are Old Questions and Answers, Notes, Summary

When You Are Old Additional Questions and Answers

When You Are Old Summary in English

When You Are Old Summary in Kannada

Karnataka 2nd PUC English Textbook Answers Springs Chapter 12 The Voter

The Voter Questions and Answers, Notes, Summary

The Voter Additional Questions and Answers

The Voter Summary in English

The Voter Summary in Kannada

Karnataka 2nd PUC English Textbook Answers Springs Chapter 7 The Gardener

The Gardener Questions and Answers, Notes, Summary

The Gardener Additional Questions and Answers

The Gardener Summary in English

The Gardener Summary in Kannada