2nd PUC Basic Maths Question Bank Chapter 17 Limit and Continuity of a Function Ex 17.5

Students can Download Basic Maths Exercise 17.5 Questions and Answers, Notes Pdf, 2nd PUC Basic Maths Question Bank with Answers helps you to revise the complete Karnataka State Board Syllabus and score more marks in your examinations.

Karnataka 2nd PUC Basic Maths Question Bank Chapter 17 Limit and Continuity of a Function Ex 17.5

Part – A

2nd PUC Basic Maths Limit and Continuity of a Function Ex 17.5 Two or Three Marks Questions and Answers

Question 1.
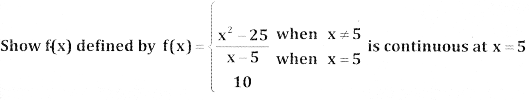
2nd PUC Basic Maths Question Bank Chapter 17 Limit and Continuity of a Function Ex 17.5 - 1
Answer:
Also f(x) at x = 5 is 10; i.e, f(5) = 10

∴ the function f(x) is continuous at x = 5

Question 2.
2nd PUC Basic Maths Question Bank Chapter 17 Limit and Continuity of a Function Ex 17.5 - 3
Answer:

Question 3.
Define f(0) so that f(x) = \(\frac{x}{1-\sqrt{1-x}}\) become continuous at x = 0
Answer:
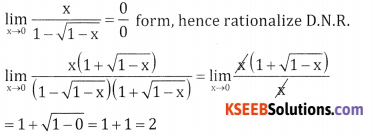
2nd PUC Basic Maths Question Bank Chapter 17 Limit and Continuity of a Function Ex 17.5 - 5
Also f(x) at x = 0 is 2
i.e f(x) = f(0) = 2
since f is continuous at x = 0.

Question 4.
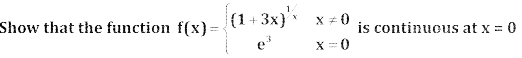
2nd PUC Basic Maths Question Bank Chapter 17 Limit and Continuity of a Function Ex 17.5 - 6
Answer:

Also f(x) at x = 0 is e³ i.e f(0) = e³

∴ f(x) is continuous at x = 0

Question 5.
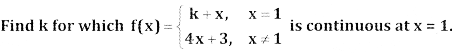
2nd PUC Basic Maths Question Bank Chapter 17 Limit and Continuity of a Function Ex 17.5 - 9
Answer:

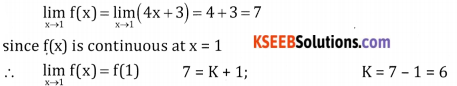
Question 6.
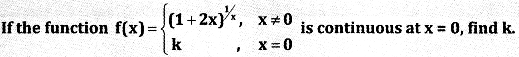
2nd PUC Basic Maths Question Bank Chapter 17 Limit and Continuity of a Function Ex 17.5 - 11
Answer:

Question 7.
2nd PUC Basic Maths Question Bank Chapter 17 Limit and Continuity of a Function Ex 17.5 - 13
Answer:

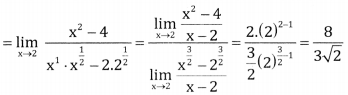
Question 8.
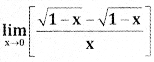
2nd PUC Basic Maths Question Bank Chapter 17 Limit and Continuity of a Function Ex 17.5 - 15
Answer:

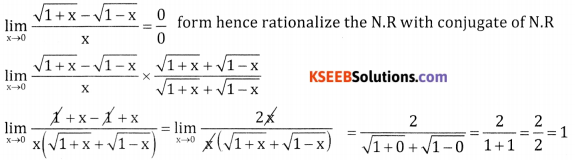
Question 9.
2nd PUC Basic Maths Question Bank Chapter 17 Limit and Continuity of a Function Ex 17.5 - 17
Answer:

Question 10.
2nd PUC Basic Maths Question Bank Chapter 17 Limit and Continuity of a Function Ex 17.5 - 19
Answer:

Part – C

2nd PUC Basic Maths Limit and Continuity of a Function Ex 17.5 Five Marks Questions and Answers

Question 1.
2nd PUC Basic Maths Question Bank Chapter 17 Limit and Continuity of a Function Ex 17.5 - 21
Answer:

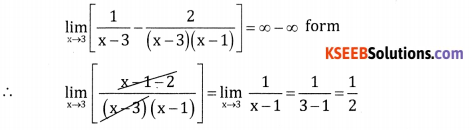
Question 2.
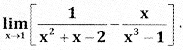
2nd PUC Basic Maths Question Bank Chapter 17 Limit and Continuity of a Function Ex 17.5 - 23
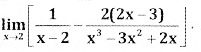
Answer:

Question 3.
2nd PUC Basic Maths Question Bank Chapter 17 Limit and Continuity of a Function Ex 17.5 - 25
Answer:

Question 4.
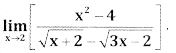
2nd PUC Basic Maths Question Bank Chapter 17 Limit and Continuity of a Function Ex 17.5 - 27
Answer:

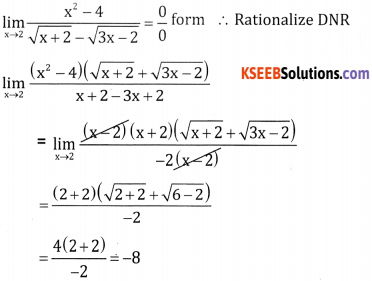
Question 5.
2nd PUC Basic Maths Question Bank Chapter 17 Limit and Continuity of a Function Ex 17.5 - 29
Answer:

Question 6.
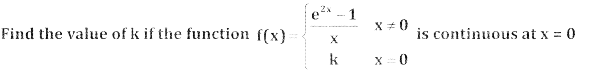
2nd PUC Basic Maths Question Bank Chapter 17 Limit and Continuity of a Function Ex 17.5 - 31
Answer:

part – B

2nd PUC Basic Maths Limit and Continuity of a Function Ex 17.5 Six Marks Questions and Answers

Limits Theorems

Question 1.
prove that \(\lim _{x \rightarrow a} \frac{x^{n}-a^{n}}{x-a}=n a^{n-1}\) for all values of n
Answer:
Case – 1 : Let n be a positive integer.
xⁿ – aⁿ = (x – a)(x^{n -1} + x^n-2 . a + x^n-3 . a² + ……. + a^n-1
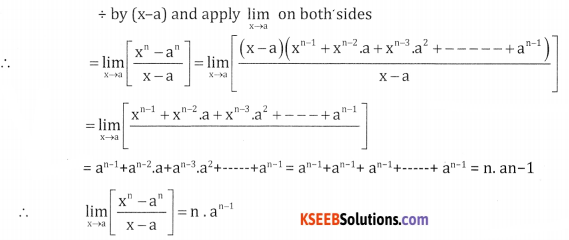
2nd PUC Basic Maths Question Bank Chapter 17 Limit and Continuity of a Function Ex 17.5 - 34

Case – 2: Let n be a positive integer
Put n = -m, m > 0
2nd PUC Basic Maths Question Bank Chapter 17 Limit and Continuity of a Function Ex 17.5 - 35

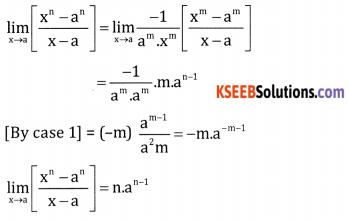
Case – 3: Let n = p/q where p and q are integers and q ≠ 0
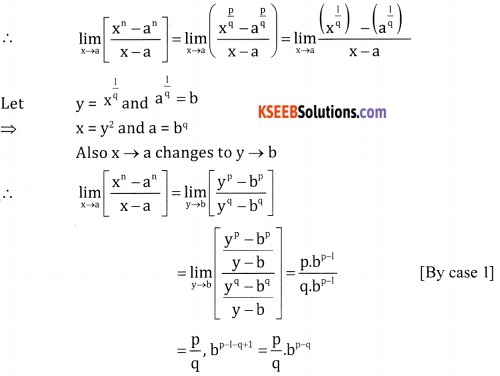
2nd PUC Basic Maths Question Bank Chapter 17 Limit and Continuity of a Function Ex 17.5 - 37

Question 2.

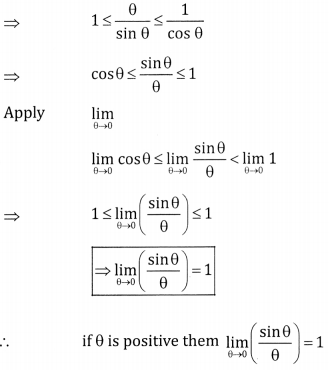
Answer:
Let ‘0’ the centre of unit circle, assume that θ is measured in positive radians

Fromn the figure, we have area of ∆AOB = area of sector AOB = area of ∆AOD
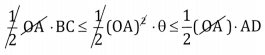
2nd PUC Basic Maths Question Bank Chapter 17 Limit and Continuity of a Function Ex 17.5 - 40
∴ Area of sector = \(\frac { 1 }{ 2 }\) (radius)² × angle in radians
From the triangle DOA, tan θ = \(\frac{\mathrm{DA}}{\mathrm{OA}}\) ∴DA = rtan θ
From the triangle BOC, sin θ = \(\frac{\mathrm{BC}}{\mathrm{OB}}\) ∴ BC = rsin θ
(1) becomes
⇒ BC ≤ θ ≤ DA
⇒ sin θ ≤ θ ≤ tan θ
Dividing by sin θ

2nd PUC Basic Maths Question Bank Chapter 17 Limit and Continuity of a Function Ex 17.5 - 41