1st PUC Basic Maths Previous Year Question Paper March 2020 (North)

Students can Download 1st PUC Basic Maths Previous Year Question Paper March 2020 (North), Karnataka 1st PUC Basic Maths Model Question Papers with Answers helps you to revise the complete syllabus.

Karnataka 1st PUC Basic Maths Previous Year Question Paper March 2020 (North)

Time: 3.15 Hours
Max. Marks: 100

Instructions:

1. The questions paper consists of five parts A, B, C, D, and E.
2. Part – A carries 10 marks, Part – B carries 20 marks, Part – C curries 30 marks, Part – D carries 30, and Part – E carries 10 marks.
3. Write the question numbers properly as indicated in the questions paper

PART-A

I. Answer any TEN questions. (10 × 1 = 10)

Question 1.
Write the imaginary part of 3-i.
Answer:
Imaginary part = – 1.

Question 2.
If A has 4 elements, how many elements will P(A) have?
Answer:
P(A) = 24 = 16 element

Question 3.
Simplify: a^x+y a^2x-y
Answer:

Question 4.
Find x if logx 25 = 2
Answer:
x² = 25
∴ x = ±5

Question 5.
Find the sum to infinity of GP 3,\(\frac{1}{1}, \frac{1}{3}\)…………..
Answer:
a = 3, r = \(\frac{1}{3}\)
∴ S_∞ = \(\frac{a}{1-r}=\frac{3}{1-\frac{1}{3}}=\frac{9}{2}\)

Question 6.
Solve for x : 7(x – 2) + 8(x – 3) = x + 10.
Answer:
7x – 14 + 8x – 24 = n + 10
∴ 14x = 48

Question 7.
Calculate SI for 2 years for ₹ 6000 at 8% pa.
Answer:

Question 8.
Find the present value of a perpetuity of 3,000 to be received forever at 14% pa.
Answer:
Perpetuity P_∞ = \(\frac{A}{r}=\frac{3000}{0.14}\) = ₹21,428

Question 9.
Convert 0.32 into a percentage.
Answer:
0.32 = 0.32 × 100 = 32%

Question 10.
Convert 45° into radians.
Answer:

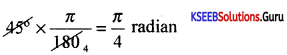
Question 11.
If A = 45° then show that sin 2 A = 2 sin A cos A.
Answer:
sin(2 × 45) = 2sin45°cos45°
sin90°= 2 × \(\frac{1}{\sqrt{2}} \times \frac{1}{\sqrt{2}}\)
1 = 1

Question 12.
Find the slope of the line 2x + 5y – 11 = 0.
Answer:
Slope = m = \(\frac{-2}{5}\)

Part-B

II. Answer any TEN questions. (10 × 2 = 20)

Question 13.
Find the number of positive divisors of 3⁴ × 5³ × 7²
Answer:
3⁴ × 5³ × 7² = p₁^α₁× p₂^α₂ × p₃^α₃
p₁ = 3, p₂ = 5, p₃ = 7, α₁ = 4, α₂ = 3, α₃ = 2
T(n) = (1 + α₁)(1 + α₂)(1 + α₃) = (1 +4)(1 + 3)(1 + 2) = (5)(4)(3) = 60

Question 14.
Three bells call at intervals 30 sec, 40 sec, 50 sec respectively. They start together. After how many minutes will the next bell call together.
Answer:

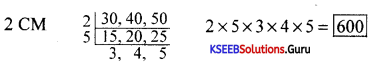
Hence the bills will fall together after 600 second or \(\frac{600}{60}\) = 10 minutes

Question 15.
If (x + y, x – y) = (5, 1) find x and y.
Answer:

Question 16.
Prove that (xb-c)a.(xc-a)b.(xa-b)c = 1
Answer:

Question 17.
Which term ofan AP \(\frac{1}{2}\), 1, \(\frac{1}{2}\) is 5?
Answer:
a = \(\frac{1}{2}\), d = 1 – \(\frac{1}{2}\)= \(\frac{1}{2}\), an = 5, n =?
a_n = a + (n – 1)d
5 = \(\frac{1}{2}\) + (n – 1)\(\frac{1}{2}\)

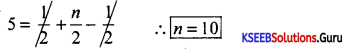
Question 18.
Solve x + 2y = 1
3x – 2y = 5
Answer:

x = 3/2
x + 2y = 1
\(\frac{1}{2}\) + 2y = 1
2y = 1 – \(\frac{3}{2}\)
2y = –\(\frac{1}{2}\)
∴ y = – \(\frac{1}{4}\)

Question 19.
If a and b are the roots of the equation 3x² – 6x +4 = 0. Find α² + β².
Answer:
α + β = \(-\frac{b}{a}=\frac{-3}{-6}=\frac{1}{2}\)
∴ α² + β² = (α + β)² – αβ
αβ = \(\frac{c}{a}=\frac{4}{5}\)
= \(\left(\frac{1}{2}\right)^{2}-\frac{4}{3}=\frac{1}{4}-\frac{4}{3}-\frac{3-16}{12}=\frac{-13}{12}\)

Question 20.
Solve linear inequalities and represent on the number line
7x + 3 < 5x + 9, X∈ R
Answer:

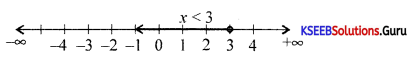
7x + 3 < 5x + 9
7x – 5x < 9 – 3
2x < 6
∴ x < 3

Question 21.
Find the future value of an annuity of 600 at 4% pa payable for 2 years,
Answer:
F=? u= 600, r = 0.04, n = 2ys
F = \(\frac{a\left[(1+r)^{n}-1\right]}{r}=\frac{600\left[(1+0.04)^{2}-1\right]}{0.04}=\frac{600\left[(1.04)^{2}-1\right]}{0.04}\) = ₹ 1224

Question 22.
Prove that (i + tan² θ)(1 – sin² θ ) = 1
Answer:
LHS= (1 + tan²θ)(1 – sin²θ)

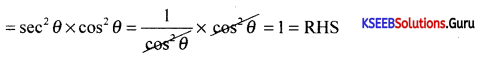
Question 23.
Find the value of cos 60° – sin 30° – cots 450
Answer:

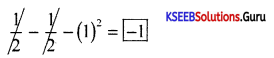
Question 24.
One end of a diameter of a circle is (1, 3) and its center is (4, -2). Find the coordinates of the other end of this diameter.
Answer:
By midsection formula:
(4, -2) = \(\left[\frac{x_{1}+1}{2}, \frac{y_{1}+3}{2}\right]\)
∴ \(\frac{x+1}{2}\) = 4
∴ x = 7
\(\frac{y_{1}+3}{2}\) = -2
∴ y = -7
∴ the other end of diameter = (x, y) = (7, -7)

Question 25.
Find the equation of the locus of all points equidistant from the point (2,4) and y-axis.
Answer:
Locus = P(x,y).A(2,4) and y-sinB (0, y)
Given PA = PB
\(\sqrt{(x-2)^{2}+(y-4)^{2}}=\sqrt{(x-0)^{2}+(y-y)^{2}}\)

y2 – 4x – 8y + 20 = 0 equation of the locus

Part-C

III. Answer any TEN questions. (10 × 3 = 30)

Question 26.
Prove that 3 + \(\sqrt{5}\) is an irrational number.
Answer:
If possible let 3 + \(\sqrt{5}\)be a rational number
∴ 3 + \(\sqrt{5}\) = \(\frac{p}{q}\) where p, q ∈ Z, q ≠ 0
∴ \(\sqrt{5}\) = \(\frac{p}{q}\) – 3 = rational – rational = rational number
∴ \(\sqrt{5}\) is a rational number which is a contradiction.
∴ our assumption is wrong
∴ 3 + \(\sqrt{5}\) is irrational.

Question 27.
If f(x) = 2x + 1 g(x) = x² + 2x + 1.
Find (i) fog (2)
(ii) gof (3)
(iii) fog (1)
Answer:
(i) fog(1) = f[g(1)] = f[13+1] = f(2) = 2
(ii) fog(2) = f[g(2)] = f[23+1] = f(9) = 9
(iii) gof(1) = g[f(1)] = g[1] = 13 + 1 = 2
(iv) gof(-1) = g[f(-1)] = g[-1] = (-1)3 + 1 = -1 + 10

Question 28.
If a^x = b^y = c^x and b² = ac show that \(\frac{1}{x} \times \frac{1}{z}=\frac{2}{y}\).
Answer:
Let a = b = c = k (say)
∴ a^x = k ⇒ a = k^1/x, b^y = k ⇒ b = k^1/y, c^z = k⇒ c^z = k^1/z
Now, b² = ac
∴ (k^1/y)² = k^1/x.k^1/z
∴ k^2/x = k^1/x+1/z

Bases are same ∴ Equating powers on both the sides, we get.
\(\frac{2}{y}=\frac{1}{x}+\frac{1}{z}\)

Question 29.
If x² + y² = 12xy show that 2log(x-y) = log2 + log5 + logx + logy.
Answer:
LHS = 2 log (x -y)
= log(x – y)² = log(x² + y² – 2xy) Put x² + y² = 12xy
= log(12xy – 2xy) = log(10xy) = log(2 × 5 × x × y)
= log2 + log5 + logx + logy = RHS

Question 30.
The 3 HM’s between \(\frac{1}{4}\) and \(\frac{1}{12}\).
Answer:
Insert 3 H.M.’s between and \(\frac{1}{4}\) and \(\frac{1}{12}\)
\(\frac{1}{4}\), h1, h2, \(\frac{1}{12}\)………. HP
4, \(\frac{1}{\mathrm{~h}_{1}}, \frac{1}{\mathrm{~h}_{2}}, \frac{1}{\mathrm{~h}_{3}}\), 12……… AP
a = 4 d =? T = 12 n = 5
Tn = a + (n – 1)d
12 = 4 + (5 – 1)d
12 – 4 = 4d
4d = 8d = 2
4,6,8,10,12,……… AP
\(\frac{1}{4}, \frac{1}{6}, \frac{1}{8}, \frac{1}{10}, \frac{1}{12}\) …………..HP .
∴ Harmonic means are \(\frac{1}{6}, \frac{1}{8}, \frac{1}{10}\)

Question 31.
Five years ago, the father’s age was 5 times as old as his son and after 3 years he will be 3 times as old as his son. Find their present ages.
Answer:
Let the present age of the son = x ys
5 yrs ago son’s age = (x – 5) yr
Father’s age = y yrs
5 year ago father age = (y – 5)
Given (y – 5) = 5(x – 5)
y – 5 = 5x – 25
5x – y – 20 = 0 …(1)
After 3 year Son’s age = (x + 3)
Father’s age = (y + 3)
∴ (y + 3) = 3(x + 3)
y + 3 = 3x + 9
∴ 3x- y + 6 = 0 …(2)

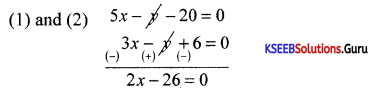
∴ x = 13yrs
y = 45 yrs

Question 32.
Solve the following graphically x + y < 5.
Answer:
x + y < 5

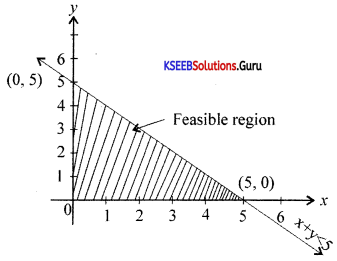
Question 33.
If ₹ 500 amounts to ₹ 725 at 9% simple interest in sometimes what will ₹ 600 amount to at 11% in the same time.
Answer:
Given P = ₹ 500, A = ₹ 725
SI = 225, R = 9%, T = ?
SI = \(\frac{\mathrm{PTR}}{100}\) = 225 = \(\frac{500 \times 7 \times 9}{100}\)
∴ T = ₹ 5yrs
Again is P = 600, R = 11% T = 5yrs I = ?
I = \(\frac{600 \times 5 \times 4}{100}\) = ₹330
∴ A = P + I = (600 + 300) = 930

Question 34.
5 kg of Sugar at the rate of ₹ 15/kg
8 kg of Wheat at a rate of ₹ 22/kg
7 kg of Rice at a rate of ₹ 351kg and
4 kg of oil at a rate of ₹ 85/kg. What is the average price/kg of all the commodities?
Answer:
Average price /kg (x̄w) = \(\frac{n_{1} w_{1}+n_{2} w_{2}+n_{3} w_{3}+n_{4} w_{4}}{n_{1}+n_{2}+n_{3}+n_{4}}\)
n = Quality, w = weight
= \(\frac{(5 \times 15)+(8 \times 22)+(7 \times 35)+(4 \times 85)}{(5+8+7+4)}\)
x̄w = \(\frac{836}{24}\) = ₹ 34.83

Question 35.
By how many percent should the use of tea be increased if the price of tea is decreased by 10% so that expenditure remains unchanged.
Answer:
Let the price of tea = ₹ 100
Quantity = 100 unit
∴ Total expenditure = (100 × 100) = 10,000
New price = 100 – 10 = 90 Net quantity = y
∴ Total expenditure = 90 y
∴ 90y= 10,000
∴ y = \(\frac{10000}{90}\) = 111.11
∴ % Increase = \(\frac{\text { Increase }}{\mathrm{CP}}\) × 100 = \(\frac{11.11}{100}\) × 100 = 11.11%

Question 36.
Find x : x sin45° cos260° = \(\frac{\tan ^{2} 60^{\circ}{cosec} 30^{\circ}}{\sec 45^{\circ} \cdot \cot ^{2} 30^{\circ}}\)
Answer:

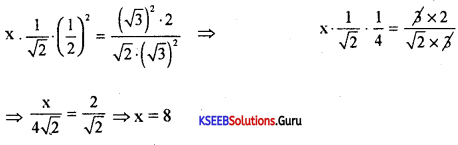
Question 37.
Three comers of a parallelogram ABCD taken in order are A(-1, -6) B(2, 5) C(7, 2). Find the fourth vertex.
Answer:
Mid point of the diagonal AC = midpt. of diagonal BD
Formal D (x, y) \(\left[\frac{-1+7}{2}, \frac{-6+2}{2}\right]=\left[\frac{x+2}{2}, \frac{y-5}{2}\right]\)

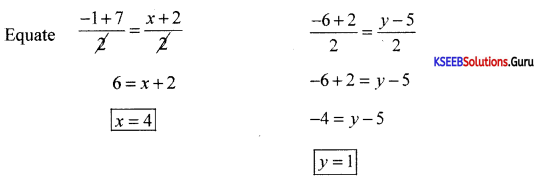
4th vertex D = (x, y) = (4,1)

Question 38.
Find the equation of a line that passes through the point (-4 5) and whose intercepts are equal in magnitude but opposite in sign.
Answer:
Let a = a, b = – a ∴ \(\frac{x}{a}+\frac{y}{b}\) = 1 (Intercept form)
at(-4, 5), \(\frac{-4}{a}+\frac{5}{a}\) = 1
∴ a = 1, b = -1

Equation of the line is \(\frac{x}{1}+\frac{y}{1}\) = 1
∴ x – y – 1 = 0

Part-D

IV. Answer any SIX questions. (6 × 5 = 30)

Question 39.
In a certain college with 500 students, 300 take the milk and 250 take tea. Find how many take (a) Milk only (b) tea only (c) both milk and tea. Also, represent this by Venn diagram.
Answer:
n(M∪T) = 500
n(M) = 300 n(T) = 250
n(M∪T)= n(M) + n(T) – n(M∩T)
n(M∪T) = n(M)+n(T) – n(M∪T) = 300 + 250 – 500
n (M ∩ T) = 50
n(M – T)=n(M) – n(M∩T) = 300 – 50= 250
n(T – M)= n(T) – n(M ∩ T) = 250 – 50 = 200

Question 40.
Solve using log table : \(\frac{5.6348 \times 25.645}{12.75 \times 19.78}\)
Answer:
Let x = \(\frac{5.6348 \times 25.645}{12.75 \times 19.78}\)
apply log on both sides
log x = log\(\left[\frac{5.6348 \times 25.845}{120+2 \times 1 \times 19.7}\right]\)
logx = log 5.6348 + log 25. 645 – log (12.72)- log (19.78)
= (0.7508) + (1.4090) -(1.1044) – (1.2962)
log x = 0.2408
x = 1.4237

Question 41.
The first and last term of the GP is 3 and 96 respectively sum to n terms is 189. Find the common ratio and the number of terms.
Answer:
Given a = 3, a_n = 96, S_n = 189, r = ?
a_n = a.r^n-1
96 = 3.(r)^n-1
∴ (r)^n-1 = 32 ………(1)
If S_n = \(\frac{a\left(l-r^{n}\right)}{(l-r)}\)
189 = \(\frac{3\left(1-r^{n}\right)}{1-r}=\frac{3\left(1-r \cdot r^{n-1}\right)}{1-r}\)
189 = \(\frac{3(1-r(32))}{1-r}\)

1 – 32r = 63 – 63r
63r – 32r = 63 – 1
31r = 62
∴ r = 62/31 = 2
∴ r^n-1 = 32 = 2^n-1 = 32
2^n-1 = 25
∴ n – 1 = 5
∴ n = 6

Question 42.
Find the quotient and remainder obtained x³ + 4x² – 7x – 10 by (x + 1) dividing and solve.
Answer:
Given x + 1 = 0

Remeinder = 0, Quotient ∴ x² – 3x -10

Question 43.
Find the difference between simple interest and compound interest on ₹ 18,000 invested for 4 years at 8% pa. where Cl is compounded annually.
Answer:
Given P = ₹ 18,000, T = 4yr, R = 8%
SI – \(\frac{\mathrm{PTR}}{100}=\frac{18,000 \times 4 \times 8}{100}\) = ₹5,760
CI = A = P[(1 + i)]ⁿ
= 18,000[1 + 0.08]⁴
A = 24,488.8
∴ CI = 24.488.8 – 18,000 = 6488.8
∴ Difference between Cl and SI = 6488.8 – 5760 = ₹728.8

Question 44.
Mrs. Geetha deposits 4,00,000 on retirement in a bank that pays 10% p.a. interest. How much can be drawn annually for a period of 10 years?
Answer:
P = 4,00,000, i = 0.10, n = 10 ys, a = ?
P = \(\frac{a\left[(1+i)^{n}-1\right]}{i(1+i)^{n}}\)
4,00,000 = \(\frac{a\left[(1+0.10)^{10}-1\right]}{0.1(1+0.1) 10}\)
4,00,000 = \(\frac{a[2.5937-1]}{0.1 \times 2.5937}\)
∴ a = \(\frac{4,00,000}{6.1445}\)
a = ₹65,098.8

Question 45.
A shopkeeper sold a watch at a 5% loss. Had he purchased it at 10% p.a. sold it for 140 more his gain would have been one-fourth of the original cost price. Find the cost price of the watch.
Answer:
Let x be the original C.P of the watch
then 5% of x = \(\frac{95 x}{100}\) = SP
given caritian
(x – 10 %g.x) + \(\frac{x}{4}=\frac{95 x}{100}\) + 140
\(\frac{90 x}{100}=\frac{x}{4}=\frac{95 x+14000}{100}\)

90x + 25x = 95x + 14000
20x = 14000
∴ x = \(\frac{14000}{20}\)
x = ₹700

Question 46.
If then prove that x = arsin AcosB, y = brsin AsinBr and z = crossA then prove that \(\) = r2
Answer:
\(\frac{\mathrm{x}}{\mathrm{a}}\) = rsinAcosB \(\frac{\mathrm{y}}{\mathrm{b}}\) = rsinAstnB
\(\frac{\mathrm{z}}{\mathrm{c}}\) = rcos A
\(\frac{x^{2}}{a^{2}}+\frac{y^{2}}{b^{2}}+\frac{z^{2}}{c^{2}}\) = r²2sin2Acos2 B + r² sin A sin2 B + r² cos2A
= r² sin2 A (cos² B + sin² B) + r² cos² A = r² sin² A + r² cos² A = r2 (sin² A + cos² A) = r² (1) = r2

Question 47.
Find the circumcenter of the triangle whose vertices are (-1,2) (3,0) (-2,4).
Answer:
Assume the circum centre in p(x, y)
A (-1,2) B(3, 0), C(-2, 4)
PA = PB = PC
If PA = PB
∴ \(\sqrt{(x+1)^{2}+(y-2)^{2}}=\sqrt{(x-3)^{2}+(y-0)^{2}}\)

∴ 2x – 4y + 5 = -6x + 3
∴ 8x – 4y – 4 = 0 + 4
∴ 2x – y – 1 = 0 ………….(1)
Let PB = PC
\(\sqrt{(x-3)^{2}+(y-0)^{2}}=\sqrt{(x+2)^{2}+(y-4)^{2}}\)
∴ (x + 1)² + (y-2)² = (x-3)² + y²
-6x – 4x + 8 v + 9 – 20 = 0
-10x + 8y- 11 = 0 …(2)
Solve (1) and (2)
2x – y – 1=0
xy by 8

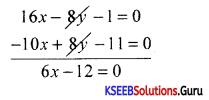
x = 2
y = 3
the circum centre = (2,3)

Question 48.
Find the foot of the perpendicular drawn from the point (-2, -1) on the line 3x + 2y – 5 = 0.
Answer:

Part-E

V. Answer any ONE question. (1 × 10 = 10)

Question 49.
(a) If A = {x : x² – 5x + 6 = 0; x ∈ N}
B = {x : x² – 7x + 12 = 0 x ∈ N}
Find (A – B) × B. 4
Answer:
A= {2, 3},B = {3,4}
∴ A – B ={2}
∴ (A – B) × B = {2} × {3 – 4} = {(2, 3), (2, 4)}

(b) Find the sum to V terms of a GP. 0.6 + 0.66 + 0.66 +
Answer:
Let S_n = 0.6 + 0.66 + 0.666 +………….n term
S_n = 6{0.1 + 0.11 + 0.111 +……………n term}
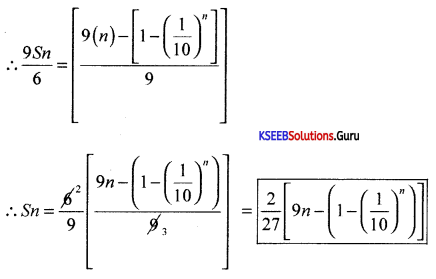
∴ \(\frac{S n}{6}\) = 0.1 +0.11 +0.11 +…………..+ n term
x^ly both side by 9
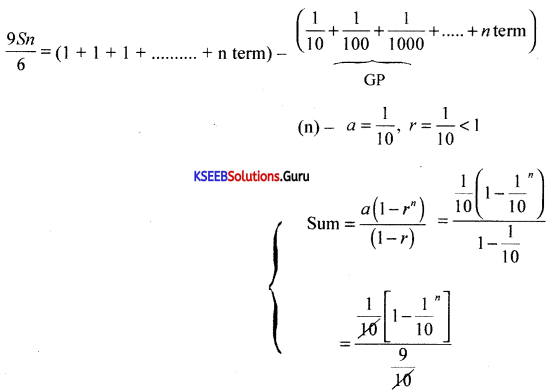
∴ \(\frac{9 S n}{6}\) = 0.9 + 0.99 + 0.999 +……………. + n term
∴ \(\frac{9 S n}{6}\) = (1-0.1) + (1 – 0.01) + (1 – 0.001) +………..+ n term
\(\frac{9 S n}{6}=\left(1-\frac{1}{10}\right)+\left(1-\frac{1}{100}\right)+\left(1-\frac{1}{1000}\right)\) + ……+ n term

(c) The average marks of 15 students of a class is 45. A student also has secured 50 marks leaves the class room. Find the average marks of the remaining 14 students.
Answer:
A × B = LCM × HCF
HCF 16, hem = 160, A = 64

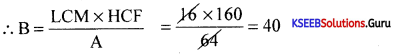
∴ another no. = 4

Question 50.
(a) If the cost function C(x) of producing ‘x’ unit of a product is given by C(x)= 500x² + 2500 x + 5000 and if each unit of the product is sold at 6000 then find BEP.
Answer:
Given C(x) = 500x² + 2500 x ± 5000 (x = output)
∴ Total Revenue = R(x) = price x quantity = 6000x
For B ∈ P: C(x) = R(x)
∴ 500x² + 2500x + 5000 = 6000x
∴ 500x² – 3500x + 50000
÷500 ∴ x² – 7x + 10 = 0
x = 5 or 2 units

(b) Find ’a’ so that the lines x – 6y + a = 0, 2x + 3y + 4 = 0 and x + 4y + 1 = 0 are concurrent.
Answer:
Solve 2x + 3y + 4 = 0 ….(1) and x + 4y + 1 = 0 xly by 2
2x + 8y + 2 = 0 …(2)
(1) and (2)

2x + 3(2/5) + 4 = 0
2x = \(\frac{-6}{5}\) – 4 = \(\frac{-26}{5}\)
∴ x = -13/5
Sub in x – 6y + a = 0
a = 6y – x
∴ a = 6(2/5) – (-13/5)
a = \(\frac{12+13}{5}\) = 5

(c) The average marks of 15 students of a class is 45. A student also has secured 50 marks leaves the class room. Find the average marks of the remaining 14 students.
Answer:
Total marks and A. 15 students = 45 × 15 = 675

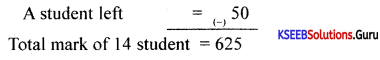
∴ Average marks of 14 student = \(\frac{625}{14}\) = 44.64